What REALLY is e? (Euler’s Number)
Вставка
- Опубліковано 21 лис 2024
- To try everything Brilliant has to offer-free-for a full 30 days, visit brilliant.org/.... You’ll also get 20% off an annual premium subscription.
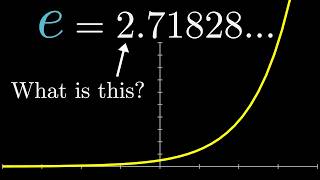
In this video, we explain where Euler's number e = 2.71828... comes from. We start by studying the example of compound interest, and use it to generalize e to being a constant that describes continuous self-referential (exponential) growth.
Enjoy!
Subscribe for more: www.youtube.co...
/ foolish.chemist
/ foolishchemist
Feel free to reach out to me at:
zenon.chemistry@gmail.com
#maths #calculus #multivariable #multivariablecalculus #perspective #some #someπ #learn #learning #intuition #intuitive
FTC Disclaimer: This video was sponsored by Brilliant








To try everything Brilliant has to offer-free-for a full 30 days, visit brilliant.org/FoolishChemist. You’ll also get 20% off an annual premium subscription.
Brilliant
e is the sum of all original angles. In one sentence. What is it really? Really, it's 0.11111111111111...
"It's the constant of self-similar change" is my best pre-video attempt at distilling e in one sentence.
had this exact thought as well
I worked for a bank where savings account interest was compounded continuously. I used both Euler’s Number e and natural log ln quite frequently. Your explanation made sense to me. Next time you are talking to a banker ask for a proof of how your savings interest (Annual Percentage Yield) was determined. I bet no one in the bank can do the calculation let alone explain it.
I remember once a financial journalist, might have been the BBC don't recall, took the terms of interest to a maths profession at a top university, might have been Oxford, and asked him (or her) to evaluate it. It took him four hours. Could have been three or five.
I'm guessing he was just presented with insufficient information but still, it obviously wasn't straightforward. A mere mortal wouldn't stand a chance.
Also, I accept that my hazy recollection, plus this being a UA-cam comment, is pretty much the definition of apocryphal.
I remember once a financial journalist, might have been the BBC don't recall, took the terms of interest to a maths profession at a top university, might have been Oxford, and asked him (or her) to evaluate it. It took him four hours. Could have been three or five.
I'm guessing he was just presented with insufficient information but still, it obviously wasn't straightforward. A mere mortal wouldn't stand a chance.
Also, I accept that my hazy recollection, plus this being a UA-cam comment, is pretty much the definition of apocryphal.
These people are barely educated. There’s no point showing off to them.
Nice! This explains why the exponential map appears even when you’re not working with numbers anymore
The reason we choose 100%(100% = 100/100 = 1) is because 1 is the multiplicative identity over the real numbers(and all of the numbers you will ever use)
doodoo kaka
@Yunahsky wtb modular arithmetic, for example 6 is a multiplicative identity mod 5
@paridhaxholli 6 = 1 mod 5.
The most popular definitions for e:
The one in this video: e = lim(n to inf) of (1 + 1/n)^n
The infinite sum of 1/n! starting at 0 (n! = 1*2*3*4*.....*n)
e is base for the natural logarithm
e is the base for the exponential function exp(x) where the derivative of that function is also exp(x) so f(x) = f'(x)
or the solution to the ivp y'=y, y(0)=1
you can also change that limit to the form e=lim u->1 of u^(1/u-1), which is a lot easier to compute
Thank you for making this
Thank you for making it so clear and simple. Appreciated.
Thanks for making this video sir, really helpful
Up until your discovery of constant-variable lambda I felt I was with you. From that point on I would need much more graduation. Very unfortunately I lost you from that point on.
David Lixenberg
Great video explaining e. I never thought e can be describe as "the self referential growth or decay" of itself.
Nice!!!
Nicely explained though It was difficult to understand why you were applying the lambda exponentials.
Great video! What tablet device do you use to write on in videos?
iPad!
awsome explanation
to help understanding concepts
It was a useful explanation that added to my knowledge, thank you for such educational videos, it helped to improve human knowledge and science.
What iPad app are you using?
The Goat is back🎉
This is exactly how my tutor explained e
i want to know the app you were writing in, if it is an app
Here is e in one sentence:
The only value where the derivative of a^x = a^x
a=0
@@fullfungo except 0
@@fullfungo You need for a^x to be defined for all x, and since 0^0 is undefined (I know taking the limit implies it should be 1, but that still requires a limit) you can immediately rule out 0 as a possible value for a.
You seem to have accidentally given one of the great argument for why τ (the ratio of a circle's circumference to its radius) is more practical than π (τ/2), right at 18:31 !
Ahhh, a good point! I didn’t even think about this lol
Hot Tip: (1.0000000001)^⁹ = e
This is the smallest number taken to the exponent of its decimal place which is e, or 2.718.... Just add more zeros between the ones and take it to a higher exponent to get "e" with more decimal places.
What would be e if a bacteria grew three times itself.
@achyutkarve it is a three point curve using e as the moderator.
Thank you !!!!
Ok, I'll need to watch that a few more times and with a pen and paper.
Tell me, why does e come on when making a complex circle?
Eulers formula is one of the least intuitive imo…I can’t think of a good way to describe it. The “why” of your question can be answered most easily probably using Taylor series-if you look up the Taylor expansions for e^x, cos x, and sin x, you can see why e^ix = cos x + isin x. Though that’s just a mathematical derivation, not an intuitive explanation
I'm sorry, but to ignore calculus in a video trying to give an intuition for e is a somewhat baffling move, since what makes e significant is its use in calculus (specifically for taking the derivative or anti-derivative of an exponential).
Walking through how solving f'(x) = f(x) naturally leads to an exponential, and further showing that the base of that exponential is an exact constant, also gives an intuition on why it matters in chemistry and physics, after perhaps explaining why exponential equations are used to model how certain kinds of systems evolve (and how the past of them can be predicted) based only on some constants of the system and the current state.
π has a solution,a circle Alfa and Omega
E is a letter that represents a number of
Time dialation,only correct at that moment time is a continuum
21:38 you bet!
Time Dialation,continuum only correct at that exact moment,,benchmark that point x,2x+5 =8')
Continuum has time dialation,only true at that exact moment new computing has up charge
Doode ur the goat
So Euler's number is the same as natural log. 2.718281828459 ?
A natural logarithm is a logarithm with base e, so ln e = 1.
I don't think there is any reason at all to bring the approximation into it. The other stuff is great. But I think I can do better. I'm going to try and slap something together.
scalability of large numbers by a factor, decimal converter? Used highly in diff eq, the visual helps, thanks, now how to upscale control theory into life?
Trancedental number like pi and square root of not perfect square
I tried to educate 3m to multiply the money in my bank account but they did not have the necessary algebra skills to get the right answer.
The easiest way to describe pi: It’s half tau.
Good video on euler's number
I oddly couldn't take the guy seriously because he's holding a clip-on mic in his hand for some reason
E
E
X,2x+5=8')
😞 Someday, I'd be smart enough to understand this.
If you don't understand, then it's not taught right for you.
1:06 nor is pi. Pi is irrational and cant be expressed as a ratio of two numbers.
Of two integers, no…but there’s no guarantee that the circumference and diameter of a circle will both be integers (therefore it is still a ratio of two numbers). In fact, pi being irrational tells us that that’s impossible!
@FoolishChemist so it can be expressed as a ratio of two irrational numbers?
@@michaelcolbourn6719 Doesn't even have to be two irrational numbers. In fact most of the time it's one irrational and one rational. It just can't be two integers!
Pi is the ratio of
Circumference:diameter
We aint talking about numbers here.
*Everytime*
Tipical american: all about money.
Fail.
The fact, that the language of the Math is not comprehensive to me, makes me sad.
Yet another rehashed bad way to teach e. Not knocking Foolish Chemist. It’s a good math video. We tend to teach math by backing into concepts from an historical perspective. Instead we should relegate the arduous path to discovery of things like e and i to a math history class. Now that we have a better understanding of these concepts we should start with a modern perspective.
Too much complicated
wtf is h
Plancks constant!
e = 1/0! + 1/1! + 1/2! .............
The way you hold the pen is just awful. It doesn't let to see what you are writing