A Special Cubic Equation
Вставка
- Опубліковано 7 лют 2025
- 🤩 Hello everyone, I'm very excited to bring you a new channel (aplusbi)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
/ @aplusbi
/ @sybermathshorts .
x^2-x^3=80
0^0 = 1 😄 • What is 0⁰ (0 To The P...
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @UA-cam @Apple @Desmos @NotabilityApp @googledocs @canva
Can You Simplify A Radical? • Can You Simplify A Rad...
Can You Simplify Another Radical? • Can You Simplify Anoth...
Summing An Interesting Infinite Series: • Summing An Interesting...
Solving a+2ab+b=8 | A Diophantine Equation: • Solving a+2ab+b=8 | A ...
Summing An Interesting Infinite Series: • Summing An Interesting...
Ramanujan's Famous Infinite Radical: • Ramanujan's Famous Inf...
Comparing Two Very Irrational Numbers: • Comparing Two Very Irr...
❤️ ❤️ ❤️ My Amazon Store: www.amazon.com...
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
CHECK IT OUT!!! ❤️ ❤️ ❤️
INFINITE SERIES:
❤️ • An Infinite Sum of Rec...
❤️ • Summing A Series In Tw...
❤️ • An Infinite Sum With R...
❤️ • An Interesting Sum Wit...
❤️ • Summing The Reciprocal...
⭐ Join this channel to get access to perks:→ bit.ly/3cBgfR1
My merch → teespring.com/...
Follow me → / sybermath
Subscribe → www.youtube.co...
❤️ Let's Denest A Difficult Radical | Viewer Suggested: • Let's Denest A Difficu...
⭐ Suggest → forms.gle/A5bG...
If you need to post a picture of your solution or idea:
in...
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries
PLAYLISTS 🎵 :
Number Theory Problems: • Number Theory Problems
Challenging Math Problems: • Challenging Math Problems
Trigonometry Problems: • Trigonometry Problems
Diophantine Equations and Systems: • Diophantine Equations ...
Calculus: • Calculus
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @UA-cam @Apple @Desmos @NotabilityApp @googledocs @canva
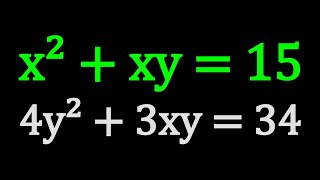








whoops you put (y+½)² at 1:59
About the 1st path: you cannot substitute x as y+1/3 and y+1/2 at the same time in the same equation.
0= x^2 - x^3 - 80 = x^2 - 16 - (x^3 + 64) = (x+4)(x-4) - (x+4)(x^2 -4x + 16) -->
(x+4)(x^2 - 5x + 20) = 0 ...
x^3-x^2+/-x+80=0 , (x+4)(x^2-5x+20)=0 , x= -4 , x=(5+/-V(25-80))/2 , x=(5+/-i*V(55))/2 ,
1 4
-5 -20
20 80
Cubic with trivial root, can be solved in memory
Χ=-4,χ=(5+-55^(1/2)*i)/2.
Other Method. x^3-x^2+80=0. Obvius root x=(-4) since (-4)^3-(-4)^2+80=-64-16+80=0. We apply Horner's Short Division x=-4
1 -1 0 80 || -4
-4 +20 -80
1 -5 20 0
So (x-(-4))•(x^2-5x+20)=0 so x+4=0 or x=(+5+-√-55)/2 so x1=-4 or x2,3=(5+-i•√55)/2.
x = -4
x^3-x^2=-80
(x+4)(x^2-5x+20)=0
x = {5+-(25-80)^(1/2)}/2 = [5+-{5^(1/2)*11^(1/2)}i]*2^(-1)
Got em all using RRT and long division.
x=-4,x=(5+i√55)/2,x=(5-i√55)/2
By a special Syberguy.
x^3-x^2+80=0 so x^3+64-x^2+16=0 so (x^3+4^3)-(x^2-4^2)=0 so (x+4)(x^2-4x+16)-(x+4)(x-4)=0 so (x+4)(x^2-4x+16-x+4)=0 so (x+4)(x^2-5x+20)=0 so x+4=0 or x^2-5x+4=0 so x1=-4 or x2,3=(+5+-√25-80)/2=(5+-i•√55)/2.
The third summand is 20(x+4) not 4(x+4), in your second method.
Exactly
-4 ?
Yes.
La met.1 x^2=(y+1/3) nu (y+ 1/2) in rest totul ok
you are mistaken