Calculus 3: Tensors (4 of 28) The Dyad: 3 Vectors Define "Stress" at the 3 Planes

Visit ilectureonline.com for more math and science lectures!
In this video I will explain a dyad, a tensor of rank 2, by drawing 3 vectors, each on the surface of a cube representing a piece of a beam.
Next video in the series can be seen at:
• Calculus 3: Tensors (5...

[@barryhughes9764]

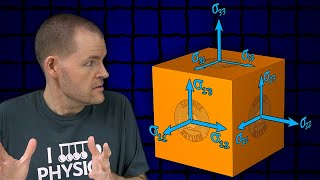
At last , someone who is explaining the 'Tensor concept ' in a form I can comprehend. Thank you sir.

[@philwatson698]

At last a real Explanation of tensors instead of the usual incomprehensible definition-and-proof torture

[@samyuen8234]

there's a minor mistake which is the component of T on
x-plane, in z basis vector. it shld be Txz = a13 not Txy again.

[@MichelvanBiezen]

You are correct. Thank you.

[@201120555]

Thank you so much Dr ,you are explained in a clear side..

[@fizixx]

Beautifully explained! 🥳

[@MichelvanBiezen]

Thank you. 🙂

[@fizixx]

@@MichelvanBiezen 💪

[@amicablefire9693]

This is awesome, thank you for the clear explanation

[@MichelvanBiezen]

You are welcome. Glad you liked it. 🙂

[@maryamgholinasab4531]

wow sir thank you, very well explained

[@MichelvanBiezen]

Thanks and welcome

[@davidjones5319]

Excellent!!

[@HD-wp7fu]

Thank you so much! it is so so so helpful

[@MichelvanBiezen]

Glad it was helpful! Thank you for the feeback. 🙂

[@sgiri2012]

Sir, cube have 6 Faces. Why don't we consider stresses in all faces instead of just three of them and represented as tensor? This stresses may cause the cube to elongate, compress and made them to shear. How do we account for this ? Sir.

[@MichelvanBiezen]

If you watch the rest of the videos, some of your questions will be answered.

[@gurumoorthy8247]

Should we use a plane always while using tensor

[@BONGPHYSICS]

Thank you sir

[@donaldbagwell6309]

in the top row, there is xx,xy xy, shouldn't it be xx, xy and xz

[@yizhang7027]

The title says it all.

[@nehaj4490]

I am grateful for this video series.
I also have another request, can you please make video on Bessel, laguerre and legendre functions.

[@MichelvanBiezen]

Those topics are on the list of things to cover, but it will be a while since we are working on many other topics at this time.

[@nehaj4490]

@Michel van Biezen My exam is on 17th of june so it would be appreciated if you upload beforehand.
I am addicted to your channel you teach in such a convenient way that I am going through all your videos that are related to my syllabus. Appreciate your hard work👍.
Really, it has helped many of us. Respect!

[@jerrymahone335]

I have a ghost idea in my head. Please explain this in terms of Ax = λ x. I think I see the eigenvalues and eigenvectors in the tensors. I just need for you to confirm it.

[@JenniferBrown-ry7qg]

thank you very much

[@learning-fq7mu]

👍👏👏

[@MichelvanBiezen]

Glad you like the video. 🙂

[@gurumoorthy8247]

Can we use tensor without a plane?

[@curtpiazza1688]

😊

[@MichelvanBiezen]

👍

[@vikasdwivedi3245]

very good bt camera shi nhi hi s

[@PATHMINDER]

The 1 st row should have:
xx xy xz