
- 27
- 325 897
Proof of Concept
United States
Приєднався 10 лип 2015
Mathematics for aspiring mathematicians. Created by Dr. Katherine Stange at the University of Colorado, Boulder. Learn about me under the "Community" tab, or here: math.katestange.net/
Rethinking the real line #SoME3
We take a geometric approach to rational numbers, to rethink how to organize the real line. Along the way, we visualize Diophantine approximation and continued fractions. And your favourite number, pi.
Much of the mathematics here is based on the following article:
Series, C. The geometry of markoff numbers. The Mathematical Intelligencer 7, 20-29 (1985). doi.org/10.1007/BF03025802
A big thanks to the Summer of Math Exposition competition for the motivation to make this happen, and a big thanks to my audience for forgiving my video-editing non-skills.
Some of the software used in creating this: Sage Mathematics Software, Manim, VPython, p5.js, Krita, Audacity, Kdenlive.
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Music used in the video:
Walk Through the Park -- TrackTribe
George Street Shuffle -- Kevin MacLeod
Quarter Mix -- Freedom Trail Studio
Love Struck -- E's Jammy Jams
George Street Shuffle by Kevin MacLeod is licensed under a Creative Commons Attribution 4.0 license. creativecommons.org/licenses/by/4.0/
Source: incompetech.com/music/royalty-free/index.html?isrc=USUAN1300035
Artist: incompetech.com/
Much of the mathematics here is based on the following article:
Series, C. The geometry of markoff numbers. The Mathematical Intelligencer 7, 20-29 (1985). doi.org/10.1007/BF03025802
A big thanks to the Summer of Math Exposition competition for the motivation to make this happen, and a big thanks to my audience for forgiving my video-editing non-skills.
Some of the software used in creating this: Sage Mathematics Software, Manim, VPython, p5.js, Krita, Audacity, Kdenlive.
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Music used in the video:
Walk Through the Park -- TrackTribe
George Street Shuffle -- Kevin MacLeod
Quarter Mix -- Freedom Trail Studio
Love Struck -- E's Jammy Jams
George Street Shuffle by Kevin MacLeod is licensed under a Creative Commons Attribution 4.0 license. creativecommons.org/licenses/by/4.0/
Source: incompetech.com/music/royalty-free/index.html?isrc=USUAN1300035
Artist: incompetech.com/
Переглядів: 100 518
Відео
The Intuition behind the Double-And-Add / Square-And-Multiply Algorithm (Also Just a Fun Puzzle!)
Переглядів 2,7 тис.3 роки тому
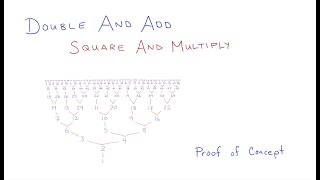
This video develops the Double-And-Add Algorithm entirely intuitively through a fun recreational math puzzle, so you will always be able to recreate it for yourself and use it when you need it. (Also known as Square-And-Multiply and occasionally as the Chanda Sutra Method.) The video requires that you are familiar with binary. Here's the tree I draw in the video: oeis.org/A232559 (In my video, ...
ADFGVX Cipher: Encryption and Decryption (Updated)
Переглядів 19 тис.3 роки тому
Encryption and Decryption of the World War One ADFGVX Cipher, through examples. This video replaces an earlier one with the same content, but has some improvements and corrections. #cryptography
Lehmer Factor Stencils: A paper factoring machine before computers
Переглядів 54 тис.3 роки тому
In 1929, Derrick N. Lehmer published a set of paper stencils used to factor large numbers by hand before the advent of computers. We explain the math behind the stencils, which includes modular arithmetic, quadratic residues, and continued fractions, including my favourite mathematical visualization for continued fractions. *VIDEO CORRECTION*: I made a copying error when setting up the recurren...
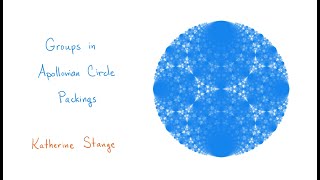
Studying Apollonian Circle Packings using Group Theory
Переглядів 6 тис.4 роки тому
This video is in response to a colleague who asked for short videos about how we use group theory in our research. One of my interests is Apollonian circle packings. Some links: Wikipedia: en.wikipedia.org/wiki/Apollonian_gasket An article in New Scientist by Dana Mackenzie: www.americanscientist.org/article/a-tisket-a-tasket-an-apollonian-gasket A good math paper to start: arxiv.org/abs/math/0...
The modular inverse via Gauss not Euclid
Переглядів 2,6 тис.4 роки тому
We demonstrate a lesser-known algorithm for taking the inverse of a residue modulo p, where p is prime. This algorithm doesn't depend on the extended Euclidean algorithm, so it can be learned independently. This is part of a larger series on modular arithmetic: ua-cam.com/play/PLrm9Y qlNyWBQEKBSrr_Uh16gEWhJzw.html
The extended Euclidean algorithm in one simple idea
Переглядів 13 тис.4 роки тому
An intuitive explanation of the extended Euclidean algorithm as a simple modification of the Euclidean algorithm. This video is part of playlist on GCDs and the Euclidean algorithm: ua-cam.com/play/PLrm9Y qlNxXccpwYQfllCrHRJWwMky-.html
ADFGVX Cipher: Encryption and Decryption (OLD VERSION: SEE NEW VERSION LINK BELOW)
Переглядів 11 тис.4 роки тому
This video has been replaced with an updated video: visit ua-cam.com/video/T0xfKiU9Rr4/v-deo.html Encryption and Decryption of the World War One ADFGVX Cipher, through examples.
Cryptanalysis of Vigenere cipher: not just how, but why it works
Переглядів 34 тис.4 роки тому
The Vigenere cipher, dating from the 1500's, was still used during the US civil war. We introduce the cipher and explain a standard method of cryptanalysis based on frequency analysis and the geometry of vectors. We focus on visual intuition to explain why it works. The only background needed is some familiarity with vectors and probabilities. For more on this method: _Introduction_to_Cryptogra...
The Euclidean Algorithm: How and Why, Visually
Переглядів 37 тис.4 роки тому
We explain the Euclidean algorithm to compute the gcd, using visual intuition. You'll never forget it once you see the how and why. Then we write it out formally and do an example. This is part of a playlist on GCDs and the Euclidean algorithm: ua-cam.com/play/PLrm9Y qlNxXccpwYQfllCrHRJWwMky-.html
Greatest common divisor and least common multiple: building blocks
Переглядів 3,1 тис.4 роки тому
The intuition behind gcd and lcm, from the perspective of factorizations. For aspiring mathematicians already familiar with prime numbers as the building blocks of integers. Learn the intuition, the definitions, and gain some practice. This video is appropriate for high school, for an introduction to proof course, for undergraduate mathematics majors, or for the mathematically inclined, especia...
Modular Arithmetic: Multiplication in Motion
Переглядів 1,7 тис.4 роки тому
Modular arithmetic visually! We explore multiplication modulo n, and discover and prove that the multiplication-by-a map f(z) = az mod n is bijective if and only if a and n are coprime. We use a visualization tool called a "dynamical portrait." This treatment is inspired by Martin H. Weissman's beautiful book, An Illustrated Theory of Numbers. This video is appropriate for an introduction to pr...
Modular Arithmetic: Addition in Motion
Переглядів 1,6 тис.4 роки тому
Modular arithmetic visually! We explore addition modulo n, and discover and prove the number of cycles and their sizes. We use a visualization tool called a "dynamical portrait." This treatment is inspired by Martin H. Weissman's beautiful book, An Illustrated Theory of Numbers. This video is appropriate for an introduction to proof course, for undergraduate mathematics majors, or for the mathe...
Modular Arithmetic: In Motion
Переглядів 3,3 тис.4 роки тому
Modular arithmetic visually! We use a visualization tool called a "dynamical portrait." We explore addition and multiplication modulo n, and discover and prove the portrait is made of cycles if and only if the function (f(z) = z a mod n or f(z) = az mod n) is bijective. This treatment is inspired by Martin H. Weissman's beautiful book, An Illustrated Theory of Numbers. This video is appropriate...
Modular Arithmetic: Under the Hood
Переглядів 2,4 тис.4 роки тому
Modular arithmetic visually! For aspiring mathematicians already familiar with modular arithmetic, this video describes how to formalize the concept mathematically: to define the integers modulo n, to define the operations of addition and multiplication, and check that these are well-defined. This video is appropriate for an introduction to proof course, for undergraduate mathematics majors, or...
Intro to LaTeX for beginning math majors
Переглядів 1,3 тис.4 роки тому
Intro to LaTeX for beginning math majors
Abelian sandpile animation: toppling of an odometer of integral linear growth
Переглядів 2305 років тому
Abelian sandpile animation: toppling of an odometer of integral linear growth
Abelian sandpile animation: toppling of an odometer of integral quadratic growth
Переглядів 2415 років тому
Abelian sandpile animation: toppling of an odometer of integral quadratic growth
Abelian sandpile animation: toppling of an odometer of rational linear growth
Переглядів 1555 років тому
Abelian sandpile animation: toppling of an odometer of rational linear growth
Abelian sandpile animation: toppling of an odometer of rational quadratic growth
Переглядів 2255 років тому
Abelian sandpile animation: toppling of an odometer of rational quadratic growth
Abelian sandpile animation: toppling of an odometer of rational quadratic growth
Переглядів 3005 років тому
Abelian sandpile animation: toppling of an odometer of rational quadratic growth
The Intuition Behind Proof by Induction
Переглядів 12 тис.6 років тому
The Intuition Behind Proof by Induction
The Dystopian Perspective on Logical Negation
Переглядів 4286 років тому
The Dystopian Perspective on Logical Negation
Anatomy of a proof: if n is even, then n squared is even
Переглядів 13 тис.8 років тому
Anatomy of a proof: if n is even, then n squared is even






@1:34 isnt 5^2 supposed to only be two blocks? Not there?
This was amazing. Thank you.
This video is an absolute gem
Cool
Amazing work 👍🏻
Great, hence THIS IS HOW TO SIMPLIFY FRACTIONS THE EASIEST WAY: If the difference between the numerator and denominator is 1, the fraction cannot be simplified. A. Ask: does the numerator go into the denominator evenly (i.e. without any remainder)? If yes, proceed to B. If not, proceed to C. B. Great! The simplest fraction is 1 over the number of times the numerator fits in the denominator. (e.g. 20/60, 20 fits evenly 3 times, hence 1/3.) C. Write the remainder, (e.g. 20/46, (20 fits 2 times in 46, with a remainder of) 6.) to the right of the fraction, and ask: does the remainder go evenly into the numerator? If yes, proceed to D. If not, proceed to E. D. Great! The "remainder" is the Greatest Common Factor. Divide both the numerator and denominator by the GCF/remainder to have the simplest fraction. E. Write the "new remainder" (using the last example, 20/46, 6 fits into 20 with a "new remainder" of 2) to the right of the previous "remainder", and ask: does the "new remainder" fit evenly into the previous "remainder" (2 into 6)? If yes, proceed to D. If not, proceed to E. Please, (with your amazing talent,) produce a video demonstrating it. //Euclidean Algorithm.
Mind boggling. ❤❤❤
what a great video! after learning about surreal numbers Im trying to get back into maths and this feels like a bridge I was missing from my school knowledge
this is perfect. thanks!
tip bit me😊
swallow😂
its' 13 of lentgh
bro why you are so good at this? we need you in competitive programming community please teach us more of this way of thinking about numbers.
beautiful video
Thanks
after like 6 hrs of constantly thinking, i kinda get how the algorithm work now. basically, if we have 2 natural numbers a and b, we can write them in the form: a = C*ua b = C*ub where C is the least common divisor and u's are the unique divisors the goal here is to reduce u to 1. we can do that by constantly taking remainder division which preserves C if we write a = nb*b+R and substitute a and b in, you will see the remainder always contains C R = C(ua - nb*ub) we will know whether either of the u's is 1 when the remainder is 0 ua = ua - nb*ub makes ua < ub since ua < (nb + 1)*ub (nb is the greatest number of b contained in a, aka a/b) so everytime we take remainder, the larger/smaller side switches making u reduce constantly while staying positive there will NEVER be such C*2*3 and C*2 case since that makes C*2 the gcd, wich contradicts with the claim that C is the gcd
This + thinking gives refreshing perspective on what the heck my lecturer been trying to get to me. It's always the fact that you need to process raw information first, before understanding it normally.
The quotes of the beauty of math outside practical applications sum up math programs I've written. Haven't contributed much to my career with it...
this is the best explanation i ever have❣❣
Whaaaaaaaaaaaat?! Wow! thank you so much for this visual example, it's so good and can be configured for an activity on one of my classes!
I love you so mucchhh. This makes Visually tremendously more sense…
the best explanation, much much easier but the problem is lecture just want exactly like how they explain
Thank you very much ❤❤❤
Wow, this video is simply amazing, not only do you help us visualize the algorithm but you also make it intuitive to the point where you can derive it yourself. Thank you so much! I will be sure to check your videos out for future concepts!
SO UNDERRATED
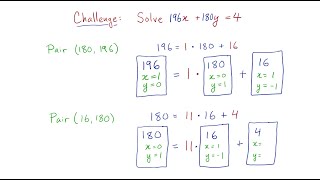
At 4:53 you write: 180=11*16+4 but correct would be +20 instead of +4 and then you get 20=16+4 followed by 16=4*4+0 and gcd=4
7:14 I said "Ohhhh" out loud as now I think I get it.Thank you
The best explanation on this topic
Wouldn't it be easier to factor the 10 million numbers and publish a table lol.
Great video that i watch every once in a while.
damnnnnn bro! 🤯🤯
Best explaination ever made for this topic by just one joing lines of a traingle you explained the smallest detail. Thank you very much for this it was so much helpful.
почему нет руского перевода??? правильно ютуб в России забанили поделом
In terms of an orchid problem, I like Phi, rhe golden ratio instead if pi. Since it's "the most irrational number" meaning there is no good approximations. This approach or even the surreal numbers, allow for ording all the irrationals. so doesnt that allow a mapping from rationals to irrationals and showing that they have the same cardinality? Ruining the second diagonal argument? Instead of numebrs, you can also do this with powersets over sll rationals.
Florence Union
thank you for the awesome content
i truly wish i saw this video first years ago. how many times i have given up, confused on the notations, given its apparent inconsistency in use. now that you pointed out that it is a side note, it is so clear now. thank you. just wish i saw this years ago.
Its been a month since I graduated engineering, and now is the day when I truly understand this algorithm
4 years for me haha
If you just have L/R options, would there be a significant difference between using fairy* subdivisions instead of binary ones? Also, the fractions you put on the grid don't quite make sense to me. (1,1) is further from the origin than (0,1) and (1,0), so it seems like it should represent 1/2 instead, since it looks smaller, with (1,0) representing 1, (1,2) and (2/1) representing 1/3 and 2/3, (1,3) and (3/1) representing 1/4 and 3/4, and so on. *[sic is what you get for being called Farey and not spelling it out :P]
Williams Ronald White Frank Thomas Anna
UA-cam has a lot of trash on it - and then it has things like this. I think this is a serious contender for the best STEM-related video I've ever seen.
Here's how to calculate the numbers used in the sequence of a continued fraction. You take the number, - the biggest whole number you can find, then flip the remainder as a fractional addition, and then repeat. ie: 3.14 - 3 = .14, 1/.14= 7... 1/... = 15... 1/...= 1... 1/... = etc where 3,7,15,1 would be the 'address' instead of 3,1,4,...(base 10 address) Loved the video, and now that i understand the process to generate continued fractions, I agree with you, it's actually VERY easy to calculate the continued fraction. It only requires simple subtraction and division, where as calcuating base 10 of a number also requires simple subtraction and division. Now getting the actual 'value' back from a continued fraction, is a lot more involved (every address you go, requires another division and addition (and the addition involves multiplication), where as with any normal base address it only requires 1 simple multiplication per address, so indexing in is much easier than indexing out, which means numberical bases are here to stay, like you said, but its still quite clever and I loved thinking through the arguments and watching the visualization. Edit: I did some more thinking about this, and actually you need the base 10 system to write out the continued fraction in the first place. So this is an index, of an index. If we wrote all the numbers in base 2, the continued fraction would look way different. Doesn't change much, but I did overlook that, this isn't a 'better' address divorced of the base 10 address, it's an even more specific, base 10 address.
honestly, this was so cool
Thanks for your explanation! Just a quick correction, in your example, the number of dots is 57 instead of 54. gcd(21, 57) = 3 is still true tho.
i watched your video a few months ago and ive been thinking about it constantly, its changed the way i view number! super thanks!
Love you
I am interested in understanding how things work rather than memorization, and in less than a minute of the video, I knew it was special. Content such is this is absolutely vital. Thanks.
Beautiful
This is brilliant. Please continue to make more such videos. This is how science and math must be seen.
At first, I didn't quite grasp why would the GCD remain same after we delete the smaller number from larger one (B-A). But it made sense this way: Hint: We are deleting pile A from pile B and then ask what's the new GCD of leftover pile B and the pile A? Well, just remember, the deletion is also made of new GCD as we just deleted pile A- hence the whole pile B and pile A have a new GCD ;) contradiction ! Explanation: GCD is basically the largest chunk of stones that will divide both piles in some number of parts, say- xa and xb. So, pile A has xa number of GCDs and pile B has xb number of GCDs (largest chunks common for both). => A = g . xa and B = g . xb (Imagine them as bigger balls that make up the pile) Now, we remove just one copy of pile A from B. This means: => B - A = g . xb - g . xa For a moment, let's assume, the common chunk size of A and B-A, could maybe get bigger after deletion- to say g' (read: g dash) => B - A = g' . x' and A = g' . xa' This means, the leftover of pile B is made of g' size chunks with count as x' and pile A is made of g' size chunks with count as xa'. But, here's the catch: the deleted pile A from pile B must also be made of g' size chunks with count as xa'. That means: => deleted pile A + left over pile B = the original pile B => g' . xa' + g' . x' = pile B => g' (xa' + x') = pile B So, the pile B is made of g' size chunks AND pile A is also made of g' size chunks! A common divisor for A and B! What's the largest common divisor for A and B? => The GCD(A, B) = g Hence, g' = g, the original GCD of A and B!