Real Analysis 48 | Riemann Integral - Partitions
Вставка
- Опубліковано 9 лют 2025
- 📝 Find more here: tbsom.de/s/ra
👍 Support the channel on Steady: steadyhq.com/e...
Other possibilities here: tbsom.de/sp
You can also support me via PayPal: paypal.me/brig...
Or via Ko-fi: ko-fi.com/theb...
Or via Patreon: / bsom
Or via other methods: thebrightsideo...
Join this channel on UA-cam: / @brightsideofmaths
💬 Access to the community forum: thebrightsideo...
🕚 Early access for videos: thebrightsideo...
❓ FAQ: thebrightsideo...
🛠️ What tools do you use: thebrightsideo...
📚 Download my books: thebrightsideo...
🆓 Ad-free access to all videos: thebrightsideo...
Please consider to support me if this video was helpful such that I can continue to produce them :)
Each supporter gets access to the additional material. If you need more information, just send me an email: tbsom.de/s/mail
Watch the whole video series about Real Analysis and download PDF versions, quizzes and exercises: tbsom.de/s/ra
Supporting me via Steady is the best option for me and you. Please consider choosing a supporter package here: tbsom.de/s/sub...
🌙 There is also a dark mode version of this video: • Real Analysis 48 | Rie...
🔆 There is also a bright mode version of this video: • Real Analysis 48 | Rie...
🔆 To find the UA-cam-Playlist, click here for the bright version: • Real Analysis
🌙 And click here for the dark version of the playlist: • Real Analysis [dark ve...
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
This is my video series about Real Analysis. We talk about sequences, series, continuous functions, differentiable functions, and integral. I hope that it will help everyone who wants to learn about it.
x
#RealAnalysis
#Mathematics
#Calculus
#LearnMath
#Integrals
#Derivatives
#Studying
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
For questions, you can contact me: steadyhq.com/e...
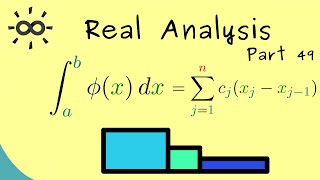
![Real Analysis 49 | Riemann Integral for Step Functions [dark version]](http://i.ytimg.com/vi/Ewwk0pHeiQk/mqdefault.jpg)
![Real Analysis 49 | Riemann Integral for Step Functions [dark version]](/img/tr.png)






You really help me understand this subject. My only regret is that I didn't discover you sooner!
Thank you very much :)
Honestly thankful for the series.. went 😂 to class and all I could recall is how clearly you explained everything
More good material! Thanks 😊
The lecture is so crystal clear and to the point ❤
Thanks a lot :) And thanks for your support!
If I recall correctly all you have to do to give the Riemann integral more power than Lebesque is to allow the widths of the partitioning of the domain to depend on x when taking the limit. The concept was developed Ralph Henstock. But the Lebesque intergal is nicer to work with when the domain is complicated (multi-dimensional or not R).
To be honest, I don't know this Henstock partition idea. Do you have a good reference for this?
I always wondered what analysis II is about and how is it studied, thanks!
It becomes very simple when you teaching that
Thanks! That was my goal :)
At 4:25 you mentioned that you have lessons on Lebesque integral as separate series. Which series cover that topic?
Measure Theory :)
Hier you can find it: thebrightsideofmathematics.com/measure_theory/overview/
@@brightsideofmaths Thanks a lot. I wish I had access to these material before starting my PhD! I am sure these material will help so many of those desperate people along their projects!
Does this lesson, Reimann integral, apply in Real analysis II? I hope that makes sense. Thank you.
Could you elaborate on the difference between Riemann integral, darboux integral and Riemann stieltjes integral? Which is stronger and more general?
This is something for a separate video :)
@@brightsideofmaths will be highly awaited! 🖤
Was this shot in OneNote? :)
No :)
@@brightsideofmaths :O on what then?
@@AlvaroALorite Xournal :)
@@brightsideofmaths which mic you use? Your voice sound so soothing
respect
Thanks :D
صوتو حلو 🤦🏻♀️😂.
Please work on your pronunciation I can't concentrate
Haha, I already do that :)