IMO 2022 P2 Review (Norway)| A Functional Inequality?

#IMO​ #2022 #Problem2 #Algebra #functional_inequality
In this video we review this year's IMO 2022, which was held in Norway, we solve Problem 2, which is a very cool functional inequality.
IMO 2022 Playlist: • IMO 2022 (Norway) review
IMO Past Problems: • IMO Past Problems
Functional Equations playlist: • Functional Equations T...
Ultimate Geometry playlist: • The Ultimate Geometry ...
Shorts: • Short Math videos
__________________________________________________________________________________________
I upload videos concerning math Olympiad contests to help students preparing for math Olympiad and all people who share the love for some interesting math problems.
Don't forget to subscribe to the channel!
__________________________________________________________________________________________
Email me your thoughts! ([e-mail])
Donate: www.paypal.com...

[@Simio_Da_Tundra]

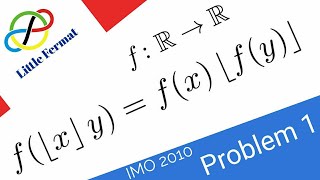
A simpler way to wrap things up:
We know that f(x)≤1/x. Suppose that for some x f(x)0 such that f(x)=1/(x+ε). Then, we know that y=x works (because 2xf(x)=2x/(x+ε)

[@fergalmdaly]

Thanks for the solution. The limit argument is very elegant but was surprising to me. When I did this I got the same sequence of results but for the last step, I thought "if it's < 1/x at some point x and x~y for some y!=x then that's going to force f(y) to be > 1/y somewhere near there". Instead of taking that limit, you look at it as a quadratic. The rule requires it to be > 2. You need to throw out the term that is known to be negative and then you can show that the remaining quadratic has a 2 roots and that one of them is > 0 and therefore a region in R+ where it goes < 0, contradicting the rule.
It's not as elegant as the limit but the idea that pushing f(x) away from 1/x in one spot is going to require it pushed in the opposite direction somewhere else is intuitive and applicable to other problems like this.

[@littlefermat]

Nice!
Indeed the limit and defining g is not the only way ;-)

[@fergalmdaly]

@@littlefermat actually I defined g. In fact I defined g at the start, which actually makes it harder to prove the earlier facts 😂

[@sithlordbinks]

Wow... very nice thank you little fermat sir!!!

[@deltaa-x4940]

PLEASE WE NEED THAT IN THE ARABIC CHANEL

[@deltaa-x4940]

Sir, when we get f(x+1)-f(x)=1
For f:Q--->Q
What's the which we can use to prove that f is linear

[@sithlordbinks]

Well this means that when x increments by 1, f(x) increments by 1, for every x, so use induction