How to Denest a Radical in Two Ways
Вставка
- Опубліковано 23 жов 2023
- 🤩 Hello everyone, I'm very excited to bring you a new channel (aplusbi)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
/ @sybermathshorts
/ @aplusbi
⭐ Join this channel to get access to perks:→ bit.ly/3cBgfR1
My merch → teespring.com/stores/sybermat...
Follow me → / sybermath
Subscribe → ua-cam.com/users/SyberMath?sub...
⭐ Suggest → forms.gle/A5bGhTyZqYw937W58
If you need to post a picture of your solution or idea:
intent/tweet?text...
#radicals #radicalexpressions #algebra
via @UA-cam @Apple @Desmos @NotabilityApp @googledocs @canva
An Exponential Equation | 2^{3^{5^x}} = 3^{2^{5^x}}: • An Exponential Equatio...
A Surprising Exponential Equation: • A Surprising Exponenti...
PLAYLISTS 🎵 :
Number Theory Problems: • Number Theory Problems
Challenging Math Problems: • Challenging Math Problems
Trigonometry Problems: • Trigonometry Problems
Diophantine Equations and Systems: • Diophantine Equations ...
Calculus: • Calculus



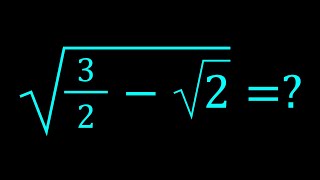





Set √(3 - √5) = √a - √b (use the sign on RHS same as the one inside the nested radicals), where a > b.
Squaring both side:
3 - √5 = (√a)² + (√b)² + 2√ab
= (a + b) + √(4ab) .
Comparing like terms, we get system:
a + b = 3 and 4ab = 5.
Solving b from the 1st equation & subbing into 2nd:
4a(3 - a) = 5
12a - 4a² = 5
4a² - 12a + 5 = 0
(2a - 5)(2a - 1) = 0
So, a = 5/2 or a = 1/2.
When a = 5/2, b = 1/2, and when a = 1/2, b = 5/2.
Since we need a > b, we choose 1st case (a,b) = (5/2,1/2).
So:
√(3 - √5) = √a - √b
= √(5/2) - √(1/2)
= √(10/4) - √(2/4)
= ( √10 - √2 )/2 Done!
That is the method I use, just did it exactly the same way.
Sure, but you can simplify this procedure a bit because you don't have to solve a quadratic equation. Once you have the system
a + b = 3
4ab = 5
you can note that
(a − b)² = (a + b)² − 4ab = 9 − 5 = 4
which implies
a − b = 2
since a − b > 0. With a + b = 3 and a − b = 2 we immediately find
a = ½((a + b) + (a − b)) = ½(3 + 2) = ⁵⁄₂
b = ½((a + b) − (a − b)) = ½(3 − 2) = ¹⁄₂
Nice!
I was getting ready to tell you about a cool way to solve it, but then you got to Method 2 and spoiled it for me! Great stuff!
Ooopsies! Sorry about that 😁
@@SyberMath -- I'm subscribed to your channel, but UA-cam stopped showing me your stuff a few months ago. It's strange how that works.
I agree 100% that knowing the first method is important, but un general one should always check the second first. Great video.
Thank you!
My method is to make the ansatz
x + sqrt(y) = sqrt(3 - sqrt(5))
and to square this equation:
(x + sqrt(y))^2 = (sqrt(3 - sqrt(5)))^2
Use the binomic formula on the left side:
x^2 + 2*x*sqrt(y) + y = 3 - sqrt(5)
Reorder the left side:
x^2 + y + 2 *x*sqrt(y) = 3 - sqrt(5)
and then make a coefficient comparison: this equation is surely fulfilled if
x^2 + y = 3
2*x*sqrt(y) = sqrt(5)
To solve this equation system, square the second equation:
4 * x^2 * y = 5
and divide it by 4. Thus we have the two equations
x^2 + y = 3
x^2 * y = 5/4
Since we know the sum and the product of the two variables x^2 and y,
we can, according to Vieta theorem, compute them from the the quadratic equation
t^2 - 3t + 5/4 = 0
or, multiplied by 4:
4t^2 - 12t + 5 = 0
The quartic formula gives the two solutions for t:
t = (-(-12) +- sqrt(12^2 - 4*4*5))/(2*4)
t = (12 +- sqrt(144 - 80))/8
t = (12 +- sqrt(64))/8
t = (12 +- 8)/8
t = 20/8 or t = 4/8
t = 5/2 or t = 1/2
This means that either
x^2 = 5/2 and y = 1/2
x = +- sqrt(10)/2 and sqrt(y) = sqrt(2)/2
or
x^2 = 1/2 and y = 5/2
x = +- sqrt(2)/2 and sqrt(y) = sqrt(10)/2
and we have the four solution candidates
(sqrt(2) + sqrt(10))/2 = 2.288...
(sqrt(2) - sqrt(10))/2 = -1.748...
(sqrt(10) + sqrt(2))/2 = 2.2888...
(sqrt(10) - sqrt(2))/2 = 0.874...
And since
sqrt(3 - sqrt(5)) = sqrt(3 - 2.236...) = sqrt(0.7639...) = 0.874...
only the last solution is correct:
sqrt(3 - sqrt(5)) = (sqrt(10) - sqrt(2))/2.
For the fiirst method, we can eliminate the candidate with both positive signs by estimation. 3-sqrt(5) < 1, so its square < 1. But, (2+sqrt(10))/2 is > 1, thus it is not the answer.
√{a - b√c} (a,b,c being rationals) can be simplified to √y - √z iff {a² - b².c} = d²
with d rational. If this is so, take y=(a+d)/2 & z=(a-d)/2. Here a=3, b=1, & c=5.
∴ d = √{3² - 1².5} = √{9-5} = √4 = 2. So y = {3+2}/2 = 5/2 and z = {3-2}/2 = 1/2.
∴ √{3-√5} = √y-√z= √(5/2)- √(1/2)= √(10/4)- √(2/4) =(√10- √2)/√4 =(√10- √2)/2.
I did this by setting the original equation equal to root( (a+root(5)b)^2 )
You end up solving a quartic system for a and b and you plug in the original solutions
4:40 You can just check it numerically: sqrt(5) is between 2 and 3, so 3 - sqrt(5) is between 0 and 1, and so is the square root of it. The first solution candidate, sqrt(10) - sqrt(2) is about 3,2 - 1,4 = 1,8, divided by 2 is 0,9 (a calculator says both radicals have a value of about 0.874). The second candidate is way too large, because sqrt(10) + sqrt(2) is about 3.2 + 1.4 = 4.6, and the half of it is 2.3. Thus the first candidate must be the solution.
I solved this using the identity (a + b sqrt(5))^2 = 3 - sqrt(5)
we get a^2 + 5b^2 = 3
and 2ab = -1.
Put b = -1/2a and substitute back into the previous equation.
a^2 + 5 / (4a^2) = 3
4a^4 - 12a^2 +5 = 0
a^2 = (12 +- sqrt(144 - 4*4*5)) / 8
a^2 = (12 +- 8)/8 = 5/2 or 1/2.
a = +- sqrt(5/2) or +- (1/2)
b = -+ 1/ 2 sqrt(5/2) = +- 1/sqrt(10); or b = +- 1/sqrt(2)
So a + b sqrt(5) = +- sqrt(5/2) -+ sqrt(5)/sqrt(10) = +- (sqrt(10) - sqrt(2) ) / 2
The same answer when using the second pair of (a,b).
The 2nd method is easy n simple for student
7:25 how can one possibly know this rearangement by just looking at the terms? Seems to me like solving it backwards once you already know the solution
Sure. this may not always come to you naturally but after seeing a problem of this type, it'll be easier. If you ever squared a radical like sqrt(a) + sqrt(b) then it'll be more straightforward
can you extend the idea to simplify answer from carnoda formula . Let look at this (20-(392)^0.5)^1/3 + (20+(392)^0.5)^1/3 = 4
Generally that cannot be done. Any attempt to do so algebraically will lead to a cubic equation which is either identical with or equivalent with
x³ − 6x − 40 = 0
but solving this with the cubic formula will give you exactly the expression you give, so you will have come full circle without ever getting what you want. To understand at a deeper level why this is so you need some very advanced mathematics.
However, it _is_ possible to denest each of the two cube roots you give, but only because the sum of these two cube roots is _rational_ and known. The cubic equation
x³ − 6x − 40 = 0
has a root x = 4 as we can easily verify. This means that according to the factor theorem the cubic polynomial at the left hand side has a factor (x − 4) and indeed the equation can be written as
(x − 4)(x² + 4x + 10) = 0
The other two roots of the cubic equation are the roots of the quadratic equation x² + 4x + 10 = 0. But this quadratic equation has the complex roots x = −2 + i·√6 and x = −2 − i·√6, so x = 4 is the _only_ real root of the cubic equation.
Now, using Cardan's formulas, the real root of this cubic equation can be expressed as
∛(20 + √392) + ∛(20 − √392)
Since √392 = √(196·2) = √196·√2 = 14√2 this can also be written as
∛(20 + 14√2) + ∛(20 − 14√2)
This evidently represents a real number and since we know that 4 is the only real root of the cubic equation, we can be certain that we have
∛(20 + 14√2) + ∛(20 − 14√2) = 4
But now let's suppose that each of these cube roots can be denested and that we have
∛(20 + 14√2) = a + √b
where a and b are rational numbers and √b is irrational, then we must also have
∛(20 − 14√2) = a − √b
It is easy to see why this is so. If we cube a + √b and a − √b then we have
(a + √b)³ = (a³ + 3ab) + (3a² + b)√b
(a − √b)³ = (a³ + 3ab) − (3a² + b)√b
So, cubes of conjugates such as a + √b and a − √b are again conjugates. This means that if we have ∛(20 + 14√2) = a + √b and therefore (a + √b)³ = 20 + 14√2 then we must also have (a − √b)³ = 20 − 14√2 and therefore ∛(20 − 14√2) = a − √b.
Clearly, if ∛(20 + 14√2)) = a + √b and ∛(20 − 14√2) = a − √b then
∛(20 + 14√2) + ∛(20 − 14√2) = 4
implies that we have
(a + √b) + (a − √b) = 4
and so 2a = 4 and therefore a = 2. To find the value of b, note that we have
∛(20 + 14√2)·∛(20 − 14√2) = ∛((20 + 14√2)·(20 − 14√2)) = ∛((400 − 392)) = ∛8 = 2
and since ∛(20 + 14√2) = a + √b and ∛(20 − 14√2) = a − √b this implies that we have
(a + √b)(a − √b) = 2
or
a² − b = 2
But we already know that a = 2, so we have 4 − b = 2 and therefore b = 2. We can now conclude that we have
∛(20 + 14√2) = 2 + √2 and ∛(20 − 14√2) = 2 − √2
and this can of course be verified by cubing 2 + √2 and 2 − √2.
0:39 2^½? 7:21 x⁴= 9-5
@SyberMath Pls. pls. explain us why (2^0.5 - 10^0.5)/2 is not a legitimate solution
Silly me. I squared the given expression giving me 3-root 5. Then I multiplied that by its conjugate giving me 9+5 = 14. I have a lot to re-learn I suppose.
Yes, that's silly, because apparently you didn't even use the identity (a − b)(a + b) = a² − b² correctly:
(3 − √5)(3 + √5) = 3² − (√5)² = 9 − 5 = 4
But if you want to use conjugates anyway, how about this. Let
√(3 + √5) + √(3 − √5) = A
√(3 + √5) − √(3 − √5) = B
If you square both these equations (and, please, do it correctly this time) then you will find that
A² = 10
B² = 2
Since A and B are positive, we have A = √10, B = √2, so
√(3 + √5) + √(3 − √5) = √10
√(3 + √5) − √(3 − √5) = √2
Now, if you add these two equations (well, actually, identities) you get
2√(3 + √5) = √10 + √2
so
√(3 + √5) = ½√10 + ½√2
and if you subtract the second identity from the first you get
2√(3 − √5) = √10 − √2
so
√(3 − √5) = ½√10 − ½√2
@@NadiehFan. Sir your solution is the best and easiest solution. I understand it perfectly well. I like your maths skill. Thank you so much.
Please post your solution not under someone's comment, so that others will see it and be grateful to you.
@@herolivesnu Thank you. I'll create a new main comment where I explain this method so others can see it as well without going through all the answers on other comments.
@@NadiehFan i guess you told me!
Third method: sqrt(3-sqrt(5)) = sqrt(2)*(phi-1)
why not (2^0.5 - 10^0.5)/2
the expression you wrote there is clearly negative (10>2 so sqrt(10) > sqrt(2) so sqrt(2) - sqrt(10) < 0), and the initial expression is positive, since the sqrt function cannot return a negative output
Here's an alternative method to denest nested square roots which takes advantage of conjugates. First note that using the identity (a − b)(a + b) = a² − b² we have
(3 − √5)(3 + √5) = 3² − (√5)² = 9 − 5 = 4
Now suppose that
√(3 + √5) + √(3 − √5) = A
√(3 + √5) − √(3 − √5) = B
If we square both these equations then, using the identities (a + b)² = a² + 2ab + b² and (a + b)² = a² − 2ab + b², we find that
(3 + √5) + 4 + (3 − √5) = A²
(3 + √5) − 4 + (3 − √5) = B²
because 2·√(3 + √5)·√(3 − √5) = 2·√((3 + √5)(3 − √5)) = 2·√4 = 2·2 = 4. So, all the square roots cancel and we simply end up with
A² = 10
B² = 2
Since A and B are positive, we have A = √10, B = √2, so we now know that
√(3 + √5) + √(3 − √5) = √10
√(3 + √5) − √(3 − √5) = √2
If we add these two equations (well, actually, identities) we get
2√(3 + √5) = √10 + √2
so
√(3 + √5) = ½√10 + ½√2
and if we subtract the second identity from the first we get
2√(3 − √5) = √10 − √2
so
√(3 − √5) = ½√10 − ½√2
This method can be used to denest any nested square root √(a ± √b) where a and b are positive rational numbers with √b irrational, provided that it _can_ be denested. To find out in advance whether or not a nested square root can be denested, you can use the following
*Theorem*
If a and b are positive rational numbers and √b is irrational, then there exist positive rational numbers p and q such that √(a ± √b) = √p ± √q _if and only if_ a² − b is the square of a rational number.
See my other main comment on this video for a proof of this theorem and its application in denesting nested square roots.
The second method for denesting a nested square root presented in this video hardly qualifies as a _method_ and appears rather haphazard. It is not at all clear (unless you already know or can guess the result) why you should start by rewriting 3 − √5 as (6 − 2√5)/2. Denesting nested square roots used to be a topic covered in high school algebra a long time ago, but it is no longer taught in schools and seems to have become something of a lost art.
Denesting nested square roots relies on two theorems, one of which is often taken for granted whereas the other is never even mentioned in videos dealing with denesting nested square roots:
*Theorem 1*
If a, b, c, d are rational numbers and b and d are positive and √b and √d are irrational then a ± √b = c ± √d implies a = c and b = d.
Proof
a ± √b = c ± √d implies ±√b = (c − a) ± √d. Squaring both sides gives b = (c − a)² + d ± 2(c − a)√d and subtracting (c − a)² + d from both sides then gives (b − d) − (c − a)² = ±2(c − a)√d. Now the left hand side is rational, therefore the right hand side must also be rational. But √d is irrational, therefore the right hand side can only be rational if c − a = 0 which implies a = c. With c − a = 0 the equation (b − d) − (c − a)² = ±2(c − a)√d becomes (b − d) − 0 = 0 which implies b = d and this completes the proof.
Next, we have
*Theorem 2*
If a and b are positive rational numbers and √b is irrational, then there exist positive rational numbers p and q such that √(a ± √b) = √p ± √q _if and only if_ a² − b is the square of a rational number.
Proof
First, let a and b be positive rational numbers, √b irrational, and suppose there exist positive rational numbers p and q such that √(a ± √b) = √p ± √q. Squaring both sides then gives a ± √b = p + q ± 2·√p·√q or a ± √b = p + q ± √4pq. Since √b is irrational the left hand side is irrational, therefore the right hand side must also be irrational. Since p and q are rational, p + q is rational, consequently √4pq must be irrational. In accordance with the previous theorem this implies a = p + q and b = 4pq. Consequently, a² − b = (p + q)² − 4pq = (p − q)² is the square of a rational number. Conversely, if a and b are positive rational numbers, √b irrational, and a² − b is the square of a rational number, then p = ½(a + √(a² − b)) and q = ½(a − √(a² − b)) are positive rational numbers with p > q and such that p + q = a and 4pq = b so 2·√p·√q = √b which implies a ± √b = p + q ± 2·√p·√q = (√p ± √q)² so √(a ± √b) = √p ± √q which completes the proof.
For √(3 − √5) we have 3² − 5 = 9 − 5 = 4 = 2² so theorem 2 guarantees that this nested square root can be denested, that is, two positive rational numbers x and y exist such that
√(3 − √5) = √x − √y
Squaring both sides gives
3 − √5 = x + y − 2·√x·√y
or
3 − √5 = x + y − √4xy
Since the left hand side is irrational, the right hand side must also be irrational. But x and y are rational, therefore x + y is rational. Consequently, √4xy must be irrational. In accordance with theorem 1 we therefore have
x + y = 3
4xy = 5
Using the identity (x − y)² = (x + y)² − 4xy we have
(x − y)² = 9 − 5 = 4
so we have x − y = 2 because √x − √y > 0 implies x − y > 0. From x + y = 3 and x − y = 2 we get
x = ½((x + y) + (x − y)) = ½(3 + 2) = ⁵⁄₂
y = ½((x + y) − (x − y)) = ½(3 − 2) = ¹⁄₂
and therefore we have
√(3 − √5) = √(⁵⁄₂) − √(¹⁄₂)
which can be written as
√(3 − √5) = ½√10 − ½√2
Of course, theorem 2 implies that we also have
√(3 + √5) = ½√10 + ½√2
Wow dude, really cool proof (honestly), where did you learn this stuff?
@@GreyJaguar725 All from old (19th century) high school algebra books.
It's actually pretty clear "why you should start by rewriting 3 − √5 as (6 − 2√5)/2", although it could perhaps have been made more explicit. The aim is to try and rewrite 3 − √5 in a form akin to a² + b² + 2ab. For this to be possible at all, then √5 must corresponds to the 2ab part. But since there is no "2" apparent in "√5", it must be reintroduced somehow. To that end, you can rewrite √5 as 2√(5/4), or (2√5))/2. Then 3 − √5 becomes (6 − 2√5)/2 just so you have a common denominator throughout. One last and very basic manipulation gives you (5 + 1 - 2√5)/2, exactly the type of a² + b² + 2ab expression we were looking for to begin within. Nothing haphazard about it.
Instead of "haphazard," you could accurately classify it as "not straightforward."
@@fadetoblah2883 Thank you for the clarification. That's the motivation behind this method and it's pretty standard in my opinion.