Real Analysis 52 | Riemann Integral - Examples
Вставка
- Опубліковано 9 лют 2025
- 📝 Find more here: tbsom.de/s/ra
👍 Support the channel on Steady: steadyhq.com/e...
Other possibilities here: tbsom.de/sp
You can also support me via PayPal: paypal.me/brig...
Or via Ko-fi: ko-fi.com/theb...
Or via Patreon: / bsom
Or via other methods: thebrightsideo...
Join this channel on UA-cam: / @brightsideofmaths
💬 Access to the community forum: thebrightsideo...
🕚 Early access for videos: thebrightsideo...
❓ FAQ: thebrightsideo...
🛠️ What tools do you use: thebrightsideo...
📚 Download my books: thebrightsideo...
🆓 Ad-free access to all videos: thebrightsideo...
Please consider to support me if this video was helpful such that I can continue to produce them :)
Each supporter gets access to the additional material. If you need more information, just send me an email: tbsom.de/s/mail
Watch the whole video series about Real Analysis and download PDF versions, quizzes and exercises: tbsom.de/s/ra
Supporting me via Steady is the best option for me and you. Please consider choosing a supporter package here: tbsom.de/s/sub...
🌙 There is also a dark mode version of this video: • Real Analysis 52 | Rie...
🔆 There is also a bright mode version of this video: • Real Analysis 52 | Rie...
🔆 To find the UA-cam-Playlist, click here for the bright version: • Real Analysis
🌙 And click here for the dark version of the playlist: • Real Analysis [dark ve...
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
This is my video series about Real Analysis. We talk about sequences, series, continuous functions, differentiable functions, and integral. I hope that it will help everyone who wants to learn about it.
x
#RealAnalysis
#Mathematics
#Calculus
#LearnMath
#Integrals
#Derivatives
#Studying
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
For questions, you can contact me: steadyhq.com/e...








My Calculus I & II Professor (Tony Tromba, UC Santa Cruz, Fall 1981) dropped the Dirichlet Function on us at the end of a Friday lecture to give something to snack on during Happy Hour.
I'm taking a foundations of analysis course and I'm struggling to understand the content, so in searching I found your videos "among others, but yours being particularly enlightening". With that said, do you have a preferred textbook for learning real analysis that you could recommend? Your videos are helping me succeed as a better person, I honestly cant thank you enough Sir!
I don't have a preferred text book but I really like Introductory Real Analysis
by A. N. Kolmogorov; S.V. Fomin.
Excellent explanation ! Thank you sir .
Awesome Explanation!!!! Now i understand what my lecturer has been saying.
Thank you!
Thank you very much :)
Thank you for what you are doing.
My pleasure! :)
This is almost the end in a real analysis course, what's the direction next? I have enjoyed this series very much rewatched a few episodes to remind me a few important points.
There is still a lot to cover about the Riemann integral. However, after this, my Real Analysis course ends. Other (related) topics will be discussed in another series :)
@@brightsideofmaths it's been an absolute Joy I'll continue with complex analysis, another outstanding series, recommended.
@@evionlast Thanks :)
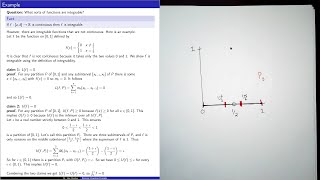
Concerning the Riemann integral of the Dirichlet function, at 3:43 we have *“the rational points lie dense in the real number line”* (although this expression is not defined). Then at 4:25 we have *_“for any segment you choose on the real number line, you always find a rational number”._*
How are we to understand the above statement, when we also have the statements:
(1) the rational numbers are “countably infinite”
(2) the real numbers are “uncountably infinite",
given that an uncountable infinity is a much larger infinity than a countable infinity?
Final Exam Extra Credit Problem: Plot the Dirichlet Function. More points = more points!
can u do multivariable calculus ,partial Derivatives and Tangent Plane also plz
first :) will you cover the riemann stieljes integral? (not sure if that was the correct spelling for steljes)
I think that the Lebesgue-Stieltjes integral is more useful and I already explained this in my Measure Theory series :)
Hello, what software do you use for these videos?
The free and perfect program Xournal :)
@@brightsideofmaths thank you very much for your response!