Volume of Solid bounded by a Cylinder and Planes
Вставка
- Опубліковано 5 лют 2025
- Find the volume of the solid bounded by a cylinder and the planes x=2y, x=0, and z=0. Video contains concept drawings to illustrate the bounded region and discusses the reasons for choosing to integrate with respect to x first.
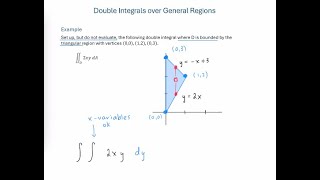


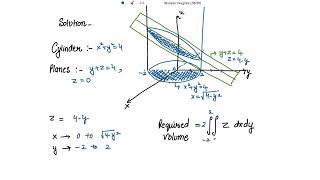





I usually don't comment on videos but I found this to be an absolute gem. I already had solved this problem after watching a multitude of video explanations of this exact problem, but none of them felt sufficient for my understanding. I sort of just went along and blindly agreed with the steps from other videos and it left me with more questions than when I had went into the problem. This video cleared every single question I had. From how to locate the actual region of integration to why dx was taken before dy, you gave such a thorough explanation and the visual representation was definitely a big help. Thank you so much!
Wow! Thank you for the kind complement. I'm a visual learner myself and really need a clear picture to understand what is happening. I'm glad this video helped you.
Why don’t the bounds change after the u-sub?
Because we changed back to the y-variable in the next step. You have two options: 1. change the limits to u and evaluate with u-limits. 2. back substitute the anti-derivative to y so that you can use the original y-limits.