A Complex Log Equation with Natural Log
Вставка
- Опубліковано 8 лют 2025
- 🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
/ @sybermathshorts
/ @sybermath
⭐ Join this channel to get access to perks:→ bit.ly/3cBgfR1
My merch → teespring.com/...
Follow me → / sybermath
Subscribe → www.youtube.co...
⭐ Suggest → forms.gle/A5bG...
If you need to post a picture of your solution or idea:
in...
#ExponentialEquations #ComplexNumbers #Logarithms
via @UA-cam @Apple @Desmos @NotabilityApp @googledocs @canva
A Complex Exponential Equation | iˣ = 2: • A Complex Exponential ...
A Quick and Easy Exponential Equation | iˣ = 1: • A Quick and Easy Expon...
A Nice Exponential Equation | iˣ = e: • A Nice Exponential Equ...
1/ln(x)=-i/2npi
PLAYLISTS 🎵 :
Number Theory Problems: • Number Theory Problems
Challenging Math Problems: • Challenging Math Problems
Trigonometry Problems: • Trigonometry Problems
Diophantine Equations and Systems: • Diophantine Equations ...
Calculus: • Calculus
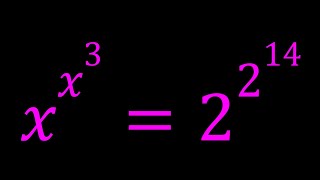








Since the natural logarithm is not defined for nonreal inputs you should use the extended function log(a + bi)= ln(a^2 + b^2) + i*argument of (a+bi)
@gregorysmathchannel5357 I think you should have it √(a^2+b^2) or (ln(a^2+b^2))/2. 🖱👍
A great problem stumping 95% of the students. Thumbs up for your humor today almost catching me in that infinite circular wheel spot who said, "That is definitely not me there n= 0 defining my location, not there also at n not equal to 0 (3:20 in the video hint 🤣😂). So x can never say I was not x at n = 0 ohhh no! Okay n=1, 2, 3, ... , 100, ... Hurry up it's not me ... Take the dang ln(x) so I can prove it wasn't me mathematics. 😂
Now we know what infinity looks like as a finite number.
A beautiful comment!!! 🧡🧡🧡
@@SyberMath lol thanks no problem.
Yes, analitical expansions of even simple functions can yield amazing results. Bravo!
Many thanks!
I made a mistake. The formula should be log(a + bi)= ln((a^2 + b^2)^0.5) + i*argument of (a+bi). In other words take the natural log of the modulus of the complex number and add i times the argument of the complex number.
This isn’t any shorter but it also works.
1 / ln x = -i / 2n(pi)
ln x = 2n(pi) / -i = 2n(pi)i
(ln x) / 2n(pi)i = 1
ln x^[1 / 2n(pi)i] = 1
x^[1 / 2n(pi)i] = e
x = e^[2n(pi)i]
Natural logarithm for complex number is denoted as
LOG () to the base e OR LN ()
this is different from
log () to the base e OR ln ( )
n ( x) = 2 n π /( -i) = 2 n π i
x = exp ( 2 n π i ) = 1
Excelente
Yo... It's gonna be great
x=e^(2nipi)
A bit of a handwave at the end! x = 1 isn't a fully valid solution as you get 1/0 = RHS, which is a problem.
Still, nice problem that you can do from looking at the thumbnail.
ln x = 2n.pi.i
x = exp(2n.pi.i) = cos(2n.pi) + i.sin(2n.pi) = 1
x = e^(2πni)
Crazy ending. So effectively, to amalgamate the two outcomes, x = exp(2nπi), n 0.
There is no solution. e to the 2n*pi*i is always! 1, whatever n element of Z is. The log of any base element of R+ of 1 is always 0. So, the only possible solution is not part of the domain.
This result nobody is catching! Yes it is true x= e^(i(2πn)) x= 1 at n=0, n=1, n=2, n=3, ... That is what the expression means ... I can't believe all those message people saying, good I have, n=1 that is not zero so I have x=e^(i(2π)) so I can quickly take lnx to be 2πi and the same situation 4π, 6π, 8π, ... Where x cyclically equals 1 but never saying x=1 so that they can infinitely say ln(x) is not cyclically equals to 0. 🤣😂 I think this teacher put these comment people in an infinite joke wheel thinking ... And yeahh out at 100π or whatever x is not 1 it is e^i(100π) so we can take a quick ln to get lnx all over again. 😵🤣😂
Then n>0
You broke mathematics. 1 over ln(1) is a complex number.
😜😁