Linearly Independent Solutions and the Wronskian Test

Linearly independent solutions are a fundamental concept in differential equations, critical for understanding the behavior of solutions to linear differential systems. This video provides a detailed explanation of what it means for solutions to be linearly independent and how to use the Wronskian test to determine independence.
The video starts by defining linearly independent and linearly dependent solutions, highlighting their importance in solving differential equations. You will learn why it is essential to identify whether solutions are independent when forming the general solution to a differential equation.
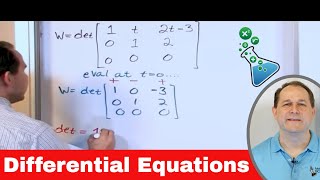
The Wronskian test is introduced as a powerful tool for determining the linear independence of a set of functions. The video explains how to compute the Wronskian determinant and interpret its value to assess independence. Step-by-step examples demonstrate the application of the Wronskian test to various sets of functions, ensuring a clear understanding of the process.
In addition to the technical aspects, the video discusses the significance of linearly independent solutions in the broader context of differential equations and their applications. Visual aids and practical examples help reinforce the concepts, making it easier to grasp the material.
Ideal for students and educators, this video equips you with the knowledge and techniques needed to confidently determine linearly independent solutions using the Wronskian test.
More Lessons: www.MathAndScie...
Twitter: / jasongibsonmath