
- 16
- 761 548
TX Tradesman
United States
Приєднався 17 тра 2021
Welcome to the Texas Tradesman, my name is Ben. This channel is dedicated to metal working tips and tricks as well as welding projects (both useful and for fun). I had the great benefit of having some very good teachers in my life in my profession as a millwright. My goal is to pass some of those useful tips, tricks, shortcuts, and skills onto the next generation through the wonders of social media.
15 Knots For Solving Real World Tasks
This selection of knots can help make your life a little easier. From tying down cargo to lifting and lowering objects and much more. What knots do you think should be included on this list.
60Watt Electric Rope Cutter
Music Track: Looking Back by Chill Pulse
Source: https//freetouse.com/music
Copyright Free Background Music
60Watt Electric Rope Cutter
Music Track: Looking Back by Chill Pulse
Source: https//freetouse.com/music
Copyright Free Background Music
Переглядів: 127
Відео
How To Accurately Find The Center Of A Board
Переглядів 6 тис.7 місяців тому
Though you generally will not need this level of accuracy to find the center of dimensional lumber, this is pretty much a fail proof way of accomplishing that task. Best of all, no measuring involved!
Find The Center Of A Circle With Thales’ Theorem
Переглядів 73 тис.7 місяців тому
One of my favorite methods for finding the center of a circle is Thales’ Theorem. This method can be accomplished over long distances with the aid of a three access laser level and string line.
3 Simple Layout Tricks To Use When Working With Pipe
Переглядів 1,1 тис.7 місяців тому
Although there are a number of specialty tools made for laying out pipe, a number of those tasks can be accomplished with more basic hand tools. This video will highlight three of those examples that I hope will aid you in your future project layouts.
Construct angles 0°, 15°, 30°, 45°, 60°, 75°, 90°, 105°, 120°, 135°, 150°, 165°, and 180°
Переглядів 3267 місяців тому
Using only compass and straight edge numerous angles can be constructed. I’ll go over how this is accomplished in this video.
Koike Aronson Pantograph Torch Cutting 3” Steel
Переглядів 2,6 тис.2 роки тому
I am currently helping out a local company to train their employees to run a new CNC plasma. Today I had the opportunity to run their pantograph torch.
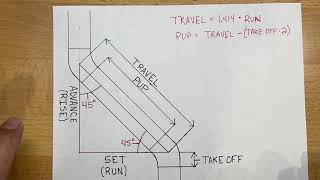
Trades Math - Plumbing 45° Offsets And Finding Constants
Переглядів 14 тис.2 роки тому
In this video we’ll go over the steps involved to calculate the constants for figuring your travel distance for different degree fittings. We’ll also figure our example offset for a 12” offset.
Trades Math - Pitches, Slopes, Grades, And Angles
Переглядів 8882 роки тому
In this video we’ll be looking at the what differentiates angles, slopes, grades, and pitches. I’ll show you how you can calculate angles based on pitches. We’ll also look at a construction calculator that simplifies these functions to help you be more productive. Construction Master Pro - amzn.to/3cTMope Construction Master Pro Spanish Edition - amzn.to/3oIKqKS Construction Master ProDesktop -...
Trades Math - Find The Center Of A Circle Using Squares
Переглядів 625 тис.2 роки тому
One of the easiest methods to find the center of a circle accurately is to use a compass and perpendicular bisectors. However, on a job site a compass is not always readily handy, but you’ll always find squares. So using those squares I’m going to show you how to accurately find the center of a circle using three different methods.
Trades Math - How To Read A Tape Measure
Переглядів 4,7 тис.2 роки тому
Reading a tape measure can be a little overwhelming if you’ve never done it before. Hopefully by the end of this video you will have a better grasp of how to read the tape measure more effectively. We’ll go over some of the characteristics of standard tape measures, how the measurements are broken up, and a couple of tips to help you read the tape measure quicker.
How To Saddle Pipe With A Band Saw
Переглядів 3,4 тис.2 роки тому
With some scrap wood you can make a simple jig to saddle pipe on a band saw. Once your jig is set you just have to mark each side of the next pipe to make fast, repeatable cuts.
Double Miter Corner Joint
Переглядів 4,3 тис.3 роки тому
The double miter corner joint is generally not used in commercial applications, but it does make a nice joint for building furniture. The key to cutting these joints accurately is the setup. To set my saw to 45 degrees, I use a 2x4 cut on a miter saw, check the angle for accuracy, then use the wood as a guide to setup my dry cut saw. I cut the first piece, then measure from the cut to the back ...
Sandblasting Cast Iron Griddle
Переглядів 1,5 тис.3 роки тому
Operator's view blasting in a blast cabinet.
Fabrication Square
Переглядів 20 тис.3 роки тому
Fabrication squares are designed to provide a quick clamp fixture to align part in a 90° or 45° orintation for tacking. These types of squares can run between $50-$150 depending on brand and model. You can save yourself quite a bit of money by building your own with about $5 of parts and some time. While it is important to carefully layout this type of tool to ensure accuracy, it is a relativel...
Bar Bender (1/4" Flat and 3/8" Round)
Переглядів 2,1 тис.3 роки тому
I finally decided to make a bar bender for my shop, something I have needed for quite some time. I built it so that it remains together as a unit. I also utilized 1/2" seamless tubing to act as a bearing of sorts, to make it easier to draw the bends. The handle is a removeable 1" schedule 40 pipe that can be made any length you desire for bends requiring more force.








0:00 The sinner of a circle?
Hey Ben, another great video . Before I started watching your channel I could only tie a couple of simple knots. My knot skills have improved dramatically from watching your videos and following your techniques. Hope you can make more metal fabrication videos in the near future.
Excellent video Ben! Keep em coming. Someone asked me where I learned to tie knots , my response was The Texas Tradesman. You are an excellent teacher even when you say nothing at all.
This is the year 2024 you Idiot!
Did I miss it or was the laser level the way he found the center of the 60' dia silo? was this to lay out a future silo on a pad bigger than 60' giving him a place to physically put the instrument- i don't see where he could have put the instrument inside of an existing silo- or outside of an existing silo (where it would have been useless anyway.)
That’s a good catch. What I had to do was use a 6” spacer to mark out a circle on the concrete that decreased the radius by 6”. Then I was able to set my laser on the smaller circle to pick up two diameters.
@@txtradesman527 Cool.
It works because of another theorem whereby the if an angle has its vertex on the circle the the angular measure of the arc enclosed within the rays of the angle will be twice the angular measure of the angle itself. Using a square (90 degrees) therefore encloses an arc with an angular measure or 180 degrees.
Great example of basic geometry!
How can you do the rafter on basic math
You can get all of the 30 degree lines without changing your compass radius. Once you get the first one by bisecting the 60 degree angle, you can then put the point on of the compass on the 30 degree line to get 30+60 aka 90 and then again on the 90 to get to 150. then after you get the 15 degree line, you can get do the same thing to get 75, & 135, then you only need to reset the compass once to get the remainder of the 15 degree lines.
You’re absolutely correct! Should have done it just the way you suggested. Would greatly reduce any errors from resetting the compass multiple times.
I just run the ruler to the widest spot... run a line... then do it again in the opposing direction and viola... center
The word is indentation. There is no such word as "indention!"
DEFINITION FOR INDENTION (1 OF 1) noun 1. The indenting of a line or lines in writing or printing. 2. The blank space left by indenting. 3. The act of indenting; state of being indented. 4. Archaic. An indentation or notch.
I would suggest that the next time you feel so inclined to attack someone based on your ”perceived” intelligence, you might want to have a clue about what you’re talking about first.
Shall I give you the definition of perceived as well, or do you think your simple minded brain can handle that big word?
GREEN PAPER....!?!?!? ARE YOU NUTS......!?!?!?
Why not just draw a rectangle or square inside the circle and draw lines from the corners? Where they cross is the center of the circle.
1. Thales's _Thales_ is not a plural word, so it takes _'s_ like every other singular noun. 2. /TAL-ess/ It's a Greek name. Initial _th_ is plosive, not soft, and the _a_ is flat, like in _flat._ The _e_ is also flat, as in _bet._ Amazing how you can rack up so many errors in just one word, but there ya go. I guess how they do teacherin' in Taxes. And you spend almost nine minutes wittering on about something that's fully explained by the diagram on the Wikipedia article. Just amazing.
Oh Sweet Jesus! Just shut up already.
I generally agree except "takes" in your comment sb "take's" - IDK what Grammarly say's, in this example "take's" isn't plural for take, it actuallt means "does take."
Measure the outside dimension from top to bottom & split the difference. finding the center of a circle is not rocket science.
Nice demonstration. I must deploy mathematics constantly in my daily work. (Customers seem to like things built well.) :)
As an applied mathematician I sometimes slum it by watching this type of video. Machinists have all sorts of hacks they use but could never prove in any rigorous way but they do work and are based on proper Euclidean geometry. A carpenter once asked me about a rule of thumb he used fo arches and I derived it from basic ellipse properties and it made sense. 17th century mathematics was highly geometric and Newton's Principia is almost unintelligible to modern readers. Indeed, Nobel Prize winning physicist Richard Feynman once tried to replicate Newton's highly geometric proof of his inverse square law of gravitation and it defeated him because Newton relied on obscure geometric properties that we simply don't learn these days because of analytic geometry etc. Newton did a geometric derivation of the shape of minimum resistance in a fluid and althougn it was obscure he got the same answer a fluid dynamicist would get woth modern techniques. Geometry is really powerful and only involves simple tools.
Draw two segments, segment ends touching the edge of the circle, shoot a 90 degree line from center of each segment, they'll cross at center of circle
OR, if you have access to a lathe, you can chuck this material up and use a center drill in the tail stock to find the center.
All this also comes from the Lord Almighty, wonderful in counsel and magnificent in wisdom. -Isaiah 28:29
The proof you gave for Thales' theorem only concerns the very special case of an isosceles triangle. Thales' theorem is much more general.
I’m aware.
Cool. I think this would be great to show to my geometry class. One pedantic note. I believe you are using the converse of Thales Theorem: if you have an inscribed right triangle in a circle, then the side opposite the right angle is a diameter. (In other words, Thales' theorem says "diameter implies right triangle" while you need "right triangle implies diameter.")
What brand of pencil
Mechanical Pencil 2mm amzn.to/3JMkitf Silver Streak Welders Pencil amzn.to/3Wox1tz
Amazing!
You make awesome videos dude.
I appreciate that!
Very interesting discussion of geometric principles. In your story: considering that the unfortunate structural engineer of record on this project actually thought that builders could construct the roof to within 4-mils of design elevation is both quite amusing and quite distressing at the same time! To construct it to within 1/2" of design would be impressive.
When you use a compass to draw the circle you already know where the center is.
Good stuff!
Draw any inscribed triangle in a circumference, then Trace the 3 mediatrixes. The point where they meet is the center of the circle! The prthocenter!!!
I think you mean orthocenter. And I have no idea what a mediatrix is.
Great story and totlaly believable Different attitudes in different professions. :-)
That's pretty cool 👍
Horrible green background color Very hard to see what your doing
Watching on my phone the pencil marks on the green paper are invisible. Might be different on a computer.
Or just use the set square to box the circle and draw the diagonals; where they cross is the centre.
"Ughh, this maths is boring! When will we ever use this stuff in real life?"
Thank you for the very direct route to explaining this! The paint story is so believeable in that I heard my father talking about how his days at nuclear sites- everything was so technically right yet painfully expensive when two surfaces did not meet because of such things.
Mr. THALES from Miletus was the first Philosopher ever. I could not imagine who told him this. He was the first ever. ❤
You just be a Millwright LOL great video
At 0:56 you could have used the endpoint of one of the existing two lines, to draw the third in a right angle, of which that third is parallel to the first. The diagram becomes simpler and easier to understand.
Thale’s theorem is just the inscribed angle theorem in reverse. The right angle used is an inscribed angle, and the inscribed angle theorem states that the angle AVB is equal to half the angle AOB, where O is the origin, and A, V, and B are points that lie on the circle. So given three points on the circle and the angle 2ø between two radii, you know the inscribed angle at the third point is equal to ø. But you can also go in reverse. Given an angle of 90° inscribed in the circle, the other two points form an angle of 180° with the center, so drawing a straight line between them must intersect the center. Then you just do that twice to find the intersection of the two diameters.
Yes. The most stunning practical demonstration of this is to draw a line AOB through the center of a circle. Then put a pencil on a third point, V, anywhere else on the circle. Even as you move that point V around the circle, the angle AVB remains exactly 90 degrees, always exactly half of AOB.
Even easier then that is take any ruler and hold one edge at 1 inch and diagonally angle the other side of the ruler at some other inch mark as well on the other side of the board. The center will be half the distance of that diagonal length... Example, start the diagonal line at one inch on one side of the board and say 5 inches on the other side. The center will be at the three inch mark of the ruler...
That technique can divide a board or panel into any number of marks. I learned it from old school sign painters. 👍
Cheers!
The other way I have heard this is that if you start with a semi-circle and draw a line from one end to any other point on the semi-circle and then from that point to the other end of the semi-circle the angle between those lines will allways be a right angle. You are using this property in reverse by putting the vertex of a known right angle on a circle. The intersection of the right angle with the circle will be a semi-circle which lets you draw a diameter line, which by definition goes through the center of the circle. Do this a second time to prodoce a sevond diameter line and the intersection of the two diameters is the center of the circle.
Stonemasons who built cathedrals and fortresses in the Middle Ages began by establishing the unit 1 by driving two studs into the rock or into a flat stone. A firm, level floor was then built around the site. Ruler, caliper, string, chain (rigid), spirit level was all they needed. Their knowledge of how to make circles, ellipses, 90 45 degrees from a line was their magic and secret. In a few places, the studs are saved for repair work. Saw a French documentary about this, if I find it again I will link it. I apologize for the language error, this is written with google translate.
I would be interested in seeing that documentary.
Love the story.
Bloody Yanks!! It's pronounced ''NEW-clee-er'' NOT ''NU-cu-ler''. Igroramuses....
What’s the matter, nobody stroking your bloated ego enough?
Very useful! Now are there 2 thales theorems?
Yes, the Proportionality Theorem.
There’s a few more theorems attributed to Thales as well, including the theorem that proves the base angles of an isosceles triangle are equal.
Here is another tip - how to create a right triangle when you have no rulers or levels or any equipment - only a long string and a knife. Use anything as a yardstick, and arm, a leg, a branch. Heck, stick two pegs in the ground, or use two rocks and define the distance as one unit length. Cut the string into three cords: 3, 4 and 5 units of length. Tie the ends of the cords together (3 to the 4, 4 to the 5 and 5 to the 3) and when you pull on the knots to the maximal extent, you got yourself a right triangle with the hypotenuse is the 5-units length cord. Of course, any multiple of the Pythagorean triplet would work: (6,8,10) , (9,12,15) etc. Pythagoras was a complete cultist loon, but a smart guy nonetheless.
Here's a handy add-on. To quickly get your cord lengths, take your string and anchor it on the corners of the 3-4-5 triangle you just layed out. Pull tight and mark at the corners. Cut at the marks and knot the ends. Now you're ready to lay out your triangle.
That is not Thales theorem You are using the central angle theorem
Yes it most certainly is.
@@txtradesman527 where are the parallel lines?
This isn’t the Proportionality Theorem.
@@txtradesman527 I know , it’s an application of the central angle theorem , twice .
It’s Thales’ Thereom, which is a special case of the Inscribed Angle Theorem.
Black pencil on green paper makes poor vision.
It never ceases to amaze me the things that people will complain about.
Set resolution to max. The compression makes it fuzzy, max resolution is clear.