Bandwidth - Gain & GBW
Вставка
- Опубліковано 9 лют 2025
- Learn more about the TI Precision Labs - Op Amp Evaluation Module used in the hands-on lab modules
www.ti.com/too...
This is the second of five videos in the TI Precision Labs - Op Amps
curriculum that addresses operational amplifier bandwidth. In this training,
we’ll discuss open and closed loop gain, gain bandwidth product, and
quiescent current vs. bandwidth. We will also simulate the bandwidth of a
circuit and show that it correlates to our calculated results.
Download the TINA-TI Spice Models for our video exercises
www.ti.com/lit...
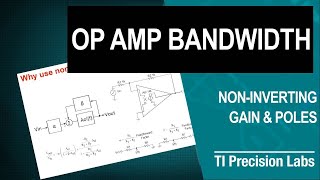







Hello, the Miller effect is missing in the formulas at the end of the presentation (Cc capacitor and the gain of the 2nd stage)
great explanation
Where did the closed loop gain equation (Acl = Aol / 1+AolB) come from? A little Kirchoff's law would be helpful here. Thanks!
Come on it’s pretty trivial
@@nothingtoseehere2189 That comment translates to "I am way smarter than you and have no time for you."
@@danmarquez3971 I didn’t mean it to sound like that but you’re right it does sound rude.
The output of the opamp I’ll call vout will be Vout=g*(vp-vm) where g is open loop gain vp is non inverting input and vm is inverting input. There is a voltage divider circuit created from Vout to ground with rf and r1 the voltage going into the non inverting input can be calculated using the voltage divider equation (this applies cause high input impedance of opamp) Vm=vout*r1/(rf+r1) letting B = r1/(rf+r1) gives vm=vout*B substituting our new value for vm into our original vout gives Vout = g(Vp-B*Vout) meaning Vout + g*B*Vout = g*Vp factoring out Vout and rearranging gives Vout = Vp*g/(1+g*B). V plus is just our input voltage and gain is defined as Vout divided by vin so if we just divide left and right side by vin we get Gcl= g/(1+g*B)
@@nothingtoseehere2189 Wow! I am impressed by your response! You were very diplomatic in the first sentence and you spent great time deriving the equations! I take back what I said! Thank you for the derivation! You rock! And sorry for the misunderstanding!
Where the books of subject,??
Thank you sir, I am from india.. this is very helpful for my studies! Thank you very much, Texas Instruments company❤️👍