Very Tricky Geometry Problem From Japan

GET MY EBOOKS
•••••••••••••••••••••••
150 Challenging Puzzles with Solutions : payhip.com/b/y...
Function : payhip.com/b/y...
Sequence and Series : payhip.com/b/B...
Differentiation : payhip.com/b/W...
Indefinite Integration : payhip.com/b/8...
Definite Integration + Area under the Curve : payhip.com/b/1...
Trigonometry : payhip.com/b/8...
OTHER CHAPTERS : COMING SOON.....
--------------------------------------------------------------------------------
Join the channel to become a member
/ @mathbooster

[@marioalb9726]

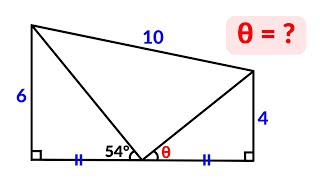
Triangle APD is similar to triangle CPD, both with internal acute angles 45° and θ.
Triangle PCQ is an isósceles triangle, with internal acute angles θ and θ.
Then, angle AQD is 2θ
Right triangle AQD,
θ + 2θ = 90° = 3θ
θ = 30° ( Solved √ )

[@lasalleman6792]

Assume magnitude of side of the square = 1 Set up line PC as base of an isoceles triangle,BPC . Both sides = 1. Give you a base such that PC =.765 Now, each base angle = 67.5 degrees. Then, set up a new isoceles triangle with .765 (PC) as the base. You have 22.5 degrees at each end of the new triangle, 135 degrees at apex Q . Works out to PQ, and QC each = .414. So, QC ,minus DC = Line DQ , which is = .586. Line DQ is sine of the angle needed. Line AD becomes the Cosine of the angle. Thus, going Pythagorean again. Sine/Cos = .586, resultant angle: 30.370. Call 30 degrees.

[@antoniopedrofalcaolopesmor6095]

Assume square side measures 1.
Let PQ = QC = a.
Triangles ABP and QDP are similar and therefore we have
DQ/PQ = AB/PA
(1-a)/a = 1/PA
PA = a/(1-a)
AQ = AP+a = a/(1-a)+a = (a+a-a^2)/(1-a) =
= (2a-a^2)/(1-a) = a(2-a)/(1-a)
Let's use Pythagorean theorem for triangle ADQ :
1^2+(1-a)^2 = a^2*(2-a)^2/(1-a)^2
Simplifying and solving this equation for a we obtain a = 1-1/sqrt3.
Let angle theta = x
tgx= DQ/AD = (1-a)/1 = 1/sqrt3 = sqrt3/3
Thus, x = arctg(sqrt3/3) = 30 degrees.

[@quigonkenny]

As ∠DAB = 90° and ∠DAQ = θ, QAB = 90°-θ. As AB and DC are parallel, ∠AQD is an alternate interior angle to ∠QAB and thus ∠AQD = ∠QAB = 90°-θ. As ∠CDA = ∠ABC = 90° and BD, as a diagonal of sauare ABCD, bisects ∠CDA and ∠ABC, ∠ABD = ∠DBC = ∠CDB = ∠BDA = 90°/2 = 45°.
Draw CP. As AB = BC, ∠ABP = ∠PBC = 45°, and BP is common, ∆ABP and ∆PBC are congruent, and thus CP = PA and ∠BCP = ∠PAB = 90°-θ. As ∠BCD = 90°, ∠PCQ = θ.
As CQ = QP, ∆CQP is an isosceles teiangle and ∠QPC = ∠PCQ = θ. As ∠PQD is an exterior angle to ∆CQP at Q, ∠PQD = ∠QPC + ∠PCQ.
∠PQD = ∠QPC + ∠PCQ
90° - θ = θ + θ
90° = θ + θ + θ = 3θ
θ = 90°/3 = 30°

[@Antony_V]

If we drop the perpendicular from P to the square's side AD and DC in points H and K we get two congruent triangles AHP and PKC. So angle PCK=θ as well as CPQ. Angle QPK=PAH =θ since AD and PK are parallel, thus 3θ=90°, θ=30°.

[@SGuerra]

Que questão bonita. Parabéns pela escolha.

[@RealQinnMalloryu4]

360°ABCDQ/2 180°ABCDQ 3^60 3^20 3^2^10 3^2^2^5 3^1^2^1 32 (ABCDQ ➖ 3ABCDQ+2).

[@marcelowanderleycorreia8876]

Linda questão!! 👌

[@harikatragadda]

Draw the diagonal AC with O as its midpoint.
∆AOP is Congruent ∆COP by SAS.
Hence ∠PAO = ∠PCO, implying ∠DAP= ∠DCP=θ
Hence, ∠PQD =2θ= 90-θ
θ=30°

[@giuseppemalaguti435]

PQ=a..l=lato del quadrato risultato le due equazioni..ltgθ+a=l e (teorema del seno su PCB) √2a^2(1+sinθ)/sin45=l/sin(90-θ/2)..sostituisco la a ,si semplifica l, risulta una equazione finale 2(1-tgθ)√(1+sinθ)=1/cos(θ/2)..θ=30

[@michaeldoerr5810]

The angle theta is 30°. That is the first time in a while we had a congruence postulate and a problem with the angle is NOT 15°.

[@arkojitsanyal3219]

Where do you get these problems?

[@errolflyne4630]

nice sir

[@professorrogeriocesar]

Ligue AC. Teorema do ângulo externo.
30.

[@me56743]

৩০

[@DhdhBdhx-m4z]

Sancs

[@Dinhnguyen-km6zd]

∠AQC =90+a. ∠CPQ=QCP=(90-a)/2