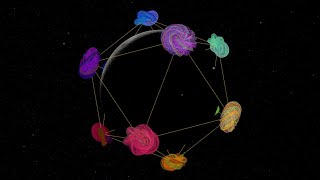
What is the Hopf Fibration?
Вставка
- Опубліковано 5 лют 2025
- In this video I shed some light on a heavily alluded to and poorly explained object, the Hopf Fibration. The Hopf Fibration commonly shows up in discussions surrounding gauge theories and fundamental physics, though its construction is not so mysterious.







Please keep making videos. Your content is fascinating and you are a great presenter.
The actual problem is that my screen is merely two-dimensional.
So r4 into 43 into r2
2 and 4 dimensions are like yesterday and tomorrow. 3rd dimension is now, and just like we imagine the 4th we imagine tomorrow. We create the 2nd dimension the same as we create pictures, videos and books about yesterday. No now no yesterday, no 3 no 2. Oh
@@painkey1189 No, the real problem is that my brain is one dimensional....
@@DaleClark1000 a 1 dimensional mind in a 3 dimensional world makes 4 dimensions.
You appear to maybe be the Tech Rules of interesting coding stuff. Subbed and looking forward to more.
Thank you for sharing this as it is helpful to clarify it's power and utility.
I would like to share a link to this video in the comment sections of other UA-cam videos where it is relevant but for some reason, one of the UA-cam algorithms has flagged me as a spammer, and blocks any comment I post if it contains a link.
Frustrating, as this video needs to be seen by more people.
People usually dont like links in comment section as its mostly either a Rick roll, self promotion or spam. So I dont think the algorithm did something its more likely that people reported those comments.
-Claim that the Hopf Fibration is the greatest thing ever
-Show cool picture
-Refuse to elaborate
-Leave
Lmao and there are also all these people in the comments who didn't understand anything but yet they say that is was amazingly interesting just because there were some cool words
Thanks for distilling this into an easy to digest clip! Hope to see more videos from you.
"Scientist" is a stretch, Weinstein's more of an intellectual grifter. If you can't explain something, you don't understand it properly, and/or you're just trying to baffle people with fancy word salad. But you explained this beautifully, great video!
I watched the Joe Rogan video just now. Weinstein seems to legitimately know what he's talking about. I think the problem is just that it's impossible to explain Gauge Theory at any meaningful level in 20 minutes to a non-mathematical audience.
@@BlueRaja Except this video did it in under 5 minutes! He knows enough gauge theory to sound clever but he has no interest in scientific method or education. He's a mathematician, not a scientist. He could have been a scientist but he used his mathematical prowess to get rich instead, and he was rude when he interviewed Roger Penrose, so fuck that guy lol
@@Lincoln_Bio Except that this video had the help of visual aids and already supposes that the viewer has at least a first year of uni level mathematics. Secondly, Weinstein is a mathematician because he researches mathematics and not science. However, neither scientists nor mathematicians are automatically educators. Learning mathematics is NOT the same as educating mathematics. University researchers are not "intellectual grifters" just because they can not explain a topic that builds upon years of prior knowledge just because a random viewer like yourself got interested in a cool sounding word for 20 minutes.
@@AD-ox4ng Good push back. I don't know why these toxic twerps can't just ignore _Geometric Unity_ and its creator. They clearly aren't interested. They must just be intellectual poseurs hoping someone else tears down Dr Eric Weinstein's work so they have adequate excuse to not read the paper. I have read the paper. I understand it. I am an art school drop out with no education in these advanced branches of _General Relativity_ or _Quantum Field Theory_ or Partial Differential Equations, or Special Orthogonal groups.
I had to look all that stuff up and it took me two years to puzzle out the gist of what was going on in _Geometric Unity_ and I doubt I would have had the time were it not for the pandemic lockdowns legally prohibiting me from leaving my house more than once a day, for exercise.
He's for real as someone with a mathematics background. What you experience is something similar to thinking French people are dumb because they're speaking gibberish to eachother.
You explained the math but not what the Hopf Fibration is or why it is relevant.
i think is clear in the begining " i gonna explain precisly what it is" it not ulgarisation i think , but he would surely gain from doing so like 3blue 1 brow
Same thought, I was disappointed the video ended before exploring potential applications
The hopf fibration may pop up in some GUT theory related to division algebras (as far as my knowledge extends to), essentially, some part of spaces were turned into ‘fibre’ where you don’t see in the new space
This was a very clear explanation. Thank you.
Keep making awesome videos, I love it
Hey dude really liked your Minecraft video, if you made another one I would watch it.
Tip: Since your video content is highly dependent on the correct way of saying things I highly recommend a tool like Descript for text-like editing of your voiceovers. I think Adobe Audition has something similar, not sure. Nonetheless: great content!
Alice took both pills simultaneously and became a Hopf fibration (Twisted Alice).Remember what the Hopf fibration said - “Feed your head”
Underrated channel
About that Minecraft AI creation videos you made a few months back, maybe try some more of those?
Finally I got some sense of this concept
Very pretty.
Here is what I believe is the fundamental shape of the universe. A minimal single sided closed surface. The central node representing the event horizon or Janus point or neutron. When energy density is at maximal. The flow into this point is gravity and the inverse side is neutron decay. The packaging and unpacking of hydrogen being the fundamental process of the universe.
Surface(cos(u/2)cos(v/2),cos(u/2)sin(v/2),sin(u)/2),u,0,2π,v,0,4π.
Notice that 4pi, 2 full rotations are needed to complete the surface. Electron half spin is an artifact of this topology.
Neutron Decay Cosmology.
The path of least action, topological, physical process solution to black hole paradoxes, dark energy, dark matter and critical density maintenance.
Interesting stuff👍🏻
I do experiments sometimes would it be possible to get your opinion on a video I took of some real world unusual topology in light.
Looks 4 dimensional with some inverse action.
@@ORBITALAURORA drop a link to it
@@KaliFissure Got images of Dark information Energy as-well from the Holographic Multiverse if you are bored 🫡
ua-cam.com/video/-75hSWs3Fl0/v-deo.html
@@KaliFissure I just do experiments with electro magnetism that I find interesting.
Coming to al sorts of conclusions🖖
My new polarising multi layered nanoparticle magnetic viewing lens👉🏻on the front of my go pro and encased within a small custom faraday cage on the way to the north face 🍿🍿🍿
For entertainment purposes only🫡
ua-cam.com/video/flaG7yi5GW8/v-deo.html
This is excellently explained
We need to go back to r² and the three-dimensional physics of the Inverse Square Law. The spherical 4πr² geometry is key to this concept, based on Huygens' Principle from 1670, which states, "Every point on a wave front of light has the potential to create a new spherical 4πr² light wave."
Each point can be considered a potential photon ∆E=hf electron interaction exchanging potential photon energy into the kinetic Eₖ=½mv² energy of matter in the form of electrons e².
We experienced this as a continuously emerging probabilistic future with the spherical 4πr² surface acting as a boundary condition or manifold for the uncertainty ∆x∆pᵪ≥h/4π encountered in daily life.
This reminds me of 3b1b great work!
So if I get what you're saying, what we're seeing on screen is actually the even more simplified representation of those points on the 2-Sphere then mapped onto the same points on the 1-Sphere as it were.
When will you upload again?
It's the symmetry of the strong force radiating outward into the nuclear force which decays into the weak force and through it into electromagnetic polarity. This is how you go from tri-polarity and higher dimensional tiny space (subspace manifolds?) into binary polarity into the three dimensional space that you are familiar with. Location means different things in different frames of these concepts. This is the fundamental shape of space itself. This is the fundamental shape of diffusion of energy. Space is a consequence of diffusion of energy. Time is a consequence of diffusion of space.
Great video. Thanks. Does it exist or is it just an abstract math concepts?
And I still don’t understand why it’s so important?
Wheres episode 2.
This was a clear exposition about the projection of an imaginary object consisting of elements created by unknown virtual reasons existing in a virtual dimension explained to 70% in abstract languages unknown to most but called topology, mathematics, holography & academic "Hold my Smoothie" gibberish...
Dang. I was looking for the "????" math for some custom visualizations. Possibly looking at fractals/mandelbrot from z1 and z2 components. Is there anything useful on the web for the "????"
Thanks for explaining
Loved the video! can't wait to see this in my reomended in 5 years tho lol
Ikr?!
Conversely, you could do this with XYZW(R4) to (XYZ)(R3) - I specify this merely because I dislike using time as a fourth dimension, because it technically isn’t. Wonderful video though
very interesting. i have a question with regard to the degrees of freedom: if we have the constraint that |z1|^2 + |z2|^2 = 1 in the input, doesn't this take away one degree of freedom such that the input has actually only 3 instead of 4 degrees of freedom? likewise, if in the output we have this constraint shown at 3:16, that the output has actually only 2 degrees of freedom instead of 3?
Yes. The Spheres are embedded in larger spaces, but because we are dealing with surfaces rather than volumes we lose a degree of freedom and a dimension. It is still easiest to talk about them as a surface in the larger space because the topology is distinct from Euclidian space, so we can not uniquely represent the whole space as n values where n is the dimensionality of the space. If we try to unwrap a sphere onto a plane you should see that you will always get some places where you have to cut it, and that can make things harder to work with.
The "look at that cool picture" version is still simplier.
;)
Well explained
Are you the guy that explained physics on a video as a kid? You sound alike. Great video btw
Great Explanation thank you!
Great video 👍🏻
Thank you! This helped a lot.
Very good.
At 3:15 the third row should be different, factoring out the squared magnitude terms and using (4-2)=2 as a factor, right?
Where are you man?. superb presentation...like a teaser!! please bring the real meat...!!
Thanks a lot, great video
Anyone know on which software was that picture made'?
Fun video. I always found this stuff interesting. 4 dimensional shapes are fun to think about. Idk about the scientist part but ill stay informed. ^^
This is great!
Ok, non-physicist here. I’ve watched several of Weinstein videos and listened to several on the “the Portal”. My take, Weinstein is really “fighting” with the String Theory people. Back to Hope Fibration. So knowing all of the formulas “map” correctly between 4 and 3 dimensions, this appears to makes Mathematicians sleep better at night. My question is by knowing about the HF, does it change anything in the world? Are people smarter and better off knowing about the HF? Or is it really just some kind of “inside baseball” academic exercise?
OK, now I understand that the Hopf fibration maps from a space with 4 degrees of freedom to a space with 3 degrees of freedom and in doing so it always maps a fiber (1 degree of freedom) onto a single point (0 degrees of freedom). You know which other function does that?
f(a, b, c, d) = (a, b, c)
A pretty boring function, right? So what's so special about the Hopf fibration?
Maybe because it uses imaginary values and geometric principles to explain that function? I could be way off on this though, I'm not nearly a mathematics major.
@@TejasIsAmazing f(a, b, c, d) = (a, b, sqrt(c² + d²)), so what?
It doesn't just ignore one coordinate and as a bonus it even maps circles onto points.
I still don't get the hype.
@@clumsyjester459 Except that it doesn't fill the whole sphere. How do you get the (0,0,-1) point?
Yea this video is very bad for this reason, it just says "ugh cool formula cool picture cool words". The actual interest of Hopf fibration lies in algebraic topology where it allows to compute some homology groups on the spheres (homology groups are kinda like the ways of triangulating a topological space)
You explained how it’s mapped, but not why the hopf sphere is significant or matters… I gained nothing new from this video than I gained from the other videos you teased in the opening.
Find it hard to understand, but like it, will try later 🙏
I have vibration is just a rotation rotated by a rotation rotated by a rotation and then showing the resulting point. Rotation vectors in three coordinates XYZ are really where this takes place. There isn't really anything four-dimensional about it. This was a very simple demo to throw together once I actually got the rotations working. And a rotation is just axis angle.,. Which the angle is just the length of the 3D vector and the axis is the direction of the vector.
It's not four dimensional anymore than representing a velocity as the three-dimensional vector and then decomposing that into speed and direction makes the velocity of four-dimensional vector
good video
I like your funni words majjizk man
where are u ?
The equation isn't very enlightening. I'd like to see some annotations. If I recall correctly, the fibers are torii when extended to R^4. It would be interesting to see the visual intuition for this.
Nice video 👍
Are there more dimensions then 4? Also wouldn’t the fibers be as thick as a plank?
Either way this is above my capacity but yay for science so have a comment.
You probably need a correction on that magic algebra at 3:15. The second and the third line are the same.
So as you can see @ 2:17 this is why they show us the col picture and then we just have to go with it... 🤷♂
Great video if you're already into math and physics lol, but this is just hieroglyphics to most people. It takes 10 minutes just to sort of make this make sense to someone else, even using math or a moving image, broken down. I feel like adding a 2 dimensional representation of the values on a graph and how it translates into 3d and then showing how it's not 4d, is extremely important to explain before even delving into the rest of the math behind this..... quantum "God."
sphere with a sphere at every point? shouldnt this be sth 4-dim?
Lmao this is literally just the transformation from a a spinor to a Bloch vector in spin half
But what does it tell us? Z1, z2 what are they?
They are two complex numbers whose 4 real constituents can be used as coordinates for the 3-sphere.
The unit 3-sphere is then described by
|z1|**2 + |z2|**2=1.
But so-called Clifford tori can also be described, and yet as a family generating the very 3-sphere:
|z1|**2=0.5+s and
|z2|**2=0.5-s
describes a Clifford torus (a 4D surface) for each s between -1/2 and +1/2, and the whole family, over the whole range of s, generates the whole 3-sphere (a 3D manifold "filled" by these tori).
Keeping z1 constant and letting |z2|**2=0.5-s, describes one circle of some Clifford torus: a Villarceau circle. Doing this for all valid values of z1 generates all Clifford tori and thus the whole 3-sphere. (By switching roles of z1 and z2 a twin family of circles is obtained).
Villarceau circles don't intersect each other. That's what is meant by them constituing a "fibration", like a set of "fibres" generating a wire, or a strand of wires, or a compound of strands, or... Clifford tori and their 3-sphere.
See my video "Wugi's 4D world- The 3-sphere and its bestiary- Part 3: the Hopf fibration":
ua-cam.com/video/5rdYjgqUoDM/v-deo.html
perhaps after watching parts 1 (Clifford torus) and 2 (stereographic projection and Dupin cyclides) on my channel.
I'm picking up Hopf Fibrations
Ngl this guy's voice just sounds smart 🤔
True!!!!
thank you ☺️
you deserve more like comment(and especially subs) than this.
Plsss upload more vids :)
A single static charge is Q.G..to avoid its own collapse it has to non causally exist as a kinetic energy eternal “ER-ER” bride. This avoids singularity & infinity Static charge is its own C.P. inverse. A 3-sphere.(Spinor)Geodesic 90Gly/2Pi*pt. The speed of light is Path of least action between these symmetries.(between 2 stereographic projection(cos^2) . Solve the static charge problem in G.R..This is why we see so much duality ,we are causality of duality. Mass without Mass.
Word salad. I diagnose you with crank.
Einstein’s biggest blunder was to make ‘C’ a postulate,we need a relativistic equation to make ‘C’ invariant..The speed of causality is ‘C’ ,the path of least action in this duality. You need a theory for mass without mass.
By saying a static charge is an oscillation of its own CP inverse you have a Superfluid theory.(CPT).
Stop spamming this on unrelated videos.
cringepost
The speed of light being c isn’t a postulate though. We have literally experimentally observed it to be the case that all observers see the speed of light to be equal to c.
The rest of your writing is completely illegible since you use a bunch of jargon like “CP inverse” and “Superfluid theory”. I have no idea what your goal is here, but if it is to convince others you really need to improve your writing.
@@kikivoorburg I agree with you that the rest of their comment is nonsense, but, I don’t think something being supported by the evidence makes it not a postulate/axiom ? It’s just, one which is justified. Special relativity is derived from the axiom that c is the same in all reference frames, and this axiom was chosen because of the experimental evidence for it?
Yeah... I still don't know what the hopf fibration is.
Neither do I. I'm speculating it may be the points where the spheres that make up the surface of a 3-sphere intersect the 3D sphere, but I honestly have no idea what the actual answer is
The equation seems suspiciously simmilar to the one that finda pythagoric triples
This is the shape of the *Universe* . It makes sense when you think about dimensions and a multiverse.
Could you explain why it looks like a torus.
yeah
excuse me sir. We need more videos from you.
This is cool and all but why is this always in my recommendations
0:03 A Eric Wienstien, definitely “scientist” dude! lol
Good 👍
my brain just melted
Cool video. Didn't understand a thing because you were explaining variables so maybe just do it without math
Bravo.
A Sphere projected on a hyper sphere projected on a 2 dimensional surface
Good video, but why the hate for Weinstein?
0:17 shows typically human thoughts about this video.
is this guy a dead-tuber
So not magic, but math.
But, science that is not understood, is magic.
So, magic.
Nice
👍👏
Now do spinors
Is anyone else getting black hole vibes from these visualizations?
HOPF FIBRATION 🤔
4 dimension don't really exist. If you want to call complex detail of a shape or object having extra dimensions you can. You have length, width and height three dimensions. You have the dimensions of your face, you wouldn't call the nose on your face a 4th dimension.
Indeed, but mathematically they do and are used often to calculate real-world things
Okay, watching videos of the backrooms somehow lead me here... x'D
yeah i didnt understand any of that lol
Imagine thinking you're smarter than Weistein.... Curb that ego. It's explained the way it is because it's hardly easy to conceptualise for a lay person.
So why is this even remotely useful?
??????
So why is it such a profound object? It just seems like a fun math exercise. How does it teach scientists anything importantt about our natural world?
Look please very nice animation of big magic squares on torus for big numbers - such homepage under number-galaxy eu.
Weinstein is pretty nervous about this polygon...
Is this a possible pocket dimension? 🧃
just found a cool guy on the internet @Poppro