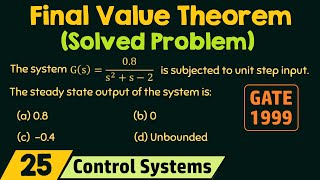
Initial Value and Final Value Theorems

Control Systems: Initial Value and Final Value Theorems
Topics discussed:
1. Initial and final values of a signal (function).
2. Significance of Initial and Final Value Theorems.
3. Initial Value Theorem.
4. Example based on the Initial Value Theorem.
5. Conditions of Initial Value Theorem.
6. Final Value Theorem.
7. Example based on the Final Value Theorem.
8. Conditions of Final Value Theorem.
9. Examples of Unbounded and Oscillatory Functions where the Final Value Theorem is not applicable.
Follow Neso Academy on Instagram: @nesoacademy(bit.ly/2XP63OE)
Contribute: www.nesoacademy...
Memberships: bit.ly/2U7YSPI
Books: www.nesoacademy...
Website ► www.nesoacademy...
Forum ► forum.nesoacad...
Facebook ► goo.gl/Nt0PmB
Twitter ► / nesoacademy
Music:
Axol x Alex Skrindo - You [NCS Release]
#ControlSystemByNeso #ControlSystems #InitalValueTheorem #FinalValueTheorem

[@aaronfritz6892]

Seriously, its so weird having a college professor jump straight into how to derive these concepts without explaining the concept in terms of what its used for or how it relates to other things. These videos are what fills the gap for me, thanks for making this intuitive for a beginner!

[@sanjayprabhu6909]

hello neso i am really happy you are uploading vedios continously thankyou very much

[@PunmasterSTP]

Initial value? More like "Incredible; thank you!" These videos really are quite splendid.

[@maharjaveed8289]

Good method and easy to understand
Again your's work is very Good

[@balasubramaniyans1426]

Excellent explanations sir

[@rahulguptha3131]

Nice video thank you we need more videos like this

[@ippalavalasaanusha3672]

Thanks a lot neso academy for the great explanation

[@mohammeduzair1276]

I can't believe the fact that such amazing videos have such few likes!

[@sohelansari-dq5kv]

great teacher

[@gowriv944]

Nice sir.upload more and more vedios about control system.

[@NitinSharma-so9hg]

Good Lecture . THANKYOU

[@patriciaa4260]

Thank you so much! You have helped me!

[@sarojinivaradharajan5598]

Great! Thank you very much

[@Happiestsoul31]

Thank you

[@s.m.senses7199]

thanks Sir🙏❤

[@aaqibrashidle6655]

Uh r great sir

[@hikmathasanov]

For the first condition, I think it is supposed to be s.F(s). That is how it was explained before at Signals and Systems Lecture Series.

[@kaverik.thekodda]

Is the value of u(0) & u(inf.) just equal to "1"?
See 01:50
I ask because, we determine the value of the function using only the term with the exponent.
(or that's how it seems)

[@kaverik.thekodda]

does the question makes sense?

[@comment8767]

u(t) looks like it might be a step function .... is is not just anything. So is does equal 1 at times later than zero.

[@vectorrehan9340]

thanks a lot

[@harshitpandey8696]

❤️❤️❤️😭😭😭tysm

[@pawandabad5263]

Nice sir

[@sudeepshetty4530]

In final value theorem 2nd example, how do you say F(s)=1/(s-2) pole value is 2? No clarity given about direct conclusion of RHS value?? Can you explain more..other than that quality of explanation is super.

[@PunmasterSTP]

A pole is a value of the variable that makes the denominator of a function equal to zero. Because the factor (s - 2) is in the denominator of F(s), we can set s - 2 = 0 to see that s = 2 is a pole.

[@agjhv3835]

5:38 but the example you took is discontinuous at t =0

[@PunmasterSTP]

Yeah I was a bit unsure of that part as well. I think the main idea is that the function can't be discontinuous immediately after t = 0, as in, f(0+) is finite and the limit of f as t approaches 0 from the right is f(0+).

[@triposat]

Please upload the lectures of Data Structure as soon as possible. please sir 🙏🙏

[@nesoacademy]

Sure 👍

[@vinayaaaaaaa]

Sir please upload transient analysis🙏😭

[@chidanandadatta4695]

Can we say, result from final value theorem as dc value of the system??

[@serenasavage9842]

For final value theorem, how does 1/s+2 = 0 if s=0? I don’t understand what I’m missing

[@comment8767]

The value of u(0) in Example 1 is unclear. If u(0) = infinity, then IV would be infinity, no?
Maybe u(t) is a step function at 0? It is not a generalized function?

[@-DanishShafi]

There is a mistake in the initial value in the first example.
It's answer is 0 not 1

[@shreyashagrawal2264]

If we do Inverse Laplace Transform of F(s) = 1/(s+2) then it will give us f(t) = e^-2t and therefore f(t) violates the condition 1 i.e. f(t) = 0, t

[@ahasnainkhan9622]

f(t)=e^-2t.u(t) for F(s)=1/(s+1) not just e^-2t, hope answered your question.

[@akshaymatsagar1710]

sir please complete the paylist .............we r waiting for further lectres

[@akshaymatsagar1710]

playlist

[@Muvva_raj]

In first example e power zero is one ....but side of that we have u(0) whole will become zero no?

[@mohamedabdulraseethma9568]

u(t) is an unit step function whose value at t=0 is equal to 1.

[@shraddhajalwani5481]

sir please upload the power system video 🙏🙏🙏🙏❤
you have uploaded 26 videos only please complete it ,

[@sushrutmdhakate9874]

If final value theorem is not apllicable , then in all cases is that unbounded ?

[@javaandroid6964]

Explain more

[@albertdot5704]

If system is marginal stable, will final value theorem applicable,??

[@syedismail1849]

🔥🔥🔥🔥🔥

[@aryanpatil4718]

Thank you so much😊

[@dungaajay6085]

u(t) is not defined at 0 right..then how e^0.u(0) = 1?please explain

[@johnaime213]

that is why they use 0+, meaning slightly after 0.

[@talhafaraz6510]

If any one have the lab report on this topic please share with me as soon as possible

[@GatreddiMohith]

😂

[@waytowardspeace-fv9mx]

Make video fast...

[@rahulguptha3131]

Nice video thank you we need more videos like this