Proof: Summation of PMF of Negative Binomial Distribution is equal to 1 (One) in English

This video shows how to prove that the Summation of the Probability Mass Function (PMF) of Negative Binomial Distribution is equal to 1 (One) in English.
Check out Proof of Newton's Binomial Theorem: • Proof of Newton's Bino...
Just minor correction:
(-r)! = (-r)(-r-1)(-r-2) .... down to negative infinity not 1. As well as (-r-x)! = (-r-x)(-r-x-1)(-r-x-2) ... down to negative infinity not 1. They will cancel out as numerator and denominator so the results should be the same. Sorry for the confusion.

[@Sairaj922]

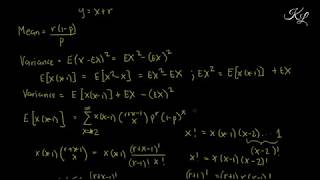
6:16 , sir i have a doubt. If i subtract 1 from a negative number again and again we will not get 0. Here r is positive so -r is negative. I am confused.

[@computationempire8603]

Your doubt is reasonable. Factorial of a negative number will be extended until negative infinity not 1. But the results will still be correct since the terms in numerator and denominators will still cancel out as they approach negative infinity. I will update this video soon. Thanks for your comment.

[@하핳하핳핳-l1v]

Wow it's really easy to understand! thank you sir!

[@rymanak9811]

Hi , isn't (Z+1)^m equal to a summation with upper bound M not infinity ??

[@computationempire8603]

It can be bounded to M or infinity, the result should be the same. This is because as i is from m+1 to infinity the terms will become zero. For example, m chooses m + 1 is zero since m + 1 is greater than m. It results in a denominator with a (-1)! which is negative infinity. Then dividing with this negative infinity will results to zero. You can find in various sources newton's binomial theorem which uses infinity or M as its upper bound.

[@rymanak9811]

Computation Empire Thanks a lot for your reply 👍

[@anowarali668]

@@computationempire8603 Thanks for the clearing. but factorial of negative numbers is not defined, right?

[@thandoemmanuel8863]

Very very very confusing,