Algorithm of Basic Concepts
Algorithm of Basic Concepts
  • 92
  • 180 631
Evaluate 𝐼=∫ᵧ 𝑥𝑑𝑧, where ᵧ be the boundary of square [0,1]×[0,1] with 𝐶 considered as 𝑅².
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍
𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:-
Evaluate 𝐼=∫ᵧ 𝑥𝑑𝑧, where ᵧ be the boundary of square [0,1]×[0,1] with 𝐶 considered as 𝑅².
𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:-
Coming Up
------------------------------------------------------------------
Solved...!!!
.
.
#complexanalysis #csirnet #complexintegration #riemannintegral
.
🔥🔥🔥Hurry Up Students for whole detailed Videos with Answer in Description for making notes 🔥🔥🔥
🔥🔥🔥JOIN JOIN JOIN JOIN 🔥🔥🔥
👉 www.youtube.com/@abcstudy
🔥🔥🔥All B.Sc., B.Tech , M.Sc. , M.A. Mathematics students join with us A great platform to get knowledge in Mathematics and science... Stay tuned....for detailed videos on whole SYLLABUS with topic wise...and also join us for CSIR-UGC National Eligibility Test (NET) for Junior Research Fellowship and Lecturer-ship COMMON SYLLABUS FOR PART 'B' AND 'C' MATHEMATICAL SCIENCE 🔥🔥🔥
------------------------------------------------------------------
Channel Link: 🔥🔥🔥
www.youtube.com/@abcstudy
Videos links 🔥🔥🔥
👇👇👇👇
------------------------------------------------------------------
88) ua-cam.com/video/suJmSKIFzic/v-deo.html
87) ua-cam.com/video/bo89kRz_Jj0/v-deo.html
86) ua-cam.com/video/O22npFevnbk/v-deo.html
85) ua-cam.com/video/8byayoA8__4/v-deo.html
84) ua-cam.com/video/EFLU7oxAgGE/v-deo.html
83) ua-cam.com/video/mIERxZKjNTU/v-deo.html
82) ua-cam.com/video/7rXn7tFaIE4/v-deo.html
80) ua-cam.com/video/0zGfyo-Vr8E/v-deo.html
79) ua-cam.com/video/nXpusovwZHE/v-deo.html
78) ua-cam.com/video/IW2jaYuoiRo/v-deo.html
77) ua-cam.com/video/gKcsLtG1X5I/v-deo.html
76) ua-cam.com/video/Cgm0X8GVP2o/v-deo.html
75) ua-cam.com/video/3Hz88WtbXPM/v-deo.html
74) ua-cam.com/video/bJm_HjsL1DQ/v-deo.html
73) ua-cam.com/video/C-C4wja_RME/v-deo.html
72 ) ua-cam.com/video/ZVEaVZVe8YM/v-deo.html
71) ua-cam.com/video/abCnc2oc9JY/v-deo.html
70 ) ua-cam.com/video/xmuCsUoz2-c/v-deo.html
69) ua-cam.com/video/QEf1wYMs5zs/v-deo.html
68) ua-cam.com/video/uFkobW2Q830/v-deo.html
67) ua-cam.com/video/hknYpQcKZHY/v-deo.html
̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅ ̅
Play List ( Complex Analysis )
👉 ua-cam.com/play/PL2b8PWU6ZN162yLzO0Qs0Eq_djb1dX83v.html
------------------------------------------------------------------
------------------------------------------------------------------
Kindly join Telegram channel 🔥🔥🔥
👉 t.me/abcstudyy
-------------------------------------------------------------
Переглядів: 308

Відео

Find ∫ ᤲ ᷝͥͥͥ ໋ ͥ(𝑧²+𝑧)𝑑𝑧 by choosing two different paths of integration & show that result are same15:16Find ∫ ᤲ ᷝͥͥͥ ໋ ͥ(𝑧²+𝑧)𝑑𝑧 by choosing two different paths of integration & show that result are same
Find ∫ ᤲ ᷝͥͥͥ ໋ ͥ(𝑧²+𝑧)𝑑𝑧 by choosing two different paths of integration & show that result are same
Переглядів 535Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Find ∫ ᤲ ᷝͥͥͥ ໋ ͥ(𝑧² 𝑧)𝑑𝑧 by choosing two different paths of integration & show that result are same 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- Coming Up Solved...!!! . . #complexanalysis #csirnet #complexintegration #riemannintegral . 🔥🔥🔥Hurry Up Students for whole detailed Videos with Answer in Description for making notes 🔥🔥🔥 🔥🔥🔥JOIN JOIN JOIN JOIN 🔥🔥🔥 👉 www.youtube...
Evaluate the integral ∫𑀾 𝑧̅ 𝑑𝑧 where 𝐶 is the straight line from the point (1,0) to the point (1,1)3:34Evaluate the integral ∫𑀾 𝑧̅ 𝑑𝑧 where 𝐶 is the straight line from the point (1,0) to the point (1,1)
Evaluate the integral ∫𑀾 𝑧̅ 𝑑𝑧 where 𝐶 is the straight line from the point (1,0) to the point (1,1)
Переглядів 3,4 тис.Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Evaluate the integral ∫𑀾 𝑧̅ 𝑑𝑧 , where 𝐶 is the straight line from the point (1,0) to the point (1,1) . 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- We know that 𝑧 = 𝑥 𝑖𝑦 ∴ 𝑑𝑧=𝑑𝑥 𝑖𝑑𝑦 ⇒𝑑𝑧=𝑖𝑑𝑦 (∵𝑥=1⇒𝑑𝑥=0) and 𝑧̅ = 𝑥 - 𝑖𝑦 Here line 𝐴𝐵 , 𝑥=1 and 𝑦 moves 0 to 1 . ∫𑀾 𝑧̅ 𝑑𝑧 = ∫ ᤲ ᷝ(1-𝑖𝑦)𝑖𝑑𝑦 ⇒∫𑀾 𝑧̅ 𝑑𝑧 = 𝑖∫ ᤲ ᷝ(1-𝑖𝑦)𝑑𝑦 ⇒∫𑀾 𝑧̅ 𝑑𝑧 = 𝑖[ 𝑦] ᤲ ᷝ [ 𝑦²/2] ᤲᤲᤲ ᷝ ⇒∫𑀾 𝑧̅ 𝑑𝑧 = 𝑖[1-0] [(1/2)...
Evaluate ∫𑀾 𝑧̅ 𝑑𝑧 , from 𝑧 = 0 to 𝑧 = 4+2𝑖 along the curve 𝐶 given by 𝑧 = 𝑡² + 𝑖𝑡 .4:32Evaluate ∫𑀾 𝑧̅ 𝑑𝑧 , from 𝑧 = 0 to 𝑧 = 4+2𝑖 along the curve 𝐶 given by 𝑧 = 𝑡² + 𝑖𝑡 .
Evaluate ∫𑀾 𝑧̅ 𝑑𝑧 , from 𝑧 = 0 to 𝑧 = 4+2𝑖 along the curve 𝐶 given by 𝑧 = 𝑡² + 𝑖𝑡 .
Переглядів 4,8 тис.Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Evaluate ∫𑀾 𝑧̅ 𝑑𝑧 , from 𝑧 = 0 to 𝑧 = 4 2 along the curve 𝐶 given by 𝑧 = 𝑡² 𝑖𝑡 . 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- Given that the curve 𝑧 = 𝑡² 𝑖𝑡 ⇒ 𝑥 𝑖𝑦 = 𝑡² 𝑖𝑡 ⇒ 𝑥 = 𝑡² and 𝑦 = 𝑡 ⇒ 𝑥 = 𝑦² this is the equation of parabola for the given parametric equation 𝑧 = 𝑡² 𝑖𝑡 ∴ 𝑧 → 0 ⇒ 𝑡 → 0 and 𝑧 → 4 2 ⇒ 𝑡 → 2 and we know that 𝑧 = 𝑡² 𝑖𝑡 ∴ 𝑑𝑧=(2𝑡 𝑖)𝑑𝑡 and 𝑧̅ = 𝑡² - 𝑖𝑡 No...
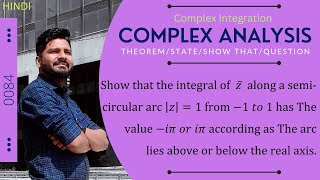
Prove ∫𑀾 𝑧̅𝑑𝑧 along semi-circular arc of |𝑧|=1 from -1→1 is -𝑖𝝅/𝑖𝝅 according above/below real axis.5:40Prove ∫𑀾 𝑧̅𝑑𝑧 along semi-circular arc of |𝑧|=1 from -1→1 is -𝑖𝝅/𝑖𝝅 according above/below real axis.
Prove ∫𑀾 𝑧̅𝑑𝑧 along semi-circular arc of |𝑧|=1 from -1→1 is -𝑖𝝅/𝑖𝝅 according above/below real axis.
Переглядів 483Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Prove that the value of integral of 𝑧̅ along a semi-circular arc of |𝑧|=1 from -1 to 1 is -𝑖𝝅 or 𝑖𝝅 accorded as the arc lies above or below the real axis. 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- We have |𝑧|=1 ⇒𝑧= 𝑒ⁱᶿ & 0≤𝜽≤2𝝅 And 𝑧̅=𝑒⁻ⁱᶿ and also 𝑑𝑧 = 𝑖𝑒ⁱᶿ𝑑𝜽 Here 𝑧 moves from along the semi-circular arc above the real axis is 𝑐₁ 𝜽 → 𝝅 to 0 ∫𑀾 ₁ 𝑧̅𝑑𝑧 = ∫ ᮣⷪ𝑒⁻ⁱᶿ𝑖𝑒ⁱᶿ𝑑𝜽...
Prove ∫𑀾 𝑑𝑧/𝑧 along semi-circular arc of |𝑧|=1 from -1→1 is -𝑖𝝅/𝑖𝝅 according above/below real axis.5:47Prove ∫𑀾 𝑑𝑧/𝑧 along semi-circular arc of |𝑧|=1 from -1→1 is -𝑖𝝅/𝑖𝝅 according above/below real axis.
Prove ∫𑀾 𝑑𝑧/𝑧 along semi-circular arc of |𝑧|=1 from -1→1 is -𝑖𝝅/𝑖𝝅 according above/below real axis.
Переглядів 573Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Prove that the value of integral of (1/𝑧) along a semi-circular arc of |𝑧|=1 from -1 to 1 is -𝑖𝝅 or 𝑖𝝅 according as the arc lies above or below the real axis. 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- We have |𝑧|=1 ⇒𝑧= 𝑒ⁱᶿ & 0≤𝜽≤2𝝅 And 1/𝑧=𝑒⁻ⁱᶿ and also 𝑑𝑧 = 𝑖𝑒ⁱᶿ𝑑𝜽 Here 𝑧 moves from along the semi-circular arc above the real axis is 𝑐₁ 𝜽 → 𝝅 to 0 ∫𑀾 ₁ (1/𝑧)𝑑𝑧 = ∫ ᮣⷪ𝑒⁻...
Evaluate the integral 𝐼=∫𑀾 𝑅𝑒𝑧 𝑑𝑧 , on the circle |𝑧|=𝑟 .5:38Evaluate the integral 𝐼=∫𑀾 𝑅𝑒𝑧 𝑑𝑧 , on the circle |𝑧|=𝑟 .
Evaluate the integral 𝐼=∫𑀾 𝑅𝑒𝑧 𝑑𝑧 , on the circle |𝑧|=𝑟 .
Переглядів 1,7 тис.Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Evaluate the integral 𝐼=∫𑀾 𝑅𝑒𝑧 𝑑𝑧 , on the circle |𝑧|=𝑟 . 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- We know that 𝑅𝑒𝑧 = ( 𝑧 𝑧̅ )/2 ∵ 𝑧 = 𝑟𝑒ⁱᶿ and 𝑧̅ = 𝑟𝑒⁻ⁱᶿ ∴𝑅𝑒𝑧 = 𝑟(𝑒ⁱᶿ 𝑒⁻ⁱᶿ)/2 Now 𝑧 = 𝑟𝑒ⁱᶿ⇒ 𝑑𝑧 = 𝑖𝑟𝑒ⁱᶿ𝑑𝜽 We have the integration 𝐼=∫𑀾 𝑅𝑒𝑧 𝑑𝑧 ⇒ 𝐼=∫𑀾 {𝑟(𝑒ⁱᶿ 𝑒⁻ⁱᶿ)/2}𝑖𝑟𝑒ⁱᶿ𝑑𝜽 ⇒2𝐼=𝑖𝑟²∫𑀾 𝑒ⁱᶿ(𝑒ⁱᶿ 𝑒⁻ⁱᶿ)𝑑𝜽 ⇒2𝐼=𝑖𝑟²∫𑀾 (𝑒ⁱ²ᶿ 1)𝑑𝜽 ⇒2𝐼=𝑖𝑟²∫𑀾 (𝑐𝑜𝑠2𝜽 𝑖𝑠𝑖𝑛2𝜽 1)𝑑𝜽 Here limits are 0→2𝝅 for...
Evaluate ∫𑀾 |𝑧| 𝑑𝑧 , where 𝐶 is the upper half of circle |𝑧|=1 .5:57Evaluate ∫𑀾 |𝑧| 𝑑𝑧 , where 𝐶 is the upper half of circle |𝑧|=1 .
Evaluate ∫𑀾 |𝑧| 𝑑𝑧 , where 𝐶 is the upper half of circle |𝑧|=1 .
Переглядів 6 тис.Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Evaluate ∫𑀾 |𝑧| 𝑑𝑧 , where 𝐶 is the upper half of circle |𝑧|=1 . 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- According to Riemann's Definition of Integration - ∫𑀾 𝑓(𝑧)𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ 𝑓 (𝑒ᵣ)(𝑧ᵣ - 𝑧ᵣ₋₁) ......(1) Where 𝑒ᵣ is any point on the arc joining the points Here 𝑓(𝑧)=|𝑧| ⇒ 𝑓 (𝑒ᵣ)=|𝑒ᵣ| =1 because 𝑒ᵣ is liying on circle |𝑧|=1 and for this Circle 𝑧₀=-1 and last poin...
Evaluate ∫𑀾 𝑧𝑑𝑧 .6:16Evaluate ∫𑀾 𝑧𝑑𝑧 .
Evaluate ∫𑀾 𝑧𝑑𝑧 .
Переглядів 1,3 тис.Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Evaluate ∫𑀾 𝑧𝑑𝑧. 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- According to Riemann's Definition of Integration - ∫𑀾 𝑓(𝑧)𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ 𝑓 (𝑒ᵣ)(𝑧ᵣ - 𝑧ᵣ₋₁) ....(1) Put 𝑓(𝑧)=z ⇒ 𝑓 (𝑒ᵣ)=𝑒ᵣ we get ⇒∫𑀾 𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ 𝑒ᵣ(𝑧ᵣ - 𝑧ᵣ₋₁) .....(2) Now take 𝑒ᵣ=𝑧ᵣ₋₁ Putting in equation (2) ⇒∫𑀾 |𝑑𝑧|= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ 𝑧ᵣ₋₁(𝑧ᵣ - 𝑧ᵣ₋₁) .......(3) Now again take 𝑒ᵣ=𝑧ᵣ Putting in equation (...
Evaluate ∫𑀾 |𝑑𝑧| .3:12Evaluate ∫𑀾 |𝑑𝑧| .
Evaluate ∫𑀾 |𝑑𝑧| .
Переглядів 435Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Evaluate ∫𑀾 |𝑑𝑧| . 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- According to Riemann's Definition of Integration - ∫𑀾 𝑓(𝑧)𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ 𝑓 (𝑒ᵣ)(𝑧ᵣ - 𝑧ᵣ₋₁) Put 𝑓(𝑧)=1 ⇒ 𝑓 (𝑒ᵣ)=1 we get ⇒∫𑀾 𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ (𝑧ᵣ - 𝑧ᵣ₋₁) Taking mod of both side ⇒∫𑀾 |𝑑𝑧|= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ |𝑧ᵣ - 𝑧ᵣ₋₁| ⇒∫𑀾 |𝑑𝑧|= 𝐥𝐢𝐦ₙ ͢ ͚( |𝑧₁ - 𝑧₀| |𝑧₂ - 𝑧₁| ..... |𝑧ₙ - 𝑧ₙ₋₁| ) ⇒∫𑀾 |𝑑𝑧|= 𝑙 ( lenght of the curv...
Happy New Year 2023 🔥 | Stay Tuned | 🔥0:18Happy New Year 2023 🔥 | Stay Tuned | 🔥
Happy New Year 2023 🔥 | Stay Tuned | 🔥
Переглядів 25Рік тому
🔥🔥🔥Hurry Up Students for whole detailed Videos with Answer in Description for making notes 🔥🔥🔥 🔥🔥🔥JOIN JOIN JOIN JOIN 🔥🔥🔥 👉 www.youtube.com/@abcstudy 🔥🔥🔥All B.Sc., B.Tech , M.Sc. , M.A. Mathematics students join with us A great platform to get knowledge in Mathematics and science... Stay tuned....for detailed videos on whole SYLLABUS with topic wise...and also join us for CSIR-UGC National Elig...
Evaluate ∫𑀾 𝑑𝑧 in Complex Integration.3:29Evaluate ∫𑀾 𝑑𝑧 in Complex Integration.
Evaluate ∫𑀾 𝑑𝑧 in Complex Integration.
Переглядів 1,1 тис.Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Evaluate ∫𑀾 𝑑𝑧 . 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- According to Riemann's Definition of Integration - ∫𑀾 𝑓(𝑧)𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ 𝑓 (𝑒ᵣ)(𝑧ᵣ - 𝑧ᵣ₋₁) Put 𝑓(𝑧)=1 ⇒ 𝑓 (𝑒ᵣ)=1 we get ⇒∫𑀾 𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚Σᵣ₌ᷠ₁ (𝑧ᵣ - 𝑧ᵣ₋₁) ⇒∫𑀾 𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚{(𝑧₁ - 𝑧₀) (𝑧₂ - 𝑧₁) ..... (𝑧ₙ - 𝑧ₙ₋₁)} ⇒∫𑀾 𝑑𝑧= 𝐥𝐢𝐦ₙ ͢ ͚(𝑧ₙ - 𝑧₀) ∵ 𝑧ₙ=𝑏 and 𝑧₀=𝑎 ⇒∫𑀾 𝑑𝑧= 𝑏 - 𝑎 For closed curve we know that points 𝑎 and...
Define the Complex line integral.OR The Riemann's Definition of Integration in Complex Integration .9:31Define the Complex line integral.OR The Riemann's Definition of Integration in Complex Integration .
Define the Complex line integral.OR The Riemann's Definition of Integration in Complex Integration .
Переглядів 731Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒍 𝑸𝒖𝒆𝒔𝒕𝒊𝒐𝒏:- Define the Complex line integral. Or The Riemann's Definition of Integration . 𝑺𝒐𝒍𝒖𝒕𝒊𝒐𝒏:- Let 𝑧= 𝑧(𝑡)=𝑥(𝑡) 𝑖𝑦(𝑡) , 𝑎 ≤ 𝑡 ≤ 𝑏 be 𝑎 given curve 𝐶 joining 𝑎 and 𝑏 and let 𝑓(𝑧) be a function of Complex variable 𝑧 defined and continues on 𝐶. Consider the partition 𝑃={𝑎=𝑡₀ , 𝑡₁ , 𝑡₂ ,......, 𝑡ₙ=𝑏} of the [𝑎,𝑏] . Let 𝑧₀ , 𝑧₁ , 𝑧₂ ,......, 𝑧ₙ be...
Rectifiable Curve in Complex Integration.7:54Rectifiable Curve in Complex Integration.
Rectifiable Curve in Complex Integration.
Переглядів 1,8 тис.Рік тому
𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑨𝒏𝒂𝒍𝒚𝒔𝒊𝒔 𝑻𝒉𝒆𝒐𝒓𝒆𝒎 𝒇𝒓𝒐𝒎 𝑪𝒐𝒎𝒑𝒍𝒆𝒙 𝑰𝒏𝒕𝒆𝒈𝒓𝒂𝒕𝒊𝒐𝒏 Rectifiable curve :- Let 𝑧= 𝑧(𝑡)=𝑥(𝑡) 𝑖𝑦(𝑡) be any given curve or parametric point and let 𝑡 take up any value between 𝑎 and 𝑏 , 𝑎 ≤ 𝑡 ≤ 𝑏 . Let 𝑃={𝑡₀ , 𝑡₁ , 𝑡₂ ,......, 𝑡ₙ} be a partition of [𝑎,𝑏] . If 𝑃₀ , 𝑃₁ , 𝑃₂ ,......, 𝑃ₙ be the points on the curve corresponding to the points 𝑡₀ , 𝑡₁ , 𝑡₂ ,......, 𝑡ₙ then the length of the polygonal line 𝑃...
Continuous Jordan curve & Contour.5:32Continuous Jordan curve & Contour.
Continuous Jordan curve & Contour.
Переглядів 313Рік тому
Continuous Jordan curve & Contour.
Jordan Arc and Regular Arc of Jordan Arc.3:46Jordan Arc and Regular Arc of Jordan Arc.
Jordan Arc and Regular Arc of Jordan Arc.
Переглядів 987Рік тому
Jordan Arc and Regular Arc of Jordan Arc.
Continuous Arc and Multiple Point in Complex Integration.4:46Continuous Arc and Multiple Point in Complex Integration.
Continuous Arc and Multiple Point in Complex Integration.
Переглядів 606Рік тому
Continuous Arc and Multiple Point in Complex Integration.
Partition and Norm of the partition in Complex Integration ꯫𝑃꯫ .3:47Partition and Norm of the partition in Complex Integration ꯫𝑃꯫ .
Partition and Norm of the partition in Complex Integration ꯫𝑃꯫ .
Переглядів 176Рік тому
Partition and Norm of the partition in Complex Integration ꯫𝑃꯫ .
Discuss the logarithmic transformation 𝑤=𝒍𝒐𝒈𝑧 .9:24Discuss the logarithmic transformation 𝑤=𝒍𝒐𝒈𝑧 .
Discuss the logarithmic transformation 𝑤=𝒍𝒐𝒈𝑧 .
Переглядів 1,8 тис.Рік тому
Discuss the logarithmic transformation 𝑤=𝒍𝒐𝒈𝑧 .
Discuss the exponential transformation 𝑤=𝑒ᶻ .24:08Discuss the exponential transformation 𝑤=𝑒ᶻ .
Discuss the exponential transformation 𝑤=𝑒ᶻ .
Переглядів 402Рік тому
Discuss the exponential transformation 𝑤=𝑒ᶻ .
Mapping 𝑤=𝑧² maps 𝐈 quadrant in 𝑧-plane bounded circles |𝑧|=𝑎,|𝑧|=𝑏 (𝑎≻𝑏≻0). Is mapping conformal ?7:27Mapping 𝑤=𝑧² maps 𝐈 quadrant in 𝑧-plane bounded circles |𝑧|=𝑎,|𝑧|=𝑏 (𝑎≻𝑏≻0). Is mapping conformal ?
Mapping 𝑤=𝑧² maps 𝐈 quadrant in 𝑧-plane bounded circles |𝑧|=𝑎,|𝑧|=𝑏 (𝑎≻𝑏≻0). Is mapping conformal ?
Переглядів 645Рік тому
Mapping 𝑤=𝑧² maps 𝐈 quadrant in 𝑧-plane bounded circles |𝑧|=𝑎,|𝑧|=𝑏 (𝑎≻𝑏≻0). Is mapping conformal ?
Show 𝑧=⎷𝑤 transform family of circles |𝑤-1|=𝑐 into family of lemniscate |𝑧-1||𝑧+1|=𝑐 (𝑐=parameter).11:07Show 𝑧=⎷𝑤 transform family of circles |𝑤-1|=𝑐 into family of lemniscate |𝑧-1||𝑧+1|=𝑐 (𝑐=parameter).
Show 𝑧=⎷𝑤 transform family of circles |𝑤-1|=𝑐 into family of lemniscate |𝑧-1||𝑧+1|=𝑐 (𝑐=parameter).
Переглядів 448Рік тому
Show 𝑧=⎷𝑤 transform family of circles |𝑤-1|=𝑐 into family of lemniscate |𝑧-1||𝑧 1|=𝑐 (𝑐=parameter).
By transformation 𝑤= 𝑧² show circle |𝑧-𝑎|=𝑐 (𝑎,𝑐 real) in 𝑧-plane correspond to limancons in 𝑤-plane9:02By transformation 𝑤= 𝑧² show circle |𝑧-𝑎|=𝑐 (𝑎,𝑐 real) in 𝑧-plane correspond to limancons in 𝑤-plane
By transformation 𝑤= 𝑧² show circle |𝑧-𝑎|=𝑐 (𝑎,𝑐 real) in 𝑧-plane correspond to limancons in 𝑤-plane
Переглядів 987Рік тому
By transformation 𝑤= 𝑧² show circle |𝑧-𝑎|=𝑐 (𝑎,𝑐 real) in 𝑧-plane correspond to limancons in 𝑤-plane
Find Bilinear Transformation that maps the points 𝑧₁=∞, 𝑧₂= 𝑖, 𝑧₃=0 into the points 𝑤₁=0, 𝑤₂=𝑖, 𝑤₃=∞5:49Find Bilinear Transformation that maps the points 𝑧₁=∞, 𝑧₂= 𝑖, 𝑧₃=0 into the points 𝑤₁=0, 𝑤₂=𝑖, 𝑤₃=∞
Find Bilinear Transformation that maps the points 𝑧₁=∞, 𝑧₂= 𝑖, 𝑧₃=0 into the points 𝑤₁=0, 𝑤₂=𝑖, 𝑤₃=∞
Переглядів 6 тис.Рік тому
Find Bilinear Transformation that maps the points 𝑧₁=∞, 𝑧₂= 𝑖, 𝑧₃=0 into the points 𝑤₁=0, 𝑤₂=𝑖, 𝑤₃=∞
Prove that the cross ratio remains invariant under a Bilinear Transformation.10:48Prove that the cross ratio remains invariant under a Bilinear Transformation.
Prove that the cross ratio remains invariant under a Bilinear Transformation.
Переглядів 3,6 тис.Рік тому
Prove that the cross ratio remains invariant under a Bilinear Transformation.
Cross Ratio & it's relation with Bilinear Transformation .6:39Cross Ratio & it's relation with Bilinear Transformation .
Cross Ratio & it's relation with Bilinear Transformation .
Переглядів 305Рік тому
Cross Ratio & it's relation with Bilinear Transformation .
Nature of a Bilinear Transformation | Hyperbolic, Elliptical, Loxodromic, Parabolic |9:07Nature of a Bilinear Transformation | Hyperbolic, Elliptical, Loxodromic, Parabolic |
Nature of a Bilinear Transformation | Hyperbolic, Elliptical, Loxodromic, Parabolic |
Переглядів 461Рік тому
Nature of a Bilinear Transformation | Hyperbolic, Elliptical, Loxodromic, Parabolic |
Find the Normal form of Bilinear Transformation which has one fixed point 𝑝 & other fixed point is ∞4:06Find the Normal form of Bilinear Transformation which has one fixed point 𝑝 & other fixed point is ∞
Find the Normal form of Bilinear Transformation which has one fixed point 𝑝 & other fixed point is ∞
Переглядів 818Рік тому
Find the Normal form of Bilinear Transformation which has one fixed point 𝑝 & other fixed point is ∞
Every Bilinear Transform with one fixed point 𝑝 can be put in 1/(𝑤-𝑝)=𝑘+{1/(𝑧-𝑝)} where 𝑘=𝑎/(𝑎-𝑐𝑝).8:10Every Bilinear Transform with one fixed point 𝑝 can be put in 1/(𝑤-𝑝)=𝑘+{1/(𝑧-𝑝)} where 𝑘=𝑎/(𝑎-𝑐𝑝).
Every Bilinear Transform with one fixed point 𝑝 can be put in 1/(𝑤-𝑝)=𝑘+{1/(𝑧-𝑝)} where 𝑘=𝑎/(𝑎-𝑐𝑝).
Переглядів 1,1 тис.Рік тому
Every Bilinear Transform with one fixed point 𝑝 can be put in 1/(𝑤-𝑝)=𝑘 {1/(𝑧-𝑝)} where 𝑘=𝑎/(𝑎-𝑐𝑝).
State & proof Normal form of Bilinear Transformation: (𝑤-𝑝)/(𝑤-𝑞)=𝑘(𝑧-𝑝)/(𝑧-𝑞) where𝑘=(𝑎-𝑐𝑝)/(𝑎-𝑐𝑞)8:23State & proof Normal form of Bilinear Transformation: (𝑤-𝑝)/(𝑤-𝑞)=𝑘(𝑧-𝑝)/(𝑧-𝑞) where𝑘=(𝑎-𝑐𝑝)/(𝑎-𝑐𝑞)
State & proof Normal form of Bilinear Transformation: (𝑤-𝑝)/(𝑤-𝑞)=𝑘(𝑧-𝑝)/(𝑧-𝑞) where𝑘=(𝑎-𝑐𝑝)/(𝑎-𝑐𝑞)
Переглядів 583Рік тому
State & proof Normal form of Bilinear Transformation: (𝑤-𝑝)/(𝑤-𝑞)=𝑘(𝑧-𝑝)/(𝑧-𝑞) where𝑘=(𝑎-𝑐𝑝)/(𝑎-𝑐𝑞)

КОМЕНТАРІ

  • @martonsinka5546
    @martonsinka5546 19 годин тому

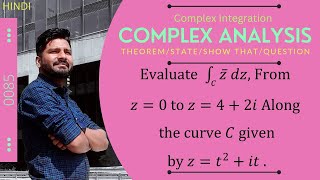
    Isn't it circular proof? You used continuity when you said lim(x-a)=0 if x->a

  • @Pihuofficial502
    @Pihuofficial502 4 дні тому

    Sir how this happens lim n tends to infinity an Z0^n=0

  • @rahuljalal5830
    @rahuljalal5830 11 днів тому

    Can you verify this question also please

  • @amansaharan6821
    @amansaharan6821 15 днів тому

    Sir agge bhi bnao video leibniz rule Cauchy integral formula for disk j.b. conway

  • @cornorjakeson8083
    @cornorjakeson8083 15 днів тому

    Lovly

  • @21yadav42
    @21yadav42 24 дні тому

    Prove that the function f(z)={ 𝑥 3(1+𝑖)−𝑦 3(1−𝑖) 𝑥 2+𝑦2 , (z≠ 0) 0 , (z= 0) is continuous and the cauchys-riemann equations are satisfied at the origin .if f ′(0)does not exist.

  • @gauravkmwt1610
    @gauravkmwt1610 28 днів тому

    Smjh to do 😂

  • @KPrabhavathi-p8n
    @KPrabhavathi-p8n Місяць тому

    Not understand properlly

  • @maheshwaribawankule915
    @maheshwaribawankule915 Місяць тому

    You are a saver in exam time ❤

  • @utkarshpandey2628
    @utkarshpandey2628 Місяць тому

    Epsilon publication hai

  • @Ruth-jm7ob
    @Ruth-jm7ob Місяць тому

    Thanks for the wonderful teaching ❤

  • @Nkitpraj
    @Nkitpraj 2 місяці тому

    bhai bs intro change karde sach me bhaiii

  • @freeshorts7739
    @freeshorts7739 2 місяці тому

    The answer is wrong right answer is 1.....

  • @monsterra4841
    @monsterra4841 2 місяці тому

    why take er as zr-1

  • @Gupta-b1z
    @Gupta-b1z 3 місяці тому

    🎉

  • @pkentertainments7105
    @pkentertainments7105 3 місяці тому

    Super sir

  • @vijaychinthapalli274
    @vijaychinthapalli274 3 місяці тому

    ❤❤thanks guru

  • @Kuldeep-75180
    @Kuldeep-75180 3 місяці тому

    Sir last me samajh nhi aya

  • @gamingparesh2413
    @gamingparesh2413 3 місяці тому

    Thanks sir

  • @bestytvideos
    @bestytvideos 3 місяці тому

    root r ka square r square kaise last step mein

  • @d1rshaan
    @d1rshaan 3 місяці тому

    👏👏

  • @VLSILovers
    @VLSILovers 4 місяці тому

    Source of this Question?

  • @pandey7372
    @pandey7372 4 місяці тому

    Cauchy reimann eqn necessary condition hai lekin sufficient condition nhi hote hai analytic proof krne k liye isme CR eqn satisfy toh hore hai lekin necessary condition ka ky wo hote hai ki jo partial derivatives hai wo b toh continuous hone chaeye na toh isme wo nhi ho rhe (the single valued fb fz is analytic in domain D if the four partial derivatives ux vx uy vy are continuous and satisfy CR eqn ) in each point D !! Isliye ye analytic nhi hai

  • @pandey7372
    @pandey7372 4 місяці тому

    😂phle khid smjh lo chle ho utube m pdane ko

  • @JumbleGaming01
    @JumbleGaming01 4 місяці тому

    Intro 💀

  • @priyeshverma9353
    @priyeshverma9353 5 місяців тому

    Awesome explanation sir ❤

  • @studentaccount0016
    @studentaccount0016 5 місяців тому

    Pls explain last line how you have written it "dw/dz exist for all finite value .........."

  • @Kislay-jj2wn
    @Kislay-jj2wn 5 місяців тому

    Very helpful video

  • @Kislay-jj2wn
    @Kislay-jj2wn 5 місяців тому

    Sir pls explain, cut along the positive real axis from 0 to infinity ka Matlab kya hai

  • @QuickTricksBySatyam
    @QuickTricksBySatyam 5 місяців тому

    ❤

  • @QuickTricksBySatyam
    @QuickTricksBySatyam 5 місяців тому

    Thanks for easy explanation 👍 sir

  • @AnjaliGupta-rh6sv
    @AnjaliGupta-rh6sv 6 місяців тому

    Thank you Sir

  • @suyashsahu6505
    @suyashsahu6505 6 місяців тому

    2:02 x^2+y^2 hoga vaha

    • @abcstudy
      @abcstudy 6 місяців тому

      You're right, it's writing mistake 👍

  • @SuhailNabiTheFuture
    @SuhailNabiTheFuture 6 місяців тому

    ye meri copy pe b likha hai , i expected explanation, which is missing

    • @Metrqchoe
      @Metrqchoe 6 місяців тому

      Wahi to...explain ka nam or Nishan nhi hay

  • @sooraj1323
    @sooraj1323 6 місяців тому

    nice explanation ,keep up doing the great work

  • @Alokyadav-dq7hk
    @Alokyadav-dq7hk 6 місяців тому

    Level sable niklenge pr niklenge usi me Jo bhayia se padegya 🎉

  • @VaibhavSingh-nv2yv
    @VaibhavSingh-nv2yv 6 місяців тому

    Wow wonder full explanation .....wow wow wow

  • @ektadk7872
    @ektadk7872 7 місяців тому

    Wow kitne achhe se represent kra h🤗

  • @ektadk7872
    @ektadk7872 7 місяців тому

    Sir plz continue....nice explaination 😊

  • @aadityasuralkar5210
    @aadityasuralkar5210 7 місяців тому

    uselesss

  • @rofficial3121
    @rofficial3121 7 місяців тому

    Find complex numbers satisfying |z−1|=|z−3|=|z−i|,

  • @muskangupta066
    @muskangupta066 8 місяців тому

    Smjhaya too hai hi nahi apn ? Note too hum.book s bhi kr lenge

  • @chickenfamily3711
    @chickenfamily3711 8 місяців тому

    🎉

  • @divyasati9091
    @divyasati9091 8 місяців тому

    Or dimak kharab ho gya khali chap rkha rt rkha smjh me to kuch nhi aara

  • @noobgamerz833
    @noobgamerz833 8 місяців тому

    Thank you😊👍

  • @nishtha.s02
    @nishtha.s02 9 місяців тому

    🙏🏽👍🏽👍🏽

  • @College-u6n
    @College-u6n 9 місяців тому

    Thank you sir

  • @Mr.respect3.00
    @Mr.respect3.00 9 місяців тому

    ❤❤

  • @harikaasi882
    @harikaasi882 9 місяців тому

    Continuous formulae use cheyadaniki avvada

  • @muskanmeena-g5m
    @muskanmeena-g5m 9 місяців тому

    Yoo your method is so esy I am confused when I see my slove paper and then I search and I found your class thnx