Відео

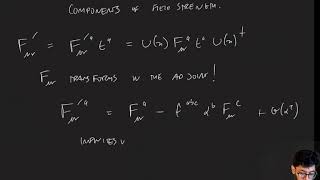
take a bow. You are a hero.

Are you the son of Dr.Md.Jafor Iqbal?

বেত দিয়ে মারবেন নাকি আপনিও কি আর সব স্যরের মত

আপনি যদি আমার স্যার হবেন তাহলে আপনি আমাকে মারবেন নাকি

আমার পাশে এসে দাঁড়ান আমাকে সাহায্য করেন

স্যার আপনি কত টাকায় আমাকে পড়াবেন

দয়া করেন ২০২৫ সালে আমি যেন দশম শ্রেনীতে উঠতে পারি

আপনি খুব ভালো

স্যার আপনার কাছে কথা বলতে ইচ্ছে করে

আমি আপনাকে খুব আদর যত্ন করবো

Could anybody tell me at what frequencies is an electron vibrating at if we consider it to be a string. Can we generate these frequencies in the lab using really sophisticated electronics therefore produce different particles in a lab . Probably a dumb question my part. But I sure would like to know range of frequencies we are dealing with.

@ 5:46 Unfortunately, there is no physical explanation for quantum fluctuations. Heisenberg's uncertainty principle is nothing but a mathematical description of these postulated fluctuations. P.S.(1) The Casimir-effect can be described using classical physics and therefore isn't proving the existence of quantum fluctuations (anymore). P.S.(2) When you don't know (or mention) the limitations of a theory, it's impossible to apply it.

I never bought the 'curling up' argument of the extra dimensions in string theory. There is a fundamental contradiction in on the one hand arguing that these dimensions are essential for the theory to make sense, and on the other hand arguing that they can be ignored because they are tiny. If tininess was ever an argument then quantum mechanics itself would be irrelevant. You can't have it both ways, saying dimensions are essential and ignorable at the same time. Something is wrong in a theory that is based on that logical inconsistency.

Ghora beshi english maray

Quantum gravity is non-renormalizable because gravitation simply isn't quantum. Classical mechanics is not quantum mechanics. Gravitation is simply inertia to acceleration; the acceleration that is the expansion of the universe as indicated by the Einstein Equivalence Principle. As is known from the Weinburg-Witten theorem, "...in all theories with a Lorentz-covariant energy-momentum tensor, such as all known renormalizable quantum field theories, composite as well as elementary massless particles with j > 1 are forbidden." That means no such things as gravitons or anything greater than a spin-1 particle. String 'theory' had been retained after QCD had been validated only because it had resulted in a massless spin-2 particle imagined to be the graviton. That it had also resulted in a tachyon and 26 dimensions did not detour others from continuing its pursuit. The dimensions themselves are a product of applying the Goddard-Thorn 'no-ghosts' theorem to eliminate the negative probabilities it originally had. It wasn't until 1974 that the proposed strings had been reduced in size from 10^13 to 10^33 to transform the theory from being a bosonic theory to being a theory for the accommodation of quantum gravity. There is great irony in a repeatedly failed hypothesis of many dimensions to have arisen out of the S-matrix theory that had denied the mere four dimensions of spacetime. Just as gravitation is inertia to the fourth-dimensional oscillation that is time, quantum mechanics is motion from the fourth-dimensional oscillation that is time. They are at the opposite ends of a mass/energy scale compliant with the Correspondence Principle.

Good afternoon, Professor. I want to express my gratitude for your enlightening lecture. Each step of the calculations has been meticulously justified, which I greatly appreciate. However, I have a question regarding the integration over omega, weighted by a Gaussian function. Could you elaborate on the justification for this step? Additionally, when you performed the delta functional integral, you justified it by its value being one. I noticed a change in the normalization factor from 𝑛 to 𝑛' , which caught my attention. Could you please clarify the reasoning behind this alteration? Thank you once again for your thorough explanation.

Thank you for breaking this down. A super introduction!

For Grassmann numbers, does it make sense to do integrals "from the left or right" like the case with derivatives?

If we were to flip the order of integration ∫dθ ∫dη [ ] → ∫dη ∫dθ [ ] we should give it a minus sign, right?

"pesky lake of experiments..." I love your presentation!

listening to you reminds me of listening to Sean Carrol

Don't mention the name "Woit" here! 🙂

The missing link with experiments is the poor side of any QFT-course.

This is really useful: 8:14

Genius

Maldacena 🇦🇷🇦🇷🇦🇷🇦🇷

you are an utterly amazing teacher sir

Keep sharing Nabil ✨

Much more interesting physics in quantum world and perhaps the most powerful method without operators !

Is there an alternative interpretation of "Asymptotic Freedom"? What if Quarks are actually made up of twisted tubes which become physically entangled with two other twisted tubes to produce a proton? Instead of the Strong Force being mediated by the exchange of gluons, it would be mediated by the physical entanglement of these twisted tubes. When only two twisted tubules are entangled, a meson is produced which is unstable and rapidly unwinds (decays) into something else. A proton would be analogous to three twisted rubber bands becoming entangled and the "Quarks" would be the places where the tubes are tangled together. The behavior would be the same as rubber balls (representing the Quarks) connected with twisted rubber bands being separated from each other or placed closer together producing the exact same phenomenon as "Asymptotic Freedom" in protons and neutrons. The force would become greater as the balls are separated, but the force would become less if the balls were placed closer together. ------------------------ String Theory was not a waste of time. Geometry is the key to Math and Physics. What if we describe subatomic particles as spatial curvature, instead of trying to describe General Relativity as being mediated by particles? Quantum Entangled Twisted Tubules: “We are all agreed that your theory is crazy. The question which divides us is whether it is crazy enough to have a chance of being correct.” Neils Bohr (lecture on a theory of elementary particles given by Wolfgang Pauli in New York, c. 1957-8, in Scientific American vol. 199, no. 3, 1958) The following is meant to be a generalized framework for an extension of Kaluza-Klein Theory. Does it agree with the “Twistor Theory” of Roger Penrose? During the early history of mankind, the twisting of fibers was used to produce thread, and this thread was used to produce fabrics. The twist of the thread is locked up within these fabrics. Is matter made up of twisted 3D-4D structures which store spatial curvature that we describe as “particles"? Are the twist cycles the "quanta" of Quantum Mechanics? When we draw a sine wave on a blackboard, we are representing spatial curvature. Does a photon transfer spatial curvature from one location to another? Wrap a piece of wire around a pencil and it can produce a 3D coil of wire, much like a spring. When viewed from the side it can look like a two-dimensional sine wave. You could coil the wire with either a right-hand twist, or with a left-hand twist. Could Planck's Constant be proportional to the twist cycles. A photon with a higher frequency has more energy. ( E=hf, More spatial curvature as the frequency increases = more Energy ). What if gluons are actually made up of these twisted tubes which become entangled with other tubes to produce quarks. (In the same way twisted electrical extension cords can become entangled.) Therefore, the gluons are a part of the quarks. Quarks cannot exist without gluons, and vice-versa. Mesons are made up of two entangled tubes (Quarks/Gluons), while protons and neutrons would be made up of three entangled tubes. (Quarks/Gluons) The "Color Force" would be related to the XYZ coordinates (orientation) of entanglement. "Asymptotic Freedom", and "flux tubes" are logically based on this concept. The Dirac “belt trick” also reveals the concept of twist in the ½ spin of subatomic particles. If each twist cycle is proportional to h, we have identified the source of Quantum Mechanics as a consequence twist cycle geometry. Modern physicists say the Strong Force is mediated by a constant exchange of Mesons. The diagrams produced by some modern physicists actually represent the Strong Force like a spring connecting the two quarks. Asymptotic Freedom acts like real springs. Their drawing is actually more correct than their theory and matches perfectly to what I am saying in this model. You cannot separate the Gluons from the Quarks because they are a part of the same thing. The Quarks are the places where the Gluons are entangled with each other. Neutrinos would be made up of a twisted torus (like a twisted donut) within this model. The twist in the torus can either be Right-Hand or Left-Hand. Some twisted donuts can be larger than others, which can produce three different types of neutrinos. Gravity is a result of a very small curvature imbalance within atoms. (This is why the force of gravity is so small.) Instead of attempting to explain matter as "particles", this concept attempts to explain matter more in the manner of our current understanding of the space-time curvature of gravity. If an electron has qualities of both a particle and a wave, it cannot be either one. It must be something else. Therefore, a "particle" is actually a structure which stores spatial curvature. Can an electron-positron pair (which are made up of opposite directions of twist) annihilate each other by unwinding into each other producing Gamma Ray photons? Does an electron travel through space like a threaded nut traveling down a threaded rod, with each twist cycle proportional to Planck’s Constant? Does it wind up on one end, while unwinding on the other end? Is this related to the Higgs field? Does this help explain the strange ½ spin of many subatomic particles? Does the 720 degree rotation of a 1/2 spin particle require at least one extra dimension? Alpha decay occurs when the two protons and two neutrons (which are bound together by entangled tubes), become un-entangled from the rest of the nucleons . Beta decay occurs when the tube of a down quark/gluon in a neutron becomes overtwisted and breaks producing a twisted torus (neutrino) and an up quark, and the ejected electron. The phenomenon of Supercoiling involving twist and writhe cycles may reveal how overtwisted quarks can produce these new particles. The conversion of twists into writhes, and vice-versa, is an interesting process. Gamma photons are produced when a tube unwinds producing electromagnetic waves. >>>>>>>>>>>>>>>>>>>>>> Within this model a black hole could represent a quantum of gravity, because it is one cycle of spatial gravitational curvature. Therefore, instead of a graviton being a subatomic particle it could be considered to be a black hole. The overall gravitational attraction would be caused by a very tiny curvature imbalance within atoms. We know there is an unequal distribution of electrical charge within each atom because the positive charge is concentrated within the nucleus, even though the overall electrical charge of the atom is balanced by equal positive and negative charge. >>>>>>>>>>>>>>>>>>>>>> In this model Alpha equals the compactification ratio within the twistor cone, which is approximately 1/137. 1= Hypertubule diameter at 4D interface 137= Cone’s larger end diameter at 3D interface where the photons are absorbed or emitted. The 4D twisted Hypertubule gets longer or shorter as twisting or untwisting occurs. (720 degrees per twist cycle.) >>>>>>>>>>>>>>>>>>>>>>> How many neutrinos are left over from the Big Bang? They have a small mass, but they could be very large in number. Could this help explain Dark Matter? >>>>>>>>>>>>>>>>>>>>>>>> Why did Paul Dirac use the twist in a belt to help explain particle spin? Is Dirac’s belt trick related to this model? Is the “Quantum” unit based on twist cycles? ++++++++++++++++++++++++++++++++++++++++++++++++++++++++++++++++++++++++++ I started out imagining a subatomic Einstein-Rosen Bridge whose internal surface is twisted with either a Right-Hand twist, or a Left-Hand twist. The model grew out of that simple idea. I was also trying to imagine a way to stuff the curvature of a 3 D sine wave into subatomic particles. .

Ami tomar vabi hoi ami tomar jonno ekta nam rekhechi namti holo tattu ghore tomar mukh kheke ghora ghora shbdo ashe tmi shudhu ghora ghorai bolte paro thik bolechi na

Fantastic video, thank you! I am writing my thesis right now and I've been confused about some specific steps you covered and explained here. I have been jumping between like 5+ sets of lecture notes to find an explanation of why we're allowed to assume the measure is invariant, or at the very least be able to discuss the implications of such an assumption. Tong's lecture notes just state it as a thing without further clarification. In none of the notes has the keyword "anomaly" been used, which not only clears up my issue but it also elucidates another concept I had heard about but didn't have any sort of theoretical entry-point for.

This is not the correct introduction to string theory. Please consider the original literature from the 1960s. You won't understand string theory if you think of it this way, although it is common in introductory books to treat it this way.

Can you record a video on important problems and current approaches with some references? I am an undergrad student in Turkey and honestly I don't think anyone here works on these problems that can provide guidance to me. I would really appreciate such a video, otherwise I'll just keep reading some lecture notes that I've found on arXiv without a concrete idea of what and why I do it.

this channel is amazing!!!

At 2:45 psi bar has a prime at the right hand side were it shouldnt have.

Grassman numbers = Absolutely crackers.

Great explanation 🎉🎉🎉

ami ononna

Nabil vaiya tomader nuhashke bole dibe ami take khub bhalobashi

ami tomar nuhasher bou hoi

Nabil tumi block chutao😢😢😢

Nabil kemon acho vaya

ami onnona

do you block me or forgive me broter

tomader nuhash vaiyar bodhu

Ami ononna

Ami tomar vabi hoi

Amar Ekta shotru chai ekta ekta ekta shotru chai keno amare chinte parchen na

Amar Naam Rapunzel ha ha ha