
- 27
- 45 102
Anup Rao
Приєднався 25 тра 2009
Borsuk-Ulam Theorem
I give a proof of the Borsuk-Ulam theorem.
You can find a written version of the proof here:
kam.mff.cuni.cz/~matousek/akt.html
You can find a written version of the proof here:
kam.mff.cuni.cz/~matousek/akt.html
Переглядів: 836
Відео
How to hang a picture using algebraic topology
Переглядів 2613 місяці тому
How can you hang a picture using n nails, so that if you pull out any one nail, the picture falls down?
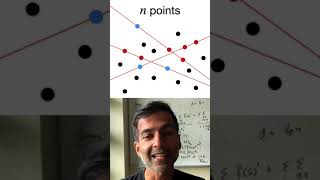
The Sylvester-Gallai Theorem
Переглядів 3163 місяці тому
I explain a nice proof of the Sylvester-Gallai Theorem References: en.wikipedia.org/wiki/Sylvester–Gallai_theorem
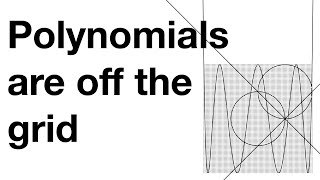
Counting grid points on a curve
Переглядів 2564 місяці тому
I discuss how to prove that polynomials cannot hit too many integer points in a given grid. For references and history, see: arxiv.org/pdf/2312.12890
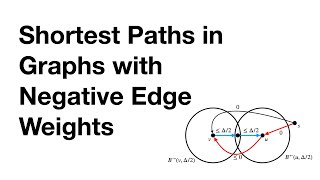
Shortest paths in graphs with negative edge weights, in nearly linear time
Переглядів 3605 місяців тому
Recently there has been some remarkable progress in the design of fast algorithms for finding shortest paths in graphs with negative edge weights. I discuss the key ideas of this recent work, which is based on these papers: Bernstein, Nanongkai, Wulff-Nilsen: arxiv.org/pdf/2203.03456 Bringmann, Cassis, Fischer: arxiv.org/pdf/2304.05279
Finding features of the Economy using Trade Networks
Переглядів 1626 місяців тому
I discuss the concept of trade networks, which I believe can be used to find efficiencies and obstacles to efficiencies in a large economy. Further discussion can be found in this paper: arxiv.org/abs/1702.03290
Gödel's incompleteness theorem: a conceptual explanation
Переглядів 6 тис.Рік тому
I explain Gödel's famous theorem at a high level. This form of the theorem is due to Chaitin. References and history can be found here: en.wikipedia.org/wiki/Gödel's_incompleteness_theorems#:~:text=Chaitin's incompleteness theorem states that,Kolmogorov complexity greater than c.
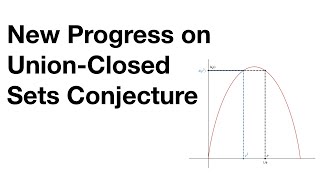
New progress on the Union-closed sets conjecture
Переглядів 2,2 тис.Рік тому
Recently there has been some exciting progress on the famous union-closed sets conjecture. I describe the beautiful ideas, which appeared in these papers: Justin Gilmer: arxiv.org/pdf/2211.09055.pdf Will Sawin: arxiv.org/pdf/2211.11504.pdf Ravi Bopanna arxiv.org/pdf/2301.09664.pdf
How much money is out there?
Переглядів 297Рік тому
I talk about how including the cash held at the treasury changes the count for how much money was injected into the system in the last couple of years.
Is the Fed easing or tightening? Yes.
Переглядів 144Рік тому
I give a short update on what the Fed has been upto since the Silicon Valley Bank crisis.
Silicon Valley Bank and the US Dollar
Переглядів 396Рік тому
I explain the big change made by the Fed to the meaning of US Dollars and other kinds of assets in the wake of the Silicon Vally Bank crisis.
Finding small gaps between the primes
Переглядів 1,6 тис.Рік тому
I describe recent progress towards proving the twin primes conjecture. The presentation here is largely based on this paper of James Maynard: arxiv.org/pdf/1311.4600.pdf 00:00 Introduction 05:58 Basic Tools 23:44 High Level approach 45:27 Actual Proof
How should money be created?
Переглядів 363Рік тому
I describe a new decentralized mechanism for creating money. My system has several advantages over the current system for creating money. Details are in this paper: arxiv.org/abs/2301.04244
Shor's Factoring algorithm and the Miller-Rabin Primality Test
Переглядів 4542 роки тому
I explain Shor's factoring algorithm and the Miller-Rabin algorithm for testing primality. Reference: kconrad.math.uconn.edu/blurbs/ugradnumthy/millerrabin.pdf 00:00 Intro 02:57 Group Theory review 04:58 Number Theory review 08:56 Hunting for witnesses 11:07 Shor witness 16:13 Miller-Rabin witness 18:44 Algorithms 20:58 Controlling number of non-witnesses
Bitcoin without jargon
Переглядів 1,9 тис.2 роки тому
I explain what Bitcoin is and how it works. 00:00 Introduction 02:23 If transactions are common knowledge, money is common knowledge 04:31 Digital signatures 05:36 Random leaders 08:51 Use computationally intensive task to pick leader
Fed up with inflation? Let's debank the financial system!
Переглядів 1,5 тис.2 роки тому
Fed up with inflation? Let's debank the financial system!
The Sunflower Lemma and Monotone Thresholds
Переглядів 7222 роки тому
The Sunflower Lemma and Monotone Thresholds
Reed-Muller codes achieve capacity on the erasure channel
Переглядів 6443 роки тому
Reed-Muller codes achieve capacity on the erasure channel
Must every large set have an arithmetic progression?
Переглядів 5403 роки тому
Must every large set have an arithmetic progression?
Using Chebyshev Polynomials to Prove Markov’s Theorem
Переглядів 9733 роки тому
Using Chebyshev Polynomials to Prove Markov’s Theorem





taking another run at this............Goedel’s indictment of the logic of mathematics, if that is the proper term, confuses me as per the following. That there is a formal system whose potential scope as it is at any given moment of consideration or as it might be/become in the wake of the addition of some new component would be incapable of proving all statements is common sense. But it relies, I think, on the assumption that the truths claimed to be known as unprovable would remain so. I think this ridiculous. In what is perhaps a departure from the form and function of traditional mathematical proofs, consider the simple logical necessity of the understandings of certain concepts. That 1+1 = 2 requires no proof for its truth is self-evident, notwithstanding Russell’s and Whiteheads Principia Mathematica, which required 379 pages for that purpose. In this statement, 1 is a term applied to the concept “a something by itself”. The term/numeral is merely a proxy for the concept and arbitrarily derived. It could be replaced with “paint can” and as long as all in witness of its presentation as a proxy for a mathematical concept were in agreement with the use of the name, it would function equally as well. 1+1 then is obviously and necessarily “a something by itself” and another “something by itself”, the understanding of the second term/something by itself a product of the same process of the awareness and understanding of the first as a distinct entity or concept. The + term of the proposition is merely an articulation of the concept of the joining of the two together in the same place or in the consideration of the second as another value, the same as the first. No proof required. The = sign is then a term applied to convey the introduction of a concept of what both “something’s by themselves” which is 2, a proxy for the two concepts once joined together. That the proposition 1+1=2 is logical is by definition true and were it not, the mind would be incapable of entertaining or understanding the concept of 1 at all, especially as distinct from the identical concept of 2, no matter what proof might be offered. Continuing on, the statement 2+2=4 is necessarily true and requires no proof by the extension of that above, i.e., that 2, already established as per the above, + another, the joined “somethings by themselves” as a single concept given a new label (4), a proxy for the joining of (2) 2’s. The continuing extension of this architecture would generate additional propositions also true by definition. That one cannot “appeal to truths to formulate a position which denies the existence of truth” (or claim that he “thinks he is not thinking”) is that to which a denial of that above would be akin. Context is also important. Consider a stone yard with two similarly sized piles of rocks of approximately the same dimensions, one granite and one limestone. One could logically call one pile “A” and the other “B” if the context of consideration were only that they were both to be understood as a piles of rocks and the general location of each within the stone yard. That “A” = “B” would be objectively true. However, were a stone mason then to come to the yard and ask for a pile of rocks but of granite, “A” could no longer be said to be equal to “B”. These calculations are also self-evident and require no proofs or calculations. Obviously, in such a manner of extension of proofs of these calculations, one could construct a very complex architecture of mathematical propositions, many if not all the supporting structure of others, none of which could be said to be true but without proof for the proof would be found in that stated above regardless of whether or not there were any axioms for that purpose. That a proposition can be defined initially at all is a product of logic or there could be no proposition defined and not means of calculating its aspects. This is the nature of epistemology and evident of the configuration of the mind itself. So, to reach out to the other end of all of this, one is compelled to ask if Goedel would have denied that above. Apparently he translated through his numbering system (which I don’t understand) a statement which stated that there were mathematical truths for which there were no proofs. This begs a few questions, one of which is that if one can claim that a statement is in fact true, he has to know how and why it is true (as per our necessary understanding of 1+1=2 or he could not make that claim) and that how and why “is” the proof. If, and I find it doubtful, that he was claiming instead that there are mathematical statements that could be made in the pursuit of previously unknown propositions for which as yet no formal process of generating their proofs had been completed, that is interesting but overall, meaningless for as per the epistemological process of that above, they would inevitably be found. I cannot find anyone who can tell me (I think because none knows himself) what would be the mathematical statements employed to have, prior to the process of translation, generated the statement “this statement cannot be proved”. Is it just more self-referencing nonsense like Quine’s liar paradox? How is it that a mathematical system which functions on strictures like those which confine the meaning of 1+1=2 to an unequivocal status, generate a self-cancelling statement like “this statement (is true but) has no proof”? Something is wrong with all this and would challenge anyone to explain how Goedel’s system generated this statement and that from which it was generated. I don’t think anyone can.
thank you for the amazing videos, I am really enjoying them. I'd be interested in your recommendations on where to learn the basics of finance as a physicist. Your explanations are always very clear and I'd love to find more that are as clear and precise as yours.
Thanks! I wish I had a good reference. For what it's worth, whenever I have talked to an actual economist or person in finance, they react as if my interpretation is insane. But they do not point to exactly where I got any of it wrong. As far as I can tell, they are operating in a reality that is built upon a very nested self-referential jargon, and their view makes complete sense after you have adopted that jargon.
@@anuprao11 Thanks, appreciate the response. Mirrors my difficulties in finding a good source :)
The Truth is whatever continuously replicates. A truth is an instance of The Truth; a fact or perspective.
14:08
1. If we consider the statement of Goedel’s that “this statement is true but cannot be proved” it can only mean; That the statement “this statement is true but cannot be proved” is itself the statement of issue (self-referencing). So, “if” “this statement is true but cannot be proved” is true as per its own definition/claim then the why and how would have to be known and discernable or all this makes no sense. I might be in error but I think that Goedel presented this “this statement is true but cannot be proved” via his translation of some mathematical statements by his number system as the calculated consequence of some “hole” in mathematics and logic, not a word puzzle/paradox. It is supposed to be a structural phenomenon of mathematics/logic. This would mean that the mathematical statement from which it was translated claimed to be true, (before translation) but could not be determined as such by means of any reasons as to how or why. This is illogical. • The problem is that the conclusion of his translation claims to be merely just that, a translation such that the mathematical statement from which the translation was the extension, had to be known to be true but also to have no proof before the translation was executed or it could not have been reflected in that translation and that makes no sense. Here we have the tail wagging the dog. • The problem is that this mathematical statement which when translated claimed that it was true, would have to have had some reason to be considered true, some structural reason which again, would mean that in reflection of that structure, we would have the how and why it was true which would be the proof. I am sorry but I do think that given the above, it is clear that this entire scheme is sophistry, i.e., that the logic is backward in the acceptance of this scheme. The logic by which his very propositions were defined is being denied in the manipulation of the components of the scheme that it might be asserted and accepted. would someone review this one last post and let me know what you think? I just think that if there is math to prove this, it must be in error or the semantic analogy would make sense and it decidedly does not. Thanks.
By true they just mean true in a larger system that we are using to talk about Meta Mathematics. Just ignore the "true" part as it is meaningless. Gödel's incompleteness theorem simply says that there are statements that cannot be proven even when I just used logical reasoning within the system I am talking about (i.e. without having to invoke metamathematical arguments to do it).
@@zemm9003 First, thank you for responding. I love these discussions and I really want to understand fully the topic but I have no math background due to having a sufficiently debilitating case of dyslexia so I could never study it as was typical when I was in school (a long time ago). Let me clarify my position….IF one formulates a proposition he can only do so by logic or it couldn’t possibly make any sense. The strictures of the structure of language would impose and make that clear. The logic by which a proposition is formulated, by which the conclusions are drawn and by which it is presented is that by which it should be critiqued. Is that not so? I would think that if this is not the case, the structure would not exist to have this discussion. Anyway, I have never seen a video on this topic that didn’t present it as a translation of a sort from mathematical language to what I think is the system of propositional calculus of B. Russell and Whitehead. Is that the case? If I am correct in this then I would think that my critique applies. When you make a statement in which a part is contingent upon another, i.e., (“this statement is true) but cannot be proved”, the latter contingent upon the former, the logic by which the dependent statement was defined has to also define the former. As stated in my post, the statement “this statement is true but cannot be proved” or “this statement cannot be proved” (as I have sometimes seen it) can only be either a self-reference, that statement referring only to itself, which I don’t think can be the case for as I understand it, Goedell translated the mathematical statement through his number system to the semantic we are discussing, or as I mentioned immediately above, it is a statement referring to some previous statement in mathematical language then translated. If the former, it makes no sense and why would he have required the translation scheme at all? If the latter, then the essence of my critique I would venture, still applies. If referring a previous statement in mathematical language, the claim of the dependent statement “…but cannot be proved” is dependent upon the former for its very existence. We are back then to consider, how and why is it asserted or assumed that the statement translated is true? Once again, if that is known or defined as such by the translation scheme then it can only be that the how and why it is true is known and again, that would be the proof. So I don’t get how this makes any sense at all and of what possible use it could be. Materiality does not permit contradictions, e.g., a rock cannot be both here and there at once. This applies to the realm of the abstract as well, itself a reflection of the material realm. All abstractions/concepts are a product of material contextual referents by which contradictions are also not permitted. Again, one cannot “appeal to truths to formulate a position which denies the existence of truth, i.e., one cannot claim “I think I am not thinking” and expect that it be considered valid. I don’t think that Goedell found a hole in math or logic but rather introduced one by the same sophistry employed in the paradox of Quine, whom Goedell apparently admired, something I cannot understand. Quine’s liar paradox, “this statement is false” was pure piffle. What do you think?
@@jamestagge3429 Gödel's Theorem is purely about syntax. So that he avoids pitfalls by purely computing what he is saying. Gödel's Theorem just means that there are some end strings (Theorems) that cannot be obtained from the starting strings (Axioms) by using inference rules to calculate what are the valid and "true" strings of the language and inside the Theory.
@@zemm9003 Thanks again for taking the time to respond. I guess what I don’t understand is the context from which all this arises. 1 + 1 = 2 is logical. Why? because 1, an arbitrary name applied to a concept which is unequivocally as to what it is, e.g., 1 is simply, logically a “thing by itself”. By virtue of the nature of our minds, whatever they are, when another “thing by itself” is observed, it is understood by the very structure of the mind as “another single thing by itself”, the second distinct from the first. With two “things by themselves, we apply the name 2 if we wish to refer to the two together so the + sign simply is a proxy name for “and” and = a proxy name for the act of their placement together. If this kind of thinking is extended out into more and more complex formulae, I don’t see the problem in there being ways to prove each and every such formula. What am I missing? That Goedell chose to translate these kinds of things into semantic analogies, certainly the means of the critique the conclusions of those translations would be by means of the brand of logic by which the semantic constructions were formulated, yes? Are these not reflective (as analogies) of the mathematics from which they were derived/translated? If not, what was the point of his scheme? If I am correct in this relationship between the original math and the consequent semantics, I would venture that to successfully critique (show the faults in) the conclusions of the theorem as expressed in language, the mathematics problem would be dispensed with by definition. How is it that this might not be true?
@@jamestagge3429 deducting valid formulas is not the same as deducting theorems. Valid sentences depend only on the definition of the language you want to use. Provable sentences depend on the language and on the axioms you want to use as a starting point (and inference rules). Not all valid sentences are theorems (even if they are good enough to be provable outside the system in a bigger system) This is what Gödel has shown. That you can always find new sentences that look like they should be Theorems (or their negation) but are actually completely independent from the Axioms and are thus undefined.
I know such truth‘s.
Thinking you know better than Gödel is height of stupidity & arrogance, after all this time nobody has been able to refute Gödel! 🤦♂️🤦♂️🤦♂️🤦♂️🤦♂️🤦♂️🤦♂️
Cagare loroto que hay que encima jigar al virre que ye preño co la vis. Podid
you cannot claim that there is a truth which has no proof for to assert such a thing, you would have to know "why" and "how" it was true and THAT WOULD BE THE PROOF. This is ridiculous. "This statement cannot be proved is as meaningless as Quine's liar paradox, "this statement is false". In both, the term statement is a set definition empty of members, i.e., no reference object. They are both piffle.
During the argument, we showed that there is a long list of statements such that most of the statements are true, and none of them can be proved. This established that there is a true statement that cannot be proved. So, we managed to give a proof that most of the statements are true. But we did not find a proof of a specific true statement in the list.
@@anuprao11 No, it doesn’t help to resolve the contradiction in Goedel’s reasoning (if the videos such as this one are to be considered correct), but I do thank you for your response. It is very instructive and makes this discussion all the more pleasurable. “If” you make the claim that there are these or those statements which are true then we are required to assume that these statements are in fact true (or there is little reason to have this discussion) and if so, we know that logically, you cannot make that claim unless “you know how and why they are true” that you might state them as such. To argue on the side of Goedel, i.e., this argument, you deny the very logic by which he formulated his proposition, he basically “appealing to truths to define a position which denies the existence of truth”. I don’t care how smart he was supposed to be, this is illogical, makes no sense and certainly does “not” show that there is a “hole in mathematics or logic”. Again, to claim that “there is a long list of statements such that most of the statements are true, and some of them can’t be proved”, one must explain how he could make the claim that they are in fact true. If he can explain how he knew them to be, THAT IS THE PROOF! This is piffle. Goedel employed structurally sound logic to formulate his propositions/theorems without which he could not have done so, i.e., if he could not have understood exactly how it was that they could be determined to be true (which is again the proof). One cannot make the claim that there are statements that cannot be proved (or “this statement cannot be proved) in the context of that above. The statement, “this statement cannot be proved” in terms of its meaning (even as a self-referencing statement) is in a sense, contingent upon the assumption that there is a logical structural necessity for the determination for certain statements to be considered true and could not then determine the lack of some statements, i.e., the logic by which the statements would be demonstrated to be unproveable would have to encompass the means to their truth. So this logical, structural necessity is the means to the proof of any true statement. I don’t see how Goedel could be considered having found a hole in logic, etc. What do you think?
If you do not find the explanation in this video convincing, then please point to the exact step of the argument made in this video that you do not find convincing, and we can talk about that.
@@anuprao11 Sounds good. I will watch in its entirety today. My comments are a result of many other videos on the same topic, none of which make sense to me. But let me leave you with this and tonight I will watch the video and comment, to which you can respond. In Quine’s liar paradox, “this statement is false”, which is obviously self-referencing, he is employing the worst kind of sophistry. I indict Goedel for he did the same. How could a man considered so brilliant think well of the liar paradox which is pure piffle and want to employ a similar self-referencing statement in his discipline? In Quine’s paradox, the term “statement” is not a subject noun like rabbit or rock, the two self-defined in a sense, but rather it is a set definition but in the paradox, one without any members, i.e., no reference object by which it violates the law of non-contradiction, it being required to be considered true and false at once. (The same violation of logic was employed in the Nelson-Gelling paradox). This is sophomoric in my view. The statement that they sky is green is false would be a valid statement formulated by uncorrupted logic. The paradox is not. So too with “this statement cannot be proved”. Also, I believe that both of these challenge the context in which set theory is discussed so the damage is twofold. In order to formulate a proposition as Goedel did, there must be a structure of logic within which this is accomplished. That structure imposes certain strictures and realities which cannot be ignored or the proposition cannot be defined initially (and it was, or we could not be discussing it). As I mentioned in my previous post, to make the claim that [there are true statements(which cannot be proved)] one would have to know why those statements which are claimed to exist but which cannot be proved are true and again, that is the proof. The logic by which that is understood as such, is the same by which the claim that “they cannot be proved” is made. The knowledge that they cannot be proved is contingent upon that by which they are known to be true, or vice versa. How can one know that this or that statement is true “and” that it cannot be proved, the latter of which would be dependent upon why and how he knows it is a true statement? One cannot have it both ways.
@@anuprao11 no response to my last post?
Maybe you people should read about first and second order logic, before stating how to theorem or the proof is wrong.
I just checked your channel and I can only say it's awesome! Non-trivial concepts in form of presentations, frequent uploads, it's the best kind. Keep the work!
Glad you like them!
Can you give a reference to all these different proofs of the theorem, list them out or tell us where we can find the listing of these proofs, please? I wish to read as many as I can. EDIT: At least, do you know any geometric/analytical proof that doesn't use any algebra or triangulation, but only smooth functions? I have come up with one and I wish to know if it is already written somewhere.
You can find notes here: kam.mff.cuni.cz/~matousek/akt.html Follow the link to chapter 2 for several proofs. There is a geometric proof, which is the first one that Matousek describes.
@@anuprao11 Thank you! Now I have read the proof you linked. I have spent a nice chunk of time trying to find the sketches of these other proofs but it was a torture for many reasons, anyway... I managed to access just enough proofs and I think my proof actually coincedes with yours or the one that uses Tucker's lemma. I didn't really follow any proof together with all details, but I feel like yours has the same spirit as the one via Tucker's lemma but is more direct maybe. Still, both proofs are presented relatively rigorously and are imemdiately jumping on the utilization of simplices (Borsuk's still does). My proof also runs through "odd mapping has odd degree" part, but all utilization of simplices is already spent on defining the degree (popular and well understood), so my intuitive proof can focus only on geometric ideas and geometric properties of the degree (geometry = smooth surfaces exclusively, no PL). I like to think that such approach is easier to grasp and understand compared to those which enjoy arbitrary constructions with simplices, but it only makes sense if we assume homological algebra or simplices are hard to understand in the first place and that the mapping degree is still easy to conceptualize. All "different" proofs of this theorem describe the same phenomenon anyway, only the language (algebra, combinatorics, geometry) differs. It is not some complicated and hard theorem after all... Maybe I will proceed with my wish to make a UA-cam video on my proof (or rather my perspective on the proof), regardless of the facts I mentioned earlier. Hopefully some other people share that geometric intuition with me and will appreciate it. I shared all this here even after I lost the reason to do it, so don't mind me please, I'm sorry for taking your time.
Fantastic! Yes, the proof is presented here is identical to the second proof of Tuckers lemma in the notes. I myself am not completely comfortable with simplicial homology, and I assumed most people who watch a video like this are not. That’s why I did not define degree or any of those concepts, in order to get to the proof as quickly as possible. If you wrote up another presentation of a proof, I at least would be interested in seeing it!
@@anuprao11 That sure is nice to hear, thank you! If I really make it later this year, I will let you know.
As it can’t be proven, the truth may be wrong .
great video!! 27:00 small note - the symbol ⊊ you used means "proper subset", so it took me a little time to realise you meant ⊈
Yes, I meant that it is not a subset!
12:18 😂
Let me suggest there are four way - theoretically, scientifically, religiously, or quadradically
My friend is giving this exact topic as a mini seminar to incoming bachelor students, what a coincidence :). Have you considered wether the repeated commutators solution for n nails is optimal? Edit: perhaps a better phrasing is concatenated commuters
Great question! What is the length of the shortest word in the free group with this property? If you want a short word you should double the number of nails in each step (rather than adding one nail at a time). That would give a word of length O(n^2) for n nails. For two nails I think it is clear that the commutator is optimal. That suggest some kind of inductive argument to prove that this is optimal, but I don’t see how to do it at the moment.
Here’s another question: what if you want the property that any k nails will hold the picture up, but k-1 nails will fail to do so. Can you design a solution?
@@anuprao11 Hmm so if we stick to concatenating the commuter it would grow exponentially, something like 4^n for n nails i would guess (maybe add a constant c to be safe). For 2 nails I agree the commuter is optimal. With some friends we thought of a way to (as you said) for n nails bound the growth to O(n^3) by rewriting the n in binary and isolating the 1's as examples of 2nails (perhaps this what you already thought of). So for example for 4 nails A,B,C,D and for loops a,b,c,d it would not be [[[[a,b],c],d] but [[a,b],[c,d]] which is smaller than the first word. For 5 = 101 this would be [[[a,b],[c,d]],e]. For your other question, wouldn't the concatenating commuters work, since if any loop turns trivial (by removing its associated nail) the whole expression disappears.
Nice! Yes that works. I guess that gives a solution of size (n choose k)^2 which is again exponentially big for k linear in n. Is there a polynomial sized solution?
@@anuprao11 Right so the idea I mentioned should indeed be in O(n^3) unless there is some faulty reasoning somewhere. I have a video where i recorded solving the above problem, its rather long but with the timestamps you should find an exact explanation of the idea above. I thought of typing it up on latex but classes just started again :X and we're still wondering if there's any better way than quadratic growth. If you have any ideas that would be wonderful ! edit 1:grammar edit 2: the bound is actually in O(n^3) not O(n^2) there was a missing term in the simplification.
Very cool. I got the idea, but it seems that the second example with two nails actually has an expression of a^{-1}ba^{-1}b^{-1}. So if you pull out b, we get a^{-2} actually.
You’re right! I guess it’s good to have some errors in the videos to make sure the audience is paying attention :).
@@anuprao11 It was indeed a good test of my understanding :)
Does this generalize to higher dimensions?
There are many generalizations (try googling it!). For example here’s one higher dimension version: www.cs.princeton.edu/~zdvir/papers/DvirHu14.pdf
Nice! If the set of points was allowed to be of infinite size, i can clearly see that the set of real points and integer points are able to “break” this theorem. And for some examples involving less chalk: - Any three parallel lines - y=0, x=0, (i, i), (-i, -i) for i > 0 and similar variations of this layout Any other interesting ones I miss?
Shapes A_i where boundary(A_j) is within boundary(A_k) for j<k for i in naturals
A nice feature is that the properties we are asking about are invariant under linear transformations. So, since the integers points work, the points of any lattice also work!
Great video! Just a couple of observations: First, the Lemma at 7:00 is true only if (a, b/a)=1; in particular, it's true when both a and b are square-free. Second, there's a typo at minute 19:22, there should be an N over the sum of the reciprocals of the squares, otherwise, the final answer should be N*pi^2/6.
Thanks for the comments! You're right about both errors!
Correct me if I'm wrong, but what if the black point is "between" the two red points?
Because every line has at least 3 points, there are always at least 3 red points! So at least two of them will be on the same side of the normal as the black point. The closer one to to the normal is always available to use as the green point.
I gave this channel a UA-cam "do not recommend". I suggest others do the same. His advice to "try to fill in the gaps yourself" invites temporary or indefinite surrender to the inviolable theories of an unchallengeable academic elite who, in another video, he wanted the viewers to believe had made, for him, a life-changing theory, a claim made all the more ridiculous when one looks closely at the theory itself and its now standard it seems, tortuous and unlikely pseudo-mathematical manoeuvres (Godel).
Your disappointment was misplaced. Did you notice that Godel's theory used a pseudo-mathematical gesture that cannot be simulated by any mathematical or physical process such as a computer program? He employed a self-identifying demonstrative gesture. Self reference, such as "This sentence..." (whether S is true or false), is not a physical or mathematical gesture, it cannot be represented in computing or by any combination of mathematical elements or semiotic indices - mathematical symbols do not "point" to sentences or places on a page! Which is "this sentence.."? Mathematics does not tell us this. The gesture relies on tradition and expectation, on phenomenalistic object behavior, not the physical object behavior of mathematics. Godel's idea, built on a trite "paradox", which in phenomenalism is no paradox at all, was a pig in a poke. Mathematician's don't make good philosophers, most of the time anyway.
It’s not so complicated! The statement I presented here is self-contained, crystal clear, unambiguous and does not require any further interpretation. There is no gesturing or pointing required.
What Gödel proved is that there is a sentence "G iff no proof of G exists (within the system)". He deliberately shifted away from semantics and made it into a syntactic problem. Precisely because he wanted to avoid the problem with involving semantics. His argument can easily be translated into computer functions. It is equivalent to saying that not all valid strings can be built using certain rules from the axiom strings and this can be simulated in a computer, it is equivalent to saying that given a sentence S I can try to create a valid sequence of strings that starts from the axiom and ends at S and Gödel's Theorem is roughly equivalent to saying that there is at least an S for which your search will never end.
@@zemm9003 Thanks for that. I wil make two devils advocate arguments against it. First, look at "G iff no proof of G exists (within the system)": irrespective of proof, we know what G is nonetheless. A proof of G, in any form, could not possibly be better or more certain than our knowing G. But didn't Godel suggest that there was a problem with knowing G? In which case, G would be an incoherence, whether presented as a proof, as a proposal, or in a proposal (such as a sentence). For example, it is an incoherence to say "we cannot know X", or "only I know X". X falls away. Also, I cannot envision any computer function that could be built around this observationn, an incoherence is an incoherence after all. Second, "Gödel's Theorem is roughly equivalent to saying that there is at least an S for which your search will never end." But isn't this a truism, plainly evident without proof? Because I note that Godel, in order to make such a claim, invokes an infinite, he assumes the necessity of the very thing that is an element of his conclusion. Let Godel make a proof without invoking an infinite.
I really like how you present the analysis for one ball first, and generalize to low-diameter decomposition later. You separate all the key ideas very nicely.
Thank you Thatchaphol! And thanks for your great work on these topics :).
Your video is so great! But I actually interpret Gödel's incompleteness theorem as good news for science. The theorem says that, for any fixed checker C, there is a statement S that C cannot prove. But given S, we can update C to C', which can prove S. For example, a human can learn, update their brain configuration from C to C', and give a good explanation for S. For another example, a mathematical foundation can update its foundation from C to C', which can derive S. My interpretation: There will never be a human with perfect knowledge or a final mathematical foundation that can prove everything. In fact, if there is one, progress is impossible in some sense as it is already perfect. With the incompleteness theorem, we actually know that, no matter how much we have learned, there will be infinitely many new things that we can learn. We can improve indefinitely. Please let me know if you think this interpretation is flawed.
Yes, maybe. But if there is a lesson that can help the human checker learn, then you could call that lesson the first part of the proof, and the theorem again applies. So if you think of the checker as the code of our brains when we are born, then there is a truth that cannot be proved no matter how much we are taught during our lives. Now the reality is that our brains are not a closed system with input and output… and our brains themselves change during our lives. So maybe we can think of the "program" as a simulator for the molecules inside our brain, and the initial configuration of that system is hardcoded in the program. Then during our entire lives, the program takes as input all interactions that our brain has with the outside world, and simulates the changes to the structure of the brain until the actual proof shows up, and then it simulates that too. The proof discussed in the video applies to this situation if the program is of finite length and all the input to it is of finite length. So, unless some step of these simulations cannot be carried out by programs, you have that there is a truth that we will not be able to verify!
Isn't the statement a proof, and a traditional proof is simply a duplicate of S in another form? Doesn't the statement prove the method of the proof? Which of them, statement or proof, has the honour or right to be considered as THE Proof?
The statement is not the proof of the method.Rather the rigorous method is the proof of the statement.
If there are two unprovable truths such that if you update the checker to be able to prove wither of them, the other becomes false, then the theorem is bad news again.
It was bad news for science because Godel said that it proved that the universe was not determined - against science which claimed it was determined. It's all crap of course, just a mathematician succumbing to a paradox and lessening his defeat by redefining it, as Godel did.
There is a truth that cannot be proved? I think you are reading too much into GIT. Fermat's Last Theorem is true, but it can't be proven in ZFC Set Theory.
I defined exactly what I meant by "cannot be proved" in the video! The result is much stronger than saying that a truth is not provable in ZFC.
@@anuprao11 There may well be truths the can't be proven. The statement sounds similar to Fitch's Paradox of Knowability. However, Gödel's Incompleteness Theorem doesn't say there are general "Truths" that can't be proven. He only says that higher order systems of logic (such has Peano Arithmetic), are incomplete. There is nothing GIT that says the sentence G can not be proven (or disproven) in some other system of logic (or even Math).
Sounds like you are talking about an older proof of GIT. The proof I explained is due to Chaitin. It is stronger.
@@anuprao11yeah, is more deep what you are saying
At 45:00 - 45:07 why do you say that the edges going from inside the ball to outside the ball under this potential function are the only ones that can have negative weights ?
This is because in the algorithm, we first use the ideas from the previous step to fix shift the potential function so that it works for all of the edges going in one direction (outside to inside). Then we are only left with negative edges in the other direction.
In 38:26 of the video, you mention phi(v) = min over u {zeta(u,v) + phi (u)} .....but if there is no incoming edge to v, then what's really zeta(u,v) and what is it's value ?
First there is a typo on this slide. The case is supposed to be that there are no incoming edges to v. Then we are worried only about the outgoing edges, and if phi(v) is set to be -min over u of zeta(v,u) - phi(u), that would be good enough. But there are no outgoing edges either, then set phi(v)=0 and that works, because v is an isolated vertex. In general, edges in one of the directions between two sets can be handled using a max or min. The most general bad case is case 3. The point is that if all the edges go in one direction, then shifting the potentials of one side far enough in the appropriate direction does the trick!
@@anuprao11 1) So if there's no incoming edge to v, phi(v) = -min(zeta(v,u) - phi(u)) over all u works 2) If there's no outgoing edge from v, phi(v) = min(zeta(u,v)+phi(u)) over all u works 3) In case there are partitions V1 and V2 with potentials phi1 and phi2 respectively with no edge from V1 to V2, following works: F(u) = phi2(u) if u is in V2 F(u) = phi1(u) +min(zeta(u2,u1)+phi2(u2)-phi1(u1)) for all u2 in V2 and u1 in V1 where (u2,u1) is an edge from V2 to V1 so that F(b)+zeta(b,a)-F(a)>=0 where b is in V2 and a in V1. So, in slide at 41:25 there's a typo in sign of min in phi1 prime ? There should be plus in front of min instead of minus ? Also, (2) is a special case of (3) where V1 is just one node and phi1 = 0 ? Is my understanding correct ?
en.wikipedia.org/wiki/Anomie
Hello @Anup Rao Very nice video. Can you please let me know the source of your animation
I used Omnigraffle to draw most of the pictures!
@@anuprao11 Thank you so much. You and your lectures are awesome
I’m glad you like them and I appreciate the support!
Wow. Before this video my brain hasn't been as dead as it is right now. However, I noticed that on 23:51 you are saying that using binomial coefficients we have shown that f(x) is bounded. It is written that |f(x)|<=O(x). But neither is true. Later in the prove, you only use that f(x)/x is bounded, which is true To be precise, you proved that x(log(2)-1)<=f(x)<=x(log(4)-1)
f(x) is O(x)… this means that f(x) is bounded by a linear function in x, as you yourself explained is true!
@@anuprao11 Oh, yes, sorry. I mixed it with little o notation. I also noticed some typos in the presentation, for example, on 29:00 in the second formula you are integrating with respect to t, however in one integral dz is written.
maybe instead of "is the economy good?" (broad, controversial) you could use the trade network to answer "is the economy robust to failures/disasters/corruption/monopolies/etc?" (scoped, less controversial) like, even if two trade networks have the exact same gdp, i would prefer the one that doesn't have the potential for catastrophic failure.
You're suggesting that I should steer *away* from controversy? Interesting approach...
Very interesting! I think it is wise on your part to focus on the descriptive value (which is a lot), and not try to derive any measure/policy from it. Economic reasoning is subtle. I would try talking to economists of the austrian school (but get to know Mises (and partly Hayek) theorem on the imposibility of economic calculation if you don't know it yet)
That is one of the lessons I learnt from talking to economists when I was doing this more actively. At first, I came up with more concrete metrics that I thought are reasonable, but over time I realized that the most significant idea here is that trade networks can be used to do "something". Determining what that "something" is requires significant additional work.
We actually know things that cannot be computed.
There are some things stronger than proof or knowledge. For example, pain. We don't "know" we are in pain, and our certainty is weak in the face of the experience itself.
@@JohnJones-tx6rt Can you elaborate please?
@@gokutarzan We can't know we are in pain. We can't check our certainty. We simply are in pain, stronger than certainty or doubt.
9:34 I found formula for Chebyshev polynomials by 1. deriving recurrence relation and solving it with exponential generating function 2. deriving ordinary differential equation and solving it with power series
Anyone who thinks "there is nothing in the world that can evade the simulation of a program" is painfully naive, for starters.
That’s not an accurate quote. What I said was that we do not know of any physical phenomenon that cannot be simulated by programs. If you disagree with that, I’d love to hear about a physical process that’s impossible to simulate with programs.
@@anuprao11 Then why did you speak it?
@@James-ll3jb it’s taken out of context.
@@anuprao11 Whatvis the context if not your own stated one of quote unquote THE WHOLE WORLD!
@@anuprao11plenty of black hole stuff , quantum physics cannot be accurately simulated. Only approximately guessed. 🤯🤦♂️🤦♂️🤦♂️🤦♂️🤦♂️
Science is mere description, and explains nothing, friend. Try Nietzsche.
Thanks a lot for making such an advanced topic really accessible! One question that I have is: is there a simple intuition for why sqrt(n) is the right factor? Outside from the proof details.
Yes: you can show that the bound is tight for the convex body that is the convex hull of the points {+1,-1}^n.
You are such a great speaker and educator. Thanks for making all of these talks available. Well done!
My pleasure!
There’s a slight subtlety in the tea leaves story (which I assume you’re using to abstract proof of work in nakamato consensus). When the leader who’s found his name in the leaves announces he is the leader he does this by proposing his block/transaction simultaneously. The details aren’t important but in a world where the leader just announces he is the leader, and then this message is broadcasted, and then they announce their proposal things get complicated with timing attacks. It’s why proof of stake protocols are so much more of a pain to describe compared to proof of work protocols. In proof of stake we need some specialised crypto to prevent the adversary from knowing the identity of the leader before seeing the block
This was a deligjtful presentation. Looking forward to engaging with your full library of videos.
"there is a truth that cannot be proven" is actually a consequence of the extra assumption that a statement must be true or false. What Gödels proofs really say is that (if you have arithmetic) you either have undecided or self-referential statements. To me, this is a proof that you always have statements that can't be assigned the value of true or false meaningfully.
There is no such assumption made here. The theorem says that there is a true statement that cannot be proved, not that there is a paradox that cannot be proved. The statement that we found is either true or false: it does not reference itself.
A statement cannot be said true unless it is proved.If you trust in the truthfulness then it is provable.
@@anuprao11 the theorem in the video is a proof by contradiction which demonstrates that C cannot have finite length. Its an uncomputable function, and so it has infinite kolmogorov complexity. There are no true, unprovable statements. Thats a gross misunderstanding of godel incompleteness perpetuated by model theorists. The godel statement is not true in every model, and so its not true in the theory. Its just unprovable.
@@samb443 The problem is that our brains only have finite size. All of the computational objects we can hope to build seem to have finite size. So, the theorem is very much relevant.
@@anuprao11 But the "proof" that "for all n, there is an x making S true" is non-constructive, and so non-computable. So the "truth" of the statement is ethereal at best in this computational context. This is where the extra assumption that cmilkau was talking about comes in. That proof uses LEM, "its not false therefore its true". The theorem is only relevant if you accept LEM, and if C(S,p) has finite length.
Great lecture! Thanks!
👍👍👍
How is program A shorter than L if A had to contain L to perform operations on its data? At the very least there is one comparison between two data variables x and L. Maybe you don't actually need to store L but only its log10(L) numbers of digits and when a comparison needs to be done you just perform arithmetic on **parts** of L's digits to conclusively verify that len(x) < L
We are not claiming that program A is shorter than L. We are claiming that program A is shorter than n. Also, the length of the program has nothing to do with x or L. The length of the program is the length of the code that describes the for loops etc. x and L are variables in the program, and their size is not relevant to the length of the program. Here n is fixed during the execution of the program, and n is hardcoded into the program using its digits. Hope that makes sense.
Eureka!!!!!!!!!I have uncovered the great secret and enigma of times past, how to solve a problem from the 2000th millennium, I am reporting "Rielmann's hypothesis" and I affirm with total truthfulness that the numbers 2, 19. 41 and many others... they are not primes, and twin primes do not exist, I managed to factor a giant number into prime numbers by factoring 04 prime numbers (2,279,269)(Two Million Two Hundred and Seventy-Nine Thousand and Two Hundred and sixty-nine) I found three prime numbers(3,532,343)(Three Million Five Hundred and Thirty-Two Thousand and Three Hundred and Forty-Three) I found three prime numbers, I say Factored into prime numbers)(4,709,233)(Four Million Seven Hundred and Nine One thousand two hundred and thirty-three) I found three prime numbers(7,262,011)(Seven Million Two Hundred and Sixty-Two Thousand and Eleven)I found three prime numbers(2,700,984,697)(Two Billion Seven Hundred Million Nine Hundred and Eighty-four Thousand and Six hundred and ninety-seven) I found 04 prime numbers (all factored only with prime numbers (5,310,765,049) (Five Billion Three Hundred and Ten Million Seven Hundred and Sixty-Five Thousand and Forty-Nine) I found 04 prime numbers (all factored only with numbers cousins from smallest to largest and from largest to smallest...so "The Rielmann Hypothesis has completely lost its force in the current era....... Mr Sidney Silva.
20:33 you claimed that it's enough to show the integral converges. I fail to see the connection 😢 If thm2 is wrong then this slice of the integration is finite. And?
Oh I get it. You want to show if the integral converges then the thm 2 is true. I get that.
Excelent video! keep up the good work (:
Thanks for the great introduction! For those who are interested in understanding an elementary proof of PNT (with basic knowledge in calculus but without any background in complex analysis), please see my video here ua-cam.com/video/JeF8Yf3DZEU/v-deo.htmlsi=e9jz2auZMjK7X1Je