
- 410
- 123 795
Combinatorics & Optimization University of Waterloo
Приєднався 9 лис 2016
AGT: Dimension of splines of degree two
Talk by Anne Schilling.
Splines are defined as piecewise polynomials on the faces of a polyhedral complex that agree on the intersections of two faces. Splines are used in approximation theory and numerical analysis, with applications in data interpolation, to create smooth curves in computer graphics and to find numerical solutions to partial differential equations. Gilbert, Tymoczko, and Viel generalized the classical splines combinatorially and algebraically: a generalized spline is a vertex labeling of a graph G by elements of the ring so that the difference between the labels of any two adjacent vertices lies in the ideal generated by the corresponding edge label. We study the generalized splines on the planar graphs whose edges are labeled by two-variable polynomials of the form (ax+by)^2.
Splines are defined as piecewise polynomials on the faces of a polyhedral complex that agree on the intersections of two faces. Splines are used in approximation theory and numerical analysis, with applications in data interpolation, to create smooth curves in computer graphics and to find numerical solutions to partial differential equations. Gilbert, Tymoczko, and Viel generalized the classical splines combinatorially and algebraically: a generalized spline is a vertex labeling of a graph G by elements of the ring so that the difference between the labels of any two adjacent vertices lies in the ideal generated by the corresponding edge label. We study the generalized splines on the planar graphs whose edges are labeled by two-variable polynomials of the form (ax+by)^2.
Переглядів: 27
Відео
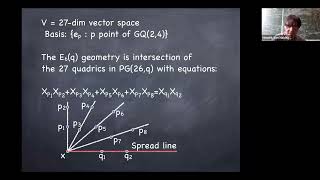
AGT: Geometric approach to some rank 3 graphs
Переглядів 3912 годин тому
Talk by Hendrik Van Maldeghem. Rank 3 graphs are graphs whose full automorphism group acts as a rank 3 group on the vertices. Finite rank 3 groups are classified and hence finite rank 3 graphs are classified. The main examples arise from geometric structures such as projective and polar spaces, and there is one class of examples related to the exceptional groups of type E6. We present a combina...
AGT: On the eigenvalues of the graphs D(5,q)
Переглядів 5212 годин тому
Talk by Himanshu Gupta. In 1995, Lazebnik and Ustimenko introduced the family of q-regular graphs D(k, q), which is defined for any positive integer k and prime power q. The connected components of the graph D(k, q) have provided the best-known general lower bound on the size of a graphfor any given order and girth to this day. Furthermore, Ustimenko conjectured that the second largest eigenval...
AGT: Thin distance-regular graphs with classical parameters $(D,q,q,\\frac{q^{t}-1}{q-1}-1)$ with ...
Переглядів 2012 годин тому
Talk by Xiaoye Liang. Title: Thin distance-regular graphs with classical parameters $(D,q,q,\frac{q^{t}-1}{q-1}-1)$ with t bigger than D are the Grassmann graphs. In the survey paper by Van Dam, Koolen and Tanaka (Distance-regular graphs, Electron. J. Comb., Dynamic Survey (2016), \#DS22), they asked to classify the thin $Q$-polynomial distance-regular graphs. In this talk, we will discuss our ...
AGT: Diagonal coefficients of Kirchhoff polynomials of 2k-regular graphs and the proof of the c_2...
Переглядів 3414 годин тому
Talk by Karen Yeats. Title: Diagonal coefficients of Kirchhoff polynomials of 2k-regular graphs and the proof of the c_2 completion conjecture. I have for many years been interested in graph invariants with the same symmetries as the Feynman period. Recently Erik Panzer found a new such invariant coming from a particular coefficient of the Martin polynomial. Together we used this to prove an ov...
AGT: Partial geometric designs, directed strongly regular graphs, and association schemes
Переглядів 3514 годин тому
Talk by Sung Song. A partial geometric design with parameters $(v, b, k, r; \alpha, \beta)$ is a tactical configuration $(P, \mathcal{B})$ (with $|P|=v$, $|\mathcal{B}|=b$, every point $p\in P$ belonging to $r$ blocks, and every block $B\in\mathcal{B}$ consisting of $k$ points) satisfying the property: \begin{quote}{for any pair $(p, B)\in P\times \mathcal{B}$, the number of flags $(q, C)$ with...
AGT: L-systems and the Lovász number
Переглядів 4214 годин тому
Talk by William Linz. For positive integers n and k, an L-system is a collection of k-uniform subsets of a set of size n whose pairwise intersection sizes all lie in in the set L. The maximum size of an L-system is equal to the independence number of a certain union of graphs in the Johnson scheme. The Lovász number is a semidefinite programming approximation of the independence number of a gra...
AGT: Quantum walk state transfer on a hypercube
Переглядів 6914 годин тому
Talk by Martin Stefanak. We investigate state transfer on a hypercube by means of a quantum walk where the sender and the receiver vertices are marked by weighted loops. First, we analyze search for a single marked vertex, which can be used for state transfer between arbitrary vertices by switching the weighted loop from the sender to the receiver after one run-time. Next, state transfer betwee...
AGT: Eigenvalues for stochastic matrices with a prescribed stationary distribution
Переглядів 5614 годин тому
Talk by Steve Kirkland. A square nonnegative matrix T is called stochastic if all of its row sums are equal to 1. Under mild conditions, it turns out that there is a positive row vector w^T (called the stationary distribution for T) whose entries sum to 1 such that the powers of T converge to the outer product of w^T with the all-ones vector. Further, the nature of that convergence is governed ...
AGT: On the diameter and zero forcing number of some graph classes in the Johnson, Grassmann...
Переглядів 3214 годин тому
Talk by Sjanne Zeijlemaker. Title: On the diameter and zero forcing number of some graph classes in the Johnson, Grassmann and Hamming association scheme. Graph classes in the Johnson, Grassmann and Hamming association scheme have received a considerable amount of attention over the last decades. Although several (NP-hard) graph parameters have been investigated for these families, many remain ...
25 Oct 2024 Tutte Subhadip Singha
Переглядів 69День тому
Tutte Colloquia 2025 Rotation-invariant web bases from hourglass plabic graphs and symmetrized six-vertex configurations
17 Oct 2024 Tutte Jessica Striker
Переглядів 13214 днів тому
Tutte Colloquia 2024 Rotation-invariant web bases from hourglass plabic graphs and symmetrized six-vertex configurations
04October2024 Tutte Purifying arbitrarily noisy quantum states Debbie Leung
Переглядів 66Місяць тому
Tutte Colloquia 2024
21 September 2024 Tutte A strongly polynomial algorithm for linear programs _Bento Natura
Переглядів 140Місяць тому
Tutte Colloquia 2024
September 13 2024 Tutte Sum of squares of positive eigenvalues_Thomás Jung Spier
Переглядів 118Місяць тому
Tutte Colloquia 2024
Tutte Colloquium - Vera Roshchina - 08/16/2024
Переглядів 1322 місяці тому
Tutte Colloquium - Vera Roshchina - 08/16/2024
Tutte Colloquium - Ryan O'Donnell - 08/02/2024
Переглядів 1542 місяці тому
Tutte Colloquium - Ryan O'Donnell - 08/02/2024
Tutte Colloquium - Bruno Lourenço - 08/09/2024
Переглядів 862 місяці тому
Tutte Colloquium - Bruno Lourenço - 08/09/2024
Tutte Colloquium - Carla Groenland - 07/26/2024
Переглядів 1,1 тис.3 місяці тому
Tutte Colloquium - Carla Groenland - 07/26/2024
Tutte Colloquium - Leonardo Colo- 07/05/2024
Переглядів 923 місяці тому
Tutte Colloquium - Leonardo Colo- 07/05/2024
Tutte Colloquium - Jason Gao- 06/28/2024
Переглядів 1113 місяці тому
Tutte Colloquium - Jason Gao- 06/28/2024
Tutte Colloquium - Patricia Klein- 07/12/2024
Переглядів 1053 місяці тому
Tutte Colloquium - Patricia Klein- 07/12/2024
Tutte Colloquium - Chaitanya Swamy- 06/14/2024
Переглядів 1513 місяці тому
Tutte Colloquium - Chaitanya Swamy- 06/14/2024
Tutte Colloquium - Paul Balduf - 06/21/2024
Переглядів 1603 місяці тому
Tutte Colloquium - Paul Balduf - 06/21/2024
Tutte Colloquium - Euiwoong Lee - 06/07/2024
Переглядів 1704 місяці тому
Tutte Colloquium - Euiwoong Lee - 06/07/2024
Special Seminar, Talk 2 - Vijay Vazirani - 05/30/2024
Переглядів 994 місяці тому
Special Seminar, Talk 2 - Vijay Vazirani - 05/30/2024
Special Seminar, Talk 1 - Vijay Vazirani - 05/29/2024
Переглядів 2054 місяці тому
Special Seminar, Talk 1 - Vijay Vazirani - 05/29/2024
Tutte Colloquium - Katya Scheinberg - 05/17/2024
Переглядів 1375 місяців тому
Tutte Colloquium - Katya Scheinberg - 05/17/2024
Tutte Colloquium - Akash Sengupta - 05/24/2024
Переглядів 2,1 тис.5 місяців тому
Tutte Colloquium - Akash Sengupta - 05/24/2024
Tutte Colloquium - Bertrand Guenin - 04/12/2024
Переглядів 2136 місяців тому
Tutte Colloquium - Bertrand Guenin - 04/12/2024







Is hadamard determinant problem proved for powers of 2?
Especially the point about density, imitating prime numbers and generating a sequence without consecutive points. Σε ευχαριστώ πολύ να είσαι πάντα ευλογημένη και πεφωτισμένη....
You deserve my gratitude. Presentation serves as a fruitful springboard to continue my research paper on independence proofs... you deserve praise and respect.
Proud of my tutor, Dr. Shirazi. From UoM
That's Downey bro, not you. Okay?
Can we get the PPT?
Where can I read more about association scheme?
You can't focus??????
Hi folks. For physics application and motivation, the thing to consider is that the kinds of numbers (multiple zeta values, special values of L functions, etc) appearing in the Feynman period roughly provides a bound on how complex the numbers can be for the same graph topology for any qft -- sometimes the extra structure of another qft could cause cancellations but it can't make the numbers more complex. This isn't anywhere near a rigorous theorem for a number of reasons (first proving much of anything about transcendentality of zeta values etc. is out of reach, second the underlying algebraic variety could have other periods and the physical one isn't proven generic in the right kind of way, and so on) but the physics reasoning should be that working with scalar field theory isn't about working with a toy qft, but is about separating out the part that comes just from the graph topology, and which is generic for that graph topology no matter the qft. As to the formal power series and rigour, the things we do in the paper are fully mathematically rigorous (unlike the paragraph above); you don't need a nonzero radius of convergence for these results. That's what the theory of formal power series is all about (of course when they do converge you get a bunch of extra analytic tools, yielding the field of analytic combinatorics). If you want to read more about that check out a text on enumerative combinatorics.
physics person here. i had to look up the paper; i was pretty instantly lost lol. i guess i would still appreciate more of a physics/computational perspective here. for physics, this looks to be some result for phi^4 theory. can it be extended to do useful things in a non-toy model quantum field theory? how difficult are these period computations without using this martin invariant? no offense, i think it's probably much nicer to mathematically to pretend phi^4 is physics, but i can almost guarantee that there are still more interesting math questions to be asked and answered in an actual (standard model) qft. combinatorics is also not often all that challenging for computers, so again even without the physics perspective, it would be nice to know what this does for people as a new computational tool/set of tools. congratulations on the result.
Grateful for the enlightening online discourse delivered by the esteemed Professor George Andrews. Thank you by Manish Kumar at BITS Hyderabad, India
You are the best
thanks for this great lesson
love it
Give an example for better understanding.
This is awesome.
Pls send me books to read more proximal method
Prof. Molloy did a great job explaining entropy compression in simple and concrete examples, comprehensible at undergrad and master's level. The talk really helps people understand the key ideas without worrying much about the details.
Kindly send me this webirPPT
Kindly send me this webirPPT
Proud to be Indian . Genius Sirinivasa Ramanujan.
Shouldn’t the Rogers-Ramanujan identities be named the “Rogers identities”? Considering LJ Rogers proved them independently in 1894 20 years before Ramanujan did?
Goated with the sauce
𝐩яⓞ𝓂𝓞Ş𝐦
Sir does matching polynomail exist for graphs with self loops ?
omg faffe
can you create one vidoe about quantum sort algorithms
Awesome!
nice tino 👍
Thank you for sharing!
Does anybody know about the billion size problems in finance? Any references? Mentioned at 49:33
Ok, i found out myself. One of the problems is trade compression in swap orderbooks.
Sir caan you please explain the theorem called All zeroes of the matching polynomial of all graphs are real valued.
Can we get this pdf file of presentation ? it is a humble request :)
The slides are availabe at homepages.dcc.ufmg.br/~gabriel/AGT/?page_id=226
omg,cool video, excellent speaker
Those videos are really helpful! Could you guys please post more related videos, tysm!
Sounds like my microphone cut out at the beginning - sorry, Abhinav! Luckily everything I said is on the first slide :)
GO August!!!!
WOW! the introduction was incredible!
Nattan der ehren mann
Hi mate! Liked the video, good job. Have you thought about using SMZeus . c o m to promote your videos!?
RIP graham :( :(
Thank you.
赞! Great job!
Very cool :)
Yo this is dope Rutger!!!