- 11
- 621
Gamma Seminar
Приєднався 24 бер 2023
The Geometry and Applications: Modern Mathematical Approaches (Gamma) Seminar is a weekly online seminar aimed at discussing basic and advanced research topics conducted by the Polish-Spanish geometric group organised by researchers from the University of Warsaw, the Universitat Rovira i Virgili and the Polytechnic University of Catalonia.
Topics:
- Geometric mechanics and field theories
- Geometric structures: symplectic and Poisson geometry, contact geometry and its generalisations
- Supergeometry
- Integrable systems, superposition rules, Lie systems
- Stochasticity and numerical methods
If you would like to stay informed about upcoming seminars and receive regular updates, please send an email to gamma-seminar(at)fuw.edu.pl with the subject line 'Subscribe to Seminar Mailing List,' and we will happily add you to our list of subscribers.
Visit our website (www.fuw.edu.pl/KMMF/gamma/) to access the links of previous sessions.
Topics:
- Geometric mechanics and field theories
- Geometric structures: symplectic and Poisson geometry, contact geometry and its generalisations
- Supergeometry
- Integrable systems, superposition rules, Lie systems
- Stochasticity and numerical methods
If you would like to stay informed about upcoming seminars and receive regular updates, please send an email to gamma-seminar(at)fuw.edu.pl with the subject line 'Subscribe to Seminar Mailing List,' and we will happily add you to our list of subscribers.
Visit our website (www.fuw.edu.pl/KMMF/gamma/) to access the links of previous sessions.
Silvia Vilariño --- k-symplectic geometry
The Newtonian formalism offers a very simple way to understand mechanical systems, but it has the difficult that it is necessary to measure and calculate the three components of the position and velocity of each particle that makes up the system.
Later, with the development of Lagrangian mechanics (1788) and Hamiltonian mechanics (1833) and their generalization to symplectic mechanics, the usefulness of the geometrical description of classical mechanics becomes clear. As we all know, this description of autonomous systems is carried out by means of the so-called symplectic manifolds.
When we make the leap from classical mechanics to classical field theory, the use of differential geometry has been a tool of great interest. At the end of the '60s and the beginning of the '70s of the past century, there are some attempts to develop a convenient geometric framework to study classical field theories. The first difficulty in this area is the generalization of the notion of symplectic form. There are different geometrical settings that allow describing classical field theory: k-symplectic formulation, k-cosymplectic formulation, multisymplectic, k-contact, etc.
The aim of this talk is to present the simplest one geometric description of classical field theories: the k-symplectic framework. The notion of k-symplectic manifolds will be introduced and these geometric structures will be analyzed. We will describe a Darboux' theorem for these structures and we will analyze certain types of submanifolds, for instance, k-symplectic orthogonal subspaces or Lagrangian of k-symplectic manifolds such as orthogonal subspaces or Lagrangian submanifolds.
Finally we will comment some interesting applications of the k-symplectic structures.
Later, with the development of Lagrangian mechanics (1788) and Hamiltonian mechanics (1833) and their generalization to symplectic mechanics, the usefulness of the geometrical description of classical mechanics becomes clear. As we all know, this description of autonomous systems is carried out by means of the so-called symplectic manifolds.
When we make the leap from classical mechanics to classical field theory, the use of differential geometry has been a tool of great interest. At the end of the '60s and the beginning of the '70s of the past century, there are some attempts to develop a convenient geometric framework to study classical field theories. The first difficulty in this area is the generalization of the notion of symplectic form. There are different geometrical settings that allow describing classical field theory: k-symplectic formulation, k-cosymplectic formulation, multisymplectic, k-contact, etc.
The aim of this talk is to present the simplest one geometric description of classical field theories: the k-symplectic framework. The notion of k-symplectic manifolds will be introduced and these geometric structures will be analyzed. We will describe a Darboux' theorem for these structures and we will analyze certain types of submanifolds, for instance, k-symplectic orthogonal subspaces or Lagrangian of k-symplectic manifolds such as orthogonal subspaces or Lagrangian submanifolds.
Finally we will comment some interesting applications of the k-symplectic structures.
Переглядів: 16
Відео
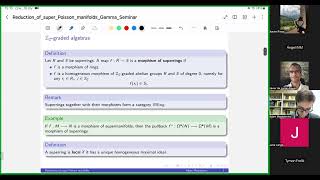
Adam Maskalaniec --- Reductions of super Poisson manifolds
Переглядів 3114 днів тому
Supergeometry is a branch of mathematics that arose from physical supersymmetric field theories. To describe fermionic degrees of freedom, supersymmetric field theories and supersymmetric quantum mechanics require the extension of differential geometry that includes both commuting and anticommuting variables. The symplectic and Poisson manifolds provide the framework for the geometric approach ...
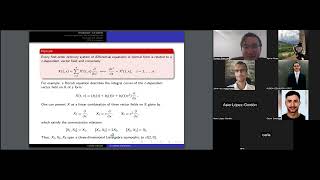
Tomasz Sobczak --- On k-contact Lie systems: theory and applications
Переглядів 3121 день тому
This talk serves as a continuation of the previous discussion on \(k\)-contact geometry and explores its application to the so-called Lie systems. These systems are a particular type of ordinary differential equations whose general solution can be expressed as a function of particular solutions and a set of constants, known as a superposition rule. Building on concepts from the previous talk, e...
Julia Lange --- Introduction to Courant algebroids
Переглядів 136Місяць тому
This talk is general introduction to the structure of Courant algebroids, which are a generalization of Lie algebroids, arising naturally in the study of Poisson geometry. These structures are defined by a vector bundle equipped with a skew-symmetric bracket, a non-degenerate symmetric pairing, and an anchor map, all satisfying a set of compatibility conditions. In this talk, I will introduce t...
Tymon Frelik --- Introduction to Cartan geometry
Переглядів 140Місяць тому
Élie Cartan's approach to differential geometry emerged as a continuation of Felix Klein's Erlangen program. The general idea of Cartan geometry is to describe curved analogues of Klein geometries, i.e., homogeneous spaces \(G/H\), where \(G\) is a Lie group and \(H\) its closed Lie subgroup. The merit of this approach is that it develops a suitable differential calculus for studying geometric ...
Leyli Mammadova --- Moment maps in multisymplectic geometry
Переглядів 31Місяць тому
In this talk, I will provide a gentle introduction to some topics in multisymplectic geometry, such as the L-infinity algebra of observables, homotopy moment maps, and weak homotopy moment maps. We will compare the two moment maps and investigate conditions under which the existence of a weak homotopy moment map implies the existence of a homotopy moment map. This part of the talk is based on j...
Bartosz M. Zawora --- On Marsden-Weinstein k-contact reductions with applications
Переглядів 462 місяці тому
In my talk, I will present a Marsden-Weinstein reduction theorem for \(k\)-contact manifolds. First, I will introduce the notion of \(k\)-symplectic manifold \((P,\boldsymbol{\omega})\), where \(\boldsymbol{\omega}\in\Omega^2(P,\mathbb{R}^k)\) is closed and satisfies \(\ker \boldsymbol\omega=0\). Then, I will briefly review the \(k\)-symplectic Marsden-Weinstein reduction theorem and introduce ...
Ana Bălibanu --- Reduction along strong Dirac maps
Переглядів 342 місяці тому
We develop a general procedure for reduction along strong Dirac maps, which are a broad generalization of Poisson moment maps. The reduction level in this setting is a submanifold of the target, and the symmetries are given by the action of a groupoid. When applied to quasi-Poisson moment maps, this framework produces new multiplicative versions of many Poisson varieties that are important to g...
Leonid Ryvkin --- Reduction of (multi)-symplectic observables
Переглядів 602 місяці тому
We develop a reduction scheme for the Lie-infinity-algebra of observables on a pre-multisymplectic manifold (M,\omega) in the presence of a compatible Lie algebra action \mathfrak{g}\curvearrowright M and subset N\subset M. This reduction relates to the geometric multisymplectic reduction recently proposed by Casey Blacker. In particular, when M is a symplectic manifold and N the level set of a...
Tomasz Sobczak --- New approaches to k-contact geometry II
Переглядів 593 місяці тому
Building on our previous discussion, I will introduce various extensions and new research topics in k-contact geometry. I will examine certain compact k-contact manifolds and explore a potential extension of the Weinstein conjecture to this setting. Additionally, I will present a new form of Hamilton-De Donder-Weyl equations for k-contact manifolds without polarization and describe a theory of ...
Javier de Lucas --- New approaches to k-contact geometry I
Переглядів 393 місяці тому
In the first part of this two-part series of talks, we will introduce k-contact geometry, an extension of contact geometry for studying field theories. I will begin by reviewing the classical method that employs k-contact structures, specifically through a reformulation called the k-contact form. We will also discuss a technical adjustment made to address a minor issue in the existing literatur...