Відео

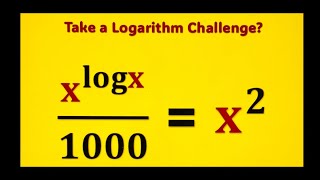
Solve (x^log(x))/1000 = x^2 where log() is the base 10 logarithm ... ... Take logarithm base 10 on both sides ... log( (x^log(x))/1000 ) = log(x^2) log( x^log(x) ) - log(1000) = 2*log(x) log(x) * log(x) - 3*log(10) = 2*log(x) ... substitute u = log(x) , and note log(10) = 1 ... u * u - 3 = 2*u u² - 2u - 3 = 0 (u - 3)(u + 1) = 0 u - 3 = 0 OR u + 1 = 0 u = 3 OR u = -1 log(x) = 3 OR log(x) = -1 x = 10^3 OR x = 10^(-1) x = 1000 OR x = 0.1 Check answers: x = 1000 : ( 1000^log(1000) ) / 1000 = 1000^2 ... note: log(1000) = 3 ... ( 1000^3 ) / 1000 = 1000^2 ( 1000^2 ) = 1000^2 ==> works out! x = 0.1 : ( (0.1)^log(0.1) )/1000 = (0.1)^2 ... note: log(0.1) = -1 ... ( (0.1)^(-1) )/1000 = (0.1)^2 ( (1/10)^(-1) )/1000 = (0.1)^2 ( 10 )/1000 = (0.1)^2 1/100 = (0.1)^2 1/100 = (0.01) ==> works out! x = 1000 OR x = 0.1 are both valid solutions.

_Answer_ : x = 153272¼ _Calculation_ : Let t₁ = ⁵√( √x + √(x+243) ) t₂ = ⁵√( √x - √(x+243) ) Note that for positive real values of x , we have t₁ > 0 > t₂ . Then t₁ + t₂ = 3 and t₁ * t₂ = ⁵√( √x + √(x+243) ) * ⁵√( √x - √(x+243) ) = = ⁵√[ (√x + √(x+243))*(√x - √(x+243)) ] = ⁵√[ (x - (x+243)) ] = ⁵√(-243) = ⁵√( (-3)⁵ ) = -3 So t₁ and t₂ are the roots of the polynomial equation t² - (t₁ + t₂)*t + (t₁ * t₂) = 0 t² - (3)*t + (-3) = 0 Solving for t: t² - 3*t = 3 t² - 2*(3/2)*t + (3/2)² = 3 + 9/4 (t - 3/2)² = 21/4 (t - 3/2) = ±(√21)/2 t = 3/2 ± (√21)/2 t = (3 ± √21)/2 t₁ = (3 + √21)/2 t₂ = (3 − √21)/2 Hence ⁵√( √x ± √(x+243) ) = (3 ± √21)/2 √x ± √(x+243) = [(3 ± √21)/2]⁵ √x ± √(x+243) = (3 ± √21)⁵ / 2⁵ ... binomial expansion on righthandside ... √x ± √(x+243) = ( 3⁵ ± 5(3⁴)(√21) + 10(3³)(√21)² ± 10(3²)(√21)³ + 5(3)(√21)⁴ ± (√21)⁵ ) / 2⁵ √x ± √(x+243) = ( 243 ± (405√21) + (5670) ± (1890√21) + (6615) ± (441√21) ) / 2⁵ √x ± √(x+243) = ( 12528 ± 2736√21 ) / 2⁵ √x ± √(x+243) = ( 783 ± 171√21 ) / 2 √x + √(x+243) = ( 783 + 171√21 ) / 2 √x − √(x+243) = ( 783 − 171√21 ) / 2 Adding both equations: 2√x = 2*( 783 ) / 2 2√x = 783 √x = 783/2 x = (783/2)² x = (800-17)²/2² x = (800² - 2*17*800 + 17²)/2² x = (640000 - 27200 + 289)/4 x = (613089)/4 x = 153272¼

👍👍😄

ua-cam.com/video/AMM6YHljHRQ/v-deo.html

Hello Everyone - Do watch other interesting videos by subscribing to my channel

A shorter way: write the equation as (x-4)^4= c^4*x^4, with c=-1, i or - i .Then you get x (1-c )=4 ,or x= 4/(1-c).= 2 ,2 (1+i) ,2 (1-i).

x^2=114^2-(64^2+50^2) x^2=(64+50)^2-(64^2+50^2) =64^2+50^2+2.64.50-64^2-50^2 x^2=(8^2)^2+(10.5)^2 ,a^2+b^2=(a+b)(-+)2ab x^2=8x8.5x5x2 x=8.5.2=80//

1:32 Why not just raise both sides to the mth power first off?

👋👋

I appreciate that

Wow!

А у меня х = 300 и равенство тоже правильное

√200=10√2=5×2√2=5(√2)³, 2=(√2)²=(√2)^(√2)², 2√200=((√2)^(√2)²) ^(5(√2)³)=((√2)⁵)^(√2)⁵

√(4 + √(24 + 4√20)) = √(4 + 2 + √20)) = √(6 + 2√5) = 1 + √5

Thumbnail shows a different expression, without the "+16" . 993*995*997*999 + 16 = = (996-3)*(996-1)*(996+1)*(996+3) + 16 = (996-3)*(996+1) * (996-1)*(996+3) + 16 = (996² - 2*996 - 3) * (996² + 2*996 - 3) + 16 = (996² - 3 - 2*996) * (996² - 3 + 2*996) + 16 = (996² - 3)² - (2*996)² + 16 = ((996²)² - 6*996² + 9) - (4 * 996²) + 16 = (996²)² - 10*996² + 25 = (996² - 5)² Therefore, √( 993*995*997*999 + 16 ) = = √( (996² - 5)² ) = 996² - 5 = (1000 - 4)² - 5 = (1000² - 2*4*1000 + 4²) - 5 = (1,000,000 - 8000 + 16) - 5 = 992,011

3ˣ - 3ʸ = 3 3ˣ⁺ʸ = 3 Substitute u = 3ˣ , v = 3ʸ . Note: since x and y are real, u and v must be real and positive. u - v = 3 uv = 3 u = 3/v ==> substitute into first equation 3/v - v = 3 3 - v² = 3v 3 = v² + 3v 3 + 9/4 = v² + 2(3/2)v + (3/2)² 21/4 = (v + 3/2)² v + 3/2 = ±√(21/4) v = -3/2 ± (1/2)√21 v = (-3 - √21)/2 OR v = (-3 + √21)/2 v = (-3 - √21)/2 < 0 ==> no real solution for (x,y) v = (-3 + √21)/2 > 0 ==> ... u - v = 3 ==> u = v+3 = v + 6/2 ... u = (3 + √21)/2 x = ln(u)/ln(3) = ln( (3 + √21)/2 )/ln(3) = [ ln(3 + √21) - ln(2) ]/ln(3) y = ln(v)/ln(3) = ln( (-3 + √21)/2 )/ln(3) = [ ln(-3 + √21) - ln(2) ]/ln(3)

log(√x) = 10^200 √x = 10^(10^200) x = 100^(10^200)

Just notice x>0 because of radical Steps: A.Raise to a power of 2 to simplify an equation. B.Introduce t = 25^2 C.it is nested radicals & can be to overwrite as t((^1/x)^1/x)=t or t^(1/x*1/x) = t or t(^1/x^2)=t. C.t is common of an equation so power of t's is equal 1/x^2=1 D.x^2=1 x=+/-1. So x=+1 is solution.

X,2×+5=8

Super easy but nice presentation.

Note that x = 5^(1/5) is not a solution to the infinite "power tower" equation x^x^x^x^x^... = 5 (You'd think that the "5" in the exponent on the lefthandside can be recursively subtituted by x^x^x^5 _ad infinitum_ ; but no, that's not valid.)

From the thumbnail: Just from inspection, x = 5^(1/5) = ⁵√5 ≈ 1.3797296615 appears to be a solution. (Not sure if it's the only solution, though.)

Thank you for explaining. I think there is another solution. It is (a, b)=(1, 18) . Actually, 18^(√1) - 1^(√18) = 18 - 1 = 17 .

√(9 + √(64 + 16√12)) = = √(9 + 4√(4 + √12)) = √(9 + 4√(4 + 2√3)) = √(9 + 4√( 3 + 1 + 2√3 )) = √(9 + 4√( (√3 + 1)² )) = √( 9 + 4(√3 + 1) ) = √( 9 + 4√3 + 4 ) = √( 13 + 4√3 ) = √( 13 + 2√12 ) = √( 12 + 1 + 2√12 ) = √( (√12 + 1)² ) = (√12 + 1) = 2√3 + 1 ≈ 4.46410

Answer: 49/111 Calculation: a³ + b³ = 10 [eq. 1] a + b = 7 [eq. 2] Find 1/a + 1/b . Use the identity a³ + b³ = (a+b)(a² - ab + b²) ... substitute using eq. 1 and 2 ... 10 = 7*(a² - ab + b²) a² - ab + b² = 10/7 [eq. 3] Furthermore, from eq. 2 : (a+b)² = 7² a² + 2ab + b² = 49 ... subtract eq. 3 ... (a² + 2ab + b²) - (a² - ab + b²) = 49 - 10/7 3ab = 343/7 - 10/7 3ab = 333/7 ab = 111/7 [eq. 4] Now, 1/a + 1/b = = b/(ab) + a/(ab) = (a+b)/(ab) ... substitute using eq. 2 and eq. 4 ... = 7 / (111/7) = 49/111

Solve for integers m and n , given ³√( 6√45 - 17 ) = m/(√n + 1) Lefthandside: ³√( 6√45 - 17 ) = = ³√( (48√45 - 136) / 8 ) = ³√( (45√45 + 3√45 - 135 - 1) / 2³ ) = ³√( (45√45 - 3*45 + 3√45 - 1) / 2³ ) = ³√( (√45 - 1)³ / 2³ ) = ³√( [ (√45 - 1)/2 ]³ ) = (√45 - 1)/2 Righthandside: m/(√n + 1) = ... multiply numerator and denominator by (√n - 1) ... = [ m*(√n - 1) ] / [ (√n + 1)*(√n - 1) ] = [ m*(√n - 1) ] / (n - 1) = (√n - 1) * m/(n-1) Equating LHS and RHS: (√45 - 1)/2 = (√n - 1) * m/(n-1) (√45 - 1) * 1/2 = (√n - 1) * m/(n-1) ... set n = 45 ... 1/2 = m/(45 - 1) 1/2 = m/44 m = 44*(1/2) = 22 ==> n = 45 , m = 22

⁴√(89 + 28√10) = = ⁴√(25 + 60 + 4 + 20√10 + 8√10) = ⁴√(25 + 20√10 + 60 + 8√10 + 4) = ⁴√(25 + 20(√5)(√2) + 60 + 8(√5)(√2) + 4) = ⁴√(25 + 4*5*(√5)(√2) + 6*10 + 4*2*(√5)(√2) + 4) = ⁴√(5² + 4*5*(√5)(√2) + 6*5*2 + 4*2*(√5)(√2) + 2²) ... Note: 5 = (√5)² , 2 = (√2)² ... = ⁴√( ((√5)²)² + 4*((√5)²)*(√5)(√2) + 6*((√5)²)*((√2)²) + 4*((√2)²)*(√5)(√2) + ((√2)²)²) = ⁴√( (√5)⁴ + 4*((√5)³)*(√2) + 6*((√5)²)*((√2)²) + 4*(√5)*((√2)³) + (√2)⁴ ) = ⁴√( (√5 + √2)⁴ ) = (√5 + √2) Alternative derivation: ⁴√(89 + 28√10) = = ⁴√( 49 + 40 + 2*14√10 ) = ⁴√( 49 + 2*7*2√10 + 40 ) = ⁴√( 7² + 2*7*2√10 + (2√10)² ) = ⁴√( (7 + 2√10)² ) ... Note: 7 + 2√10 > 0 ... = √( 7 + 2√10 ) = √( 5 + 2 + 2√10 ) = √( 5 + 2√10 + 2 ) = √( (√5)² + 2(√5)(√2) + (√2)² ) = √( (√5 + √2)² ) = (√5 + √2)

A different way: write the equation as a^4=c^4*(2^(3/2))^4 and c is a solution of c^4= -1 ,that is 1/√2 (± 1 ± i) , all 4 possible choices. Hence , a= c* 2^(3/2). We can now simplify : 2^(3/2) /√2 = 2 ,so that we have finally 2*(± 1± i) as final result.

Nice! :)

I use substitution method as it was easy. Answer is 4 and 9

perfect equations in your method

m = ᵐ√( 2^(√200) ) Assume m is real, and both sides are positive (note that for any real value of m, the righthandside cannot be non-positive anyway); then we can take the natural logarithm at both sides. ln(m) = ln( ᵐ√( 2^(√200) ) ) ln(m) = (1/m) * ln( 2^(√200) ) ln(m) = (1/m) * (√200) * ln( 2 ) m * ln(m) = (10√2) * ln(2) e^(ln(m)) * ln(m) = (10√2) * ln(2) Note that RHS here is positive ; hence there is one real (and positive) solution ln(m) = W₀( (10√2)*ln(2) ) , where W₀ is the 0'th branch of the Lambert W function. But we can derive a more convenient way to write the solution: e^(ln(m)) * ln(m) = (20√2) * (½) * ln(2) e^(ln(m)) * ln(m) = (20√2) * ln(√2) e^(ln(m)) * ln(m) = (4√2) * 5 * ln(√2) e^(ln(m)) * ln(m) = (4√2) * ln( (√2)⁵ ) e^(ln(m)) * ln(m) = (4√2) * ln( 4√2 ) e^(ln(m)) * ln(m) = e^(ln(4√2)) * ln(4√2) ln(m) = ln(4√2) m = 4√2

Thats a very neat one!

1 + 2√3

I think you're underrated Maybe you should change something ?

wow🤠 Never knew math's could be this easy!

👏

The claim that a^a = b^b is false in general. If you study the function f which maps x to x^x, you will see that it is first decreasing between 0 and e^(-1), then increasing afterwards. The conclusion is correct in our particular case, because f(x)<= 1 on the "non-injective" part [0, e^(-1)], whereas sqrt(32)>1.

Original et excellent !

👌

There is a different way: Take ln ,then you have ln m =1/m*ln a, with a= ln2*√200. If you set m= e^t then it follows that t* e^t = ln a ,which means that t = W[ln a] ,W = Lambert function. Hence m= e^(W[ln a] = 4*√2 .Of course , if the Lambert function is not known , then this solution cannot be found. Also ,the last step is not so easy to see.

This is from Albanian maths olympiade Proove that for any two arbitary numbers if you divide each of this numbers by their difference the remainder is the same but the quoation is 1 greater for example take numbers 4 and 3 3/(4-3) = 3 remainder 0 4(4-3) = 4 remainder 0

Wow - thats amazing!

oh this was pure beauty

Need more laws here. If line 2 to 3 is an equivalency , what happened between line 3 and line 4? Tom Foolery, me thinks. You made that up.

I lose you on the line that starts with 4*4th.

😮😮

How about proof? Using the method of mathematical induction for example

qui l 'eut cru !