
- 76
- 1 803
Nicole Kroeger
United States
Приєднався 24 чер 2014
Відео
12 Derivatives of Log Functions
Переглядів 69 годин тому
We learn how to take the derivatives of logarithmic functions and do several examples where we mix this with the chain, product, and quotient rules.
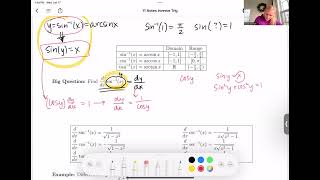
11 Inverse Trig Derivatives
Переглядів 2512 годин тому
Derivatives of inverse trig functions and some examples.
08 Implicit Differentiation Day 3
Переглядів 2316 годин тому
Implicit differentiation where x and y are functions of t
03 Chain Rule with Product and Quotient Rules
Переглядів 6День тому
03 Chain Rule with Product and Quotient Rules
04 Chain Rule within Chain Rule
Переглядів 4День тому
We do problems where we have to use the chain rule twice.
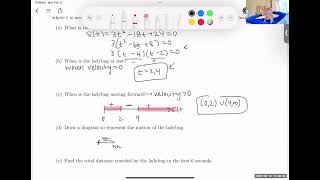
34 Curve Sketching
Переглядів 166Місяць тому
We do an example where we find intercepts, asymptotes, intervals of increasing/decreasing, max/mins, concavity, and inflection points. We then use all that information to sketch the graph of a function.
32.1 Concavity
Переглядів 35Місяць тому
What does it mean to be concave up/down and how do we use f'' to determine concavity? What are inflection points?
30 Increasing and Decreasing
Переглядів 12Місяць тому
We learn how to use the derivative to understand where a function is increasing and decreasing.
24 Derivative of Exponential Functions
Переглядів 40Місяць тому
24 Derivative of Exponential Functions
23 Washer Method with Other lines of Rotation
Переглядів 82 місяці тому
23 Washer Method with Other lines of Rotation







Great video, Nicole Kroeger. Looking forward to seeing your next upload from you. I smashed the thumbs up button on your content. Keep up the fantastic work! Your explanation of the quotient rule was very clear. How do you suggest we approach more complex functions where both product and quotient rules need to be applied simultaneously?
May you solve this one using the chain rule as well, f(t)=ye^(-at).
Yes, f(t)=e^(-at) can certainly be solved with the chain rule!
6:49 nuts