Відео

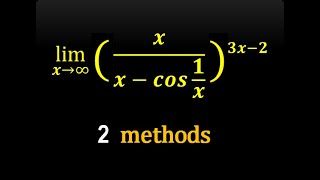
I found the same result, by using directly the fact that x^(1/x) is equal to e^(ln(x)/x), and that e^(ln(x)/x) - 1 is equivalent at ln(x)/x when x approaches infinity, since e^(x) - 1 is the same as x, when x approaches 0. Then I got (ln(x)/x)^(1/ln(x)), after that I introduced the exponential and ln functions ( a^(b) = e^(b×ln(a)) ), to finally get e^(-1).

Your math topics are very wonderful and we are very excited about how they will be solved, but your use of the paper and raising and lowering it interrupts our chain of thought and confuses us, so I hope that you use one space that accommodates the screen

Can I apply LH rule ( L'Hôpital ) 🙏

Another method over here could have been Binomial Expansion, that gives you the answer way quicker, in about 2 minutes if not less

But I have assumed, from the context, that P(x) is a polynomial.

It seems the limit as x->0 of x^x^...^x is 1 for an even number of x's and 0 for an odd number of x's.

(-3)^x If we keep x A= 2/2 Where (-3)^2 = 9 Then root 9 is 3 So x is 1

Since P(x+1) = P(x) + x, P(x) must be quadratic. Let P(x) = ax^2+bx+c. Again, P(1)=P(0) which means a+b+c = c which gives b=-a. So, P(x) = a(x^2-x) + c. Again, P(2) = P(1) + 1 = c+1. Thus, a(4-2) + c = 1+c which means 2a=1 or a = 1/2, So, P(x)=1/2(x^2-x) + c. I am assuming that P(x) is a polynomial. So, periodic functions are disallowed.

Just like i^2 = -1 similarly, this problem needs ln(-1) x = ln(3) / ln(-3) = ln(3) / (ln(3) + ln(-1)) but -1 = e^(i pi) so ln(-1) = i pi Therefore, x = ln(3) / (ln(3) + i pi) and also ln(3) / (ln(3) + i pi + i 2 pi n) [ since e^(i 2 pi n) = 1, so ln(-1) = i pi + i 2 pi n for any integer n ]

Thanks for an other video master

Solution: e^x e^x f(x) = ∫ln(t)*dt = ∫ln(t)*1*dt = 2 2 ------------------------------------ Solution by partial integration: Partial integration can be derived from the product rule of differential calculus. The product rule of differential calculus states: (u*v)’ = u’*v+u*v’ |-u’*v ⟹ (u*v)’-u’*v = u*v’ ⟹ u*v’ = (u*v)’-u’*v |∫() ⟹ ∫u*v’*dx = u*v-∫u’*v*dx ------------------------------------ e^x e^x e^x f(x) = [ln(t)*t]-∫1/t*t*dt = x*e^x-2*ln(2)-[t] = x*e^x-2*ln(2)-(e^x-2) 2 2 2 f(x) = x*e^x-e^x+2-2*ln(2) Now is: ln(2) ln(2) ∫x*f(x)*dx = ∫{x²*e^x-x*e^x+[2-2*ln(2)]*x}*dx = 0 0 (1) Solution of the indefinite integral ∫x*e^x*dx: ∫x*e^x*dx = ------------------------------------ Solution by partial integration: Partial integration can be derived from the product rule of differential calculus. The product rule of differential calculus states: (u*v)’ = u’*v+u*v’ |-u’*v ⟹ (u*v)’-u’*v = u*v’ ⟹ u*v’ = (u*v)’-u’*v |∫() ⟹ ∫u*v’*dx = u*v-∫u’*v*dx ------------------------------------ = x*e^x-∫e^x*dx = x*e^x-e^x+C = e^x*(x-1)+C (2) Solution of the indefinite integral ∫x²*e^x*dx: ∫x²*e^x*dx = ∫x*(x*e^x)*dx = ------------------------------------ Solution by partial integration: Partial integration can be derived from the product rule of differential calculus. The product rule of differential calculus states: (u*v)’ = u’*v+u*v’ |-u’*v ⟹ (u*v)’-u’*v = u*v’ ⟹ u*v’ = (u*v)’-u’*v |∫() ⟹ ∫u*v’*dx = u*v-∫u’*v*dx Look at (1): ------------------------------------ = x*(x*e^x-e^x)-∫(x*e^x-e^x)*dx = e^x*(x²-x)-(x*e^x-e^x-e^x)+C = e^x*(x²-x)-(x-2)*e^x+C = e^x*(x²-2x+2)+C Now is: ln(2) ln(2) ∫x*f(x)*dx = ∫{x²*e^x-x*e^x+[2-2*ln(2)]*x}*dx = 0 0 ln(2) = [e^x*(x²-2x+2)-e^x*(x-1)+[2-2*ln(2)]/2*x²] 0 ln(2) = [e^x*(x²-3x+3)+[1-ln(2)]*x²] 0 = 2*[ln²(2)-3*ln(2)+3]+[1-ln(2)]*ln²(2)-3 = 2*ln²(2)-6*ln(2)+6+ln²(2)-ln³(2)-3 = -ln³(2)+3*ln²(2)-6*ln(2)+3 ≈ -0,0505

Very cool

Thanks for an other video master

Thanks for an other video master

This is the Weierstrass substitution.

Why not let e raised to the power of -x equal sine theta?

Thanks for an other video master.

How did you factor a pi/2 on 2:33? I think you made too many mistakes

Also you may solve woth Feynman taknic

Wow, just now I realised you could use the d(cosx) notation for IBP 🤯🤯

Great video

Rewrite as \int{\frac{e^{x}}{1+\cos{x}} dx} + \int{\frac{e^{x}\sin{x}}{1+\cos{x}} dx} Integrate by parts \int{\frac{e^{x}\sin{x}}{1+\cos{x}} dx} with u = \frac{\sin{x}}{1+\cos{x}} , dv = e^{x}dx and combine integrals

I see integration by parts here with u = ln(x)

τ

an interesting integral

π ln (2) / 2

Using kings rule would solve it a lot quicker 🙌

Wow I would never have thought of it this way! I split it into integrals between -π~0 and 0~π and it turns into (∫[-π, 0] (x + |x|)sinx dx + ∫[-π, 0] (x + |x|)sinx dx), and now since x in the first integral is always negative, you can write |x| as -x, and the same logic allows you to write |x| as x in the second integral. So you end up with ∫[-π, 0] (x - x)sinx dx + ∫[-π, 0] (x + x)sinx dx which is ∫[-π, 0] 0 · sinx dx + ∫[-π, 0] 2x · sinx dx = 0 + 2 · ∫[-π, 0] sin dx = 0 + 2π = 2π

Your writing is so wonderful.❤❤❤❤❤

Solution: ∫1/(5^x+1)*dx = ∫(5^x+1-5^x)/(5^x+1)*dx = ∫(5^x+1)/(5^x+1)*dx-∫5^x/(5^x+1)*dx = ∫dx-∫5^x/(5^x+1)*dx = x-∫e^[x*ln(5)]/{e^[x*ln(5)]+1}*dx = x-1/ln(5)*∫e^[x*ln(5)]/{e^[x*ln(5)]+1}*ln(5)*dx --------------------- Substitution: u = e^[x*ln(5)]+1 du = ln(5)*e^[x*ln(5)] --------------------- = x-1/ln(5)*∫1/u*du = x-1/ln(5)*ln|u|+C = x-1/ln(5)*ln|e^[x*ln(5)]+1|+C = x-1/ln(5)*ln|5^x+1|+C Checking the result by deriving: [x-1/ln(5)*ln|5^x+1|+C]’ = 1-1/ln(5)*1/(e^[x*ln(5)]+1)*e^[x*ln(5)]*ln(5) = 1-5^x/(5^x+1) = (5^x+1-5^x)/(5^x+1) = 1/(5^x+1) everything okay!

I would split the fractions. First lets work on ploynomial on denominator X^4=-1 X^2=+-i X^2=i => x1=√2/2(1+i) x2=-√2/2(1+i) X^2=-i => x3=√2/2(1-i) X4=-√2/2(i-i). Now x1*x3=1/2(1+1)=1 and x1+x3=√2 X2x4=1 and x2+x4=-√2 So x^4+1=(x^2+√2x+1)(x^2-√2x+1) (Ax+B)/(X^2+√2x+1)+(Cx+D)/(X^2-√2x+1)=X^2/(x^4+1) (Ax+B)(X^2-√2x+1)+(Cx+D)(X^2+√2x+1)=X^2 X=√2/2(1+i) => (D+C*√2/2(i+1))*(i+1+i+1)=+i D(2+2i)+2√2C*i=i D=0 C=1/2√2 Similarly then A=0 B=-1/2√2 => X^2/(x^4+1)=√2/4(1/x^2-√2x+1)-1/(x^2+√2x+1))=√2/4(1/((x-√2/2)^2+(√2/2)^2)-1/((x+√2/2)^2+(√2/2)^2)) Now all that is left is using the identity Arctan'(x/a)=a/(x^2+a^2) I missed an x on nunerator so we need to first make the top similar to derivative of denominator first by afdind and subtracting a number which will resilt in logarithm antiderivative and then the arcatngent formula

a good part of mathematic

Just take divide numerator and denominator by 5^x. You get integral of dx/5^x(1+1/5^x) Then you set u=1/5^x, du=ln(1/5)/5^x. dx Substitute into the equation 1/ln(1/5) × integral of du/1+u. And the answer is ln(1+1/5^x)/ln(1/5)

an interesting integral

14‏/11‏/2024

an interesting integral

Buteful 🎉🎉🎉

an interesting integral

Thanks, if the integral had no upper and lower limits, how would it be solved, Professor?

Thanks for an other video master

Thanks

an interesting integral

an interesting integral

You amazing me with those internal half angles

an interesting integral

an interesting integral

Thanks for an other video master

an interesting integral

an interesting integral

I=int((xsec^2(x)+tan(x))/(1+xtan(x))^2)dx t=1+xtan(x) dt=(xsec^2(x)+tan(x))dx I=int(t^-2)dt I=-1/t+C I=-cos(x)/(cos(x)+xsin(x))+C