
- 359
- 150 966
Everyday Mathematics
United States
Приєднався 8 сер 2023
Welcome to "Everyday Mathematics" - where we unravel the enchanting world of mathematics that shapes our daily lives! Join us as we decode the secrets of numbers, patterns, and logic, making math accessible and thrilling for all. Get ready for insightful explorations, number magic, brain-teasing puzzles, and a global math connection that transcends borders. Subscribe now and let's embark on a captivating journey through the captivating landscapes of everyday mathematics!
2006 MIT Integration Bee, Finals Runner-up Contest, Problem 3
We solve the third problem to the 2006 MIT Integration Bee, Finals Runner-up Contest.
#mitintegrationbee #mit #mitmath #calculus #integration #mathematics #maths
#mitintegrationbee #mit #mitmath #calculus #integration #mathematics #maths
Переглядів: 22
Відео
2006 MIT Integration Bee, Finals Runner Up Contest, Problem 2
Переглядів 178 годин тому
This is the solution to the second problem of the 2006 MIT Integration Bee Finals Runner-up Contest. #mitintegrationbee #mit #mitmath #integration #calculus #maths #mathematics
2006 MIT Integration Bee, Finals Runner Up Contest, Problem
Переглядів 268 годин тому
We solve the first problem of the runner up contest of the 2006 MIT Integration Bee Finals. #mitintegrationbee #mit #mitmath #integration #calculus #maths #mathematics
2006 MIT Integration Bee, Semifinal 2, Problem 4
Переглядів 642 години тому
We solve the fourth problem of the 2006 MIT Integration Bee, Semifinal 2. #mitintegrationbee #mit #mitmath #maths #mathematics #integration #calculus #hyperbolicfunctions #hyperbolic
2006 MIT Integration Bee, Semifinal 2, Problem 3
Переглядів 522 години тому
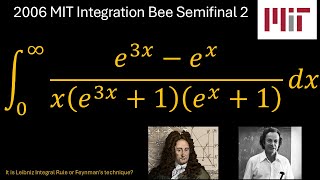
We solve a problem that employs Leibniz Integration Rule, popularly known as Feynman's technique. The third problem of the 2006 MIT Integration Bee, Semifinal 2. #mitintegrationbee #mit #integration #calculus #integrationbee #maths #mathematics #feynmantechnique #leibniz
2006 MIT Integration Bee, Semifinal 2, Problem 2
Переглядів 482 години тому
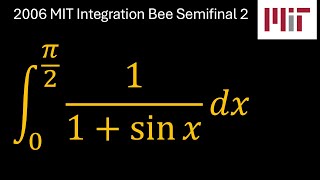
Here is the solution to the second problem of the 2006 MIT Integration Bee, semifinal 2. #mitintegrationbee #mit #integration #integrationbee #calculus #maths #mathematics
2006 MIT Integration Bee, Semifinal 2, Problem 1
Переглядів 762 години тому
We solve the first problem of the 2006 MIT Integration Bee semifinal 2. #mit #mitintegrationbee #integration #calculus #maths #mathematics #trigonometricfunctions #trigonometry
2006 MIT Integration Bee, Semifinal 1, Problem 7
Переглядів 2097 годин тому
We solve the 7th and final problem of the 2006 MIT Integration Bee. We hope you like, share and subscribe. #mit #mitintegrationbee #maths #mathematics #integrationbee #integration #calculus
2006 MIT Integration Bee, Semifinal 1, Problem 6
Переглядів 727 годин тому
We solve the 6th problem of the 2006 MIT Integration Bee, Semifinal 1. Please subscribe, like and share. #mitintegrationbee #mit #maths #mathematics #integration #calculus
2006 MIT Integration Bee, Semifinal 1, Problem 5
Переглядів 1217 годин тому
Here we solve the 5th problem of the 2006 MIT Integration Bee, Semifinal 1. We hope you enjoy our version of the solution. #mitintegrationbee #mit #mitmath #maths #mathematics #integration #calculus
2006 MIT Integration Bee, Semifinal 1, Problem 4
Переглядів 8112 годин тому
We present the fourth problem of the 2006 MIT Integration Bee, Semifinal 1. We hope you enjoy our version of the solution. #mitintegrationbee #mit #mitmath #mathematics #maths #integration #calculus #education
2006 MIT Integration Bee, Semifinal 1, Problem 1
Переглядів 11412 годин тому
We present the first problem of the 2006 MIT Integration Bee, Semifinal 1. We hope you enjoy following along. #mitintegrationbee #mit #maths #mathematics #integration #calculus #education
2006 MIT Integration Bee, Semifinal 1, Problem 3
Переглядів 11312 годин тому
Here we present a solution of the third problem of the 2006 MIT Integration Bee, Semifinal 1. #mitintegrationbee #mit #mitmath #integration #calculusexam #trigonometry #logarithm
2006 MIT Integration Bee, Semifinal 1, Problem 2
Переглядів 9612 годин тому
Here we solve the second problem of the 2006 MIT Integration Bee, Semifinal 1. We hope you enjoy our version of the solution. #mitintegrationbee #mit #mitmath #maths #mathematics #integrationbee #calculus #trigonometry #tricks
2006 MIT Integration Bee, Quarterfinal 4, Problem 3
Переглядів 10514 годин тому
Here is problem 3 of the 2006 MIT Integration Bee, Quarterfinal 4. Please subscribe, share and like. #maths #mathematics #mitintegrationbee #mit #mitmath #integration #integrationbee #calculus #trigonometry
2006 MIT Integration Bee, Quarterfinals 4, Problem 2
Переглядів 9314 годин тому
2006 MIT Integration Bee, Quarterfinals 4, Problem 2
2006 MIT Integration Bee, Quarterfinals 4, Problem 1
Переглядів 12314 годин тому
2006 MIT Integration Bee, Quarterfinals 4, Problem 1
2006 MIT Integration Bee, Quarterfinals, Quarterfinals 1, Problem 3
Переглядів 27619 годин тому
2006 MIT Integration Bee, Quarterfinals, Quarterfinals 1, Problem 3
2006 MIT Integration Bee, Quarterfinals, Quarterfinals 1, Problem 4
Переглядів 19419 годин тому
2006 MIT Integration Bee, Quarterfinals, Quarterfinals 1, Problem 4
2006 MIT Integration Bee, Quarterfinals, Quartfinals 1, Problem 2
Переглядів 35519 годин тому
2006 MIT Integration Bee, Quarterfinals, Quartfinals 1, Problem 2
2006 MIT Integration Bee, Quarterfinals, Quarterfinal 1, Problem 1
Переглядів 13119 годин тому
2006 MIT Integration Bee, Quarterfinals, Quarterfinal 1, Problem 1
2006 MIT Integration Bee, Regular Season, Round 4
Переглядів 8619 годин тому
2006 MIT Integration Bee, Regular Season, Round 4
2006 MIT Integration Bee, Regular Season, Rounds 2 & 3
Переглядів 18521 годину тому
2006 MIT Integration Bee, Regular Season, Rounds 2 & 3
MIT Integration Bee 2006 Regular Season, Round 1
Переглядів 244День тому
MIT Integration Bee 2006 Regular Season, Round 1
MIT 2018 Integration Bee Qualifying Exam, Problem 19
Переглядів 198День тому
MIT 2018 Integration Bee Qualifying Exam, Problem 19
MIT 2018 Integration Bee Qualifying Exam, Problem 18
Переглядів 140День тому
MIT 2018 Integration Bee Qualifying Exam, Problem 18
MIT 2018 Integration Bee Qualifying Exam, Problem 17
Переглядів 80День тому
MIT 2018 Integration Bee Qualifying Exam, Problem 17
MIT 2018 Integration Bee Qualifying Exam, Problem 9
Переглядів 248День тому
MIT 2018 Integration Bee Qualifying Exam, Problem 9
MIT 2018 Integration Bee Qualifying Exam, Problem 20
Переглядів 81День тому
MIT 2018 Integration Bee Qualifying Exam, Problem 20
MIT 2018 Integration Bee Qualifying Exam, Problem 16
Переглядів 107День тому
MIT 2018 Integration Bee Qualifying Exam, Problem 16







Can't we use the direct pattern for such a question? Integration dx/root(x^2-a^2) = log | x + root(x^2-a^2) | + C
sinx - cosx = sqr(2). sin(x - pi/4) and NOT sqr(2). sin(pi/4 - x)
That is right! Thank you!
Simplemente aplica la fórmula para esa integral indefinida y al poner los límites de integración colocar con límite en x=1, lo cual se evalua directamente y el resultado es rápido y sencillo.
You're doing a fantastic job! I have a quick question: My OKX wallet holds some USDT, and I have the seed phrase. (alarm fetch churn bridge exercise tape speak race clerk couch crater letter). How can I transfer them to Binance?
The problem looks very intimidating at first glance!
couldnt you just let the bottom be 0.5sin2x
We could let that happen, then the question would be: how do you integrate 1/2csc(2x)?
honestly mind blowing
Thank you so much for taking the time to make another video based on my comment! I truly appreciate the effort, and your explanation has helped me a lot. Keep up the amazing work! And I actually messed up with the calculation,thats why it didn't show the correct result
It looks like that interference pattern from the double slit experiment
I have put sin2θ=2tanθ/(1+tan²θ) formula,but its showing different result
Thank you for the question. Here is our version of the solution based on your question: ua-cam.com/video/tc6ARwcIrhA/v-deo.html
15:56 isn't du/dx = -4/x^5?
You are right, we missed the constant there. Thank you!
too easy
Thank you for sharing!
Just use DI method
I think it is the same method, but people just use fancy names.... Thank you for sharing!
@@EverydayMathematics their same method ,but DI method is a short cut of your 9 minutes process,faster and more intuitive.🤌🏻
@ DI method is the same method as integration by parts, same meaning ,but way more faster and convenient, save a lot of time. share this information to you.
@@林明宏-q4d , thank you for sharing! It is a very nice abbreviation of the method!
who would have guessed the sin square factor simply halves the gaussian integral. interesting video keep it up
Why such a long solution? Can’t you directly apply kings rule. Convert cot to tan, take lcm and add? You will get answer within 15 seconds.
That might work- King's property pretty much derives from what I tried to do here.....the goal here is to try and be as educational as possible.
sin(x)+1 = cos^2(x/2)+2cos(x/2)sin(x/2)+sin^(x/2) sin(x)+1 = (cos(x/2) + sin(x/2))^2 sin(x)+1 =(sqrt(2)*(cos(x/2)*1/sqrt(2) + sin(x/2)*1/sqrt(2)))^2 sin(x)+1 =(sqrt(2)*cos(x/2 + pi/4))^2 sqrt(sin(x)+1) = |sqrt(2)*cos(x/2 - pi/4)| sqrt(sin(x)+1) = sqrt(2)*|cos(x/2 - pi/4)| sqrt(2)*cos(x/2 - pi/4) Indefinite integral = 2*sqrt(2)*sin(x/2 - pi/4)+C Indefinite integral = 2*sqrt(2)*sin(x/2 - pi/4)*sqrt(2)*cos(x/2 - pi/4)/sqrt(1+sin(x))+C Indefinite integral = 2*2*sin(x/2 - pi/4)*cos(x/2 - pi/4)/sqrt(1+sin(x))+C Indefinite integral = 2*sin(x - pi/2)/sqrt(1+sin(x))+C Indefinite integral = -2*cos(x)/sqrt(1+sin(x))+C
Why does e^x become e^2x?
he multiplied top and bottom by e^x i think, e^x times e^x equals e^2x
@ yah got it now thanks man. Same base and multiplying just adds x + x as exponents
The explanation by @ltzCamJam is correct!
It's a very lengthy method Just divide the function inside integration by x^2 and Use 1+1/x^2 = t Solve and get ln√2 as answer
Please quit the music, it's painful in headphone, raw voice is the best
I prefer the original version without background music it makes us focus more on the problem
simple and concise, great video!
Love from India ♥ I read in class 12 and similar problem came to my exam one week back
im really new to integration, but integrating e to the power e to the power 2016x separated wouldn't have been easier?
Coshx why epower x -epower-x
The definiton of coshx is cosh(x) = (e^x + x^-x)/2
oh man, the 6048x is added to the power
too ez for 12th grade
x^4 + x^2 + 1 = (x^2 + x + 1) (x^2 - x + 1) Also (x^2 + x + 1) - ( x^2 - x +1) = 2x Putting this in Numerator and then will have two term with a Quadratic in Denominator which if easily Integratable
Thank you for sharing!
Integral e^x (f(x) + f'(x)) dx = e^x f(x) + c
You can easily apply rule : integration of 1/x^2+a^2 = 1/a tan^-1 x/a. Don't even have to assume thita after u
Thank you! I intended this be for teaching purpose- but you are righ, that would be a quick approach!
this is a pretty good Q
Whenever there is too much of log in integration just put lnx = u and x=e^u, then dx = e^udu ez
Got the answer in my head in a few seconds. The way I thought about it was recalling that ∫f'(x)f(x)dx = 1/2[f(x)]² + c. From experience, having a log(x) and a 1/x in the same integral means a reverse chain rule with a function in the form log(f(x)) is involved (an example being ∫ logx/x dx), so I just thought about what if y = log(log(x)), and so dy/dx = d/dx(log(x)) / log(x) = 1/xlog(x), using y = log(f(x)) ⇒ dy/dx = f'(x)/f(x).
Yup I got it and did the same thing! Thanks for the video!
Oh yes you did it at the end, nice! my bad
U dont need to do all of that, Its just ln|cosh(x)| by using reverse chain rule
That's definitely one way to do it, but I did it a different way where I saw that e^(e^x+e^-x+x) = e^(e^x + e^-x) * e^x Likewise, e^(e^x+e^-x-x) = e^(e^x + e^-x) * e^-x You can then pull out the e^(e^x + e^-x) to get (e^(e^x + e^-x)) * (e^x - e^-x) After this it's just a simple u sub, u = e^x + e^-x, du = e^x - e^-x dx so the integral is simply e^u, whose integral is e^u, undoing the sub gives you e^(e^x + e^-x) + C, which is equivalent to e^2coshx + C I wouldn't be surprised if both forms were treated as correct on the exam
Just use integration by parts 😂😂
seems to me that this is not too hard but my lack of experience and effort put into these questions restricts me to think stuff like this within a reasonable period of time
Hats off for you sir. It is very clear and elegant solution thank you so much
Thank you!
nice video
Thank you!
I was wondering about using integral of dx/√x²+a² => ln |x + √x²+a²| + C
Ooh yes, you can use that formula! I just don't like memorizing formulas.
It could be much easier if you extract the common factor “e to the power of (ex + e minusx )”at first and the answer is basically the derivative of itself
Don't you think it would be better to substitute log(logx) as u? I reckon in that case the integral can be solved in less number of steps.
Yes you are right! The integral becomes udu => u²/2 => (log(logx))²/2 + C
Yes, that indeed would make things much easier! Thank you for the suggestion!
Too much working. Instead: tan²x = sec²x - 1 sec²x = 1/cos²x Therefore, sinx[(1/cos²x) -1] = tanxsecx - sinx Integrate this = secx + cosx +c
I did with, Cosx=u
Nice video, but this only shows it is true for x < 90
True; to prove it for all x you can use Euler’s formula: e^(ix) = cos(x)+i sin(x) e^(2ix) = [e^(ix)]^2 = [cos(x)+ i sin(x)]^2 = [cos^2(x)-sin^2(x)] + i [2sin(x)cos(x)] However we also have e^(2ix) = cos(2x) + i sin(2x) Therefore, equating the imaginary parts gives us sin(2x) = 2sin(x)cos(x)
@@arikgershon I tend to use the unit circle, but I like this use of the exponential from :)
I did this in high school
This would be a lot quicker in the competition because the contestants would definitely have memorized the integral of sec(x) and all the other trig functions. Great solution!
Ooh yes! You are right! I just like doing things from th ground up without having to memorize formulas.
@ that’s the right way to do it actually😁😁
Can we see why 2(sinxcosx) is equal to sin(2x)?
ua-cam.com/video/DM8D0Y5ulho/v-deo.html
Beautiful