
- 80
- 26 269
Ginger Math
Приєднався 1 січ 2024
Yes - fun math with a ginger! Mostly all forms of calculus, complex analysis, analytic number theory, and diff eq, with a bit of physics sprinkled on top on Phridays.
Special thanks to Maria for the awesome banner!!
Special thanks to Maria for the awesome banner!!
A Sigma Proof (Complex Analysis)
Next video: Ginger Apologizes for Inappropriate use of "Sigma"
(0:00) Intro and f Assumptions
(1:35) Integer Residues
(4:15) Contour Integration
(7:35) Upper Bound of |cot|
(13:12) Yes, f goes to 0
(14:27) We did it!!
(0:00) Intro and f Assumptions
(1:35) Integer Residues
(4:15) Contour Integration
(7:35) Upper Bound of |cot|
(13:12) Yes, f goes to 0
(14:27) We did it!!
Переглядів: 89
Відео
Literally. Logs. (MIT Integration Bee)
Переглядів 16114 днів тому
Semifinal #2 Problem 2: math.mit.edu/~yyao1/pdf/2024_semifinal.pdf
Dew You Know the Residues of the Gamma Function?
Переглядів 6614 днів тому
More complex analysis to come :)
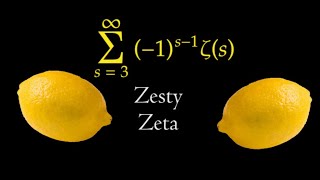
Zeta-ing a Zesty Sum
Переглядів 150Місяць тому
Original Post! math.stackexchange.com/questions/4985650/show-zeta2-1-sum-s-3-infty-1s1-zetas
Complex Analysis-ing a STUPENDOUS @maths_505 Integral
Переглядів 374Місяць тому
Check out @maths_505 original video! ua-cam.com/video/9DV0R5W9feA/v-deo.htmlsi=3xY4UHaRXx151yKi More complex analysis in the works as well :) (0:00) Introoooo (0:30) Integrals Around the Contour (12:52) Complex Analysis (mini edition) (20:53) RESIDUES
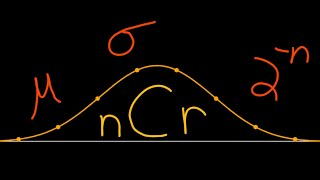
What's the MEANing of This...? (nCr Mean & Stdev)
Переглядів 62Місяць тому
Project is still going... lots of fun though with the Galton Board :) Here's that identity as well: en.wikipedia.org/wiki/Combination#Number_of_k-combinations
Besting a Berkeley Integral (Complex Analysis-ing)
Переглядів 259Місяць тому
Also check out Maths505's take: ua-cam.com/video/YJ6j1DXS4AU/v-deo.htmlsi=OiXFRpL5jeXQ5DrR
You Spin Me Like a Squircle Baby (#PhysicsPhriday 9)
Переглядів 81Місяць тому
You spin me right round baby right round like a [squircle] baby right round round round...
e-ATING Up a Sum
Переглядів 76Місяць тому
Yummmmm... en.wikipedia.org/wiki/Bell_number oeis.org/A000110 (0:00) Intro Short Life Update (0:40) Sum-ing (9:27) Bell Numbers Induction
Ginger Tries His Hand at Environmental Science
Переглядів 58Місяць тому
Ecosystem Services Project for AP Environmental Science! Another math video on the way :)
Raising the Roof with Ceiling Dion
Переглядів 7152 місяці тому
My heart will go ooooonnnn... Credit to Noemi for the title :) Quarterfinal #3, Problem 2: math.mit.edu/~yyao1/pdf/2022_quarterfinal.pdf (0:00) Video Bit (13:30) Blooper
The Beta-Zeta Combo
Переглядів 2902 місяці тому
This has been one of my favorite videos yet!! :) Lower Incomplete Gamma Function Bit: ua-cam.com/video/NO3EaJBiPaw/v-deo.html Dirichlet Beta function: en.wikipedia.org/wiki/Dirichlet_beta_function Yes, I swear this works: www.desmos.com/calculator/y605ozegsq (0:00) Intro (0:22) Expansion (4:24) Lower Incomplete Gamma Function (6:37) Unit Circle Bits (8:05) Sum More Summing (12:34) Sum #1 (Zeta)...
Half a Gamma?! - The Incomplete Gamma Function
Переглядів 1602 місяці тому
GONE WRONG?? BLACK HOLE CREATED???? Ft. Mr Beast?? Nah, just kidding - wanted to add that for a friend for funsies :) Anyways, here's the Wolfram MathWorld page: mathworld.wolfram.com/IncompleteGammaFunction.html
Glasser-ing a Great Integral
Переглядів 2072 місяці тому
Sources, resources, and recourses: en.wikipedia.org/wiki/Glasser's_master_theorem pC_UHgJjyVDY/
A Hot Potato Weighs more than a Cold One?! #PhysicsPhriday 8
Переглядів 512 місяці тому
A Hot Potato Weighs more than a Cold One?! #PhysicsPhriday 8
Looting a Log Integral with Complex Analysis
Переглядів 1493 місяці тому
Looting a Log Integral with Complex Analysis
Speedrunning Complex Analysis-ing a NUTS @maths_505 Integral
Переглядів 1353 місяці тому
Speedrunning Complex Analysis-ing a NUTS @maths_505 Integral
The Kth Dimensional Squircle - Squircles FINALE!
Переглядів 1113 місяці тому
The Kth Dimensional Squircle - Squircles FINALE!
Great EXPectations - Laplace Transforms
Переглядів 1,3 тис.4 місяці тому
Great EXPectations - Laplace Transforms
Greek Letter Tier List - 250 SUB SPECIAL
Переглядів 634 місяці тому
Greek Letter Tier List - 250 SUB SPECIAL
Complex Analysis-ing a CRAZY Integral
Переглядів 4034 місяці тому
Complex Analysis-ing a CRAZY Integral
REALizing it's Complex - Integration Two Ways
Переглядів 2754 місяці тому
REALizing it's Complex - Integration Two Ways







Thank you for this video. If you would like to calculate the x value corresponding to a 95% confidence interval with df > 2, how can you apply the approximate function? It will be exciting if I can watch an example in your new video!
🌲🌳 log
Naturally
freaky sonic video next
ur bald fans (me) were anxiously awaiting this video
One can make life simpler by choosing b as the Feynman parameter. dI/db = 2b \int^{\infty}_{-\infty} dx i/(x^2+a^2)(x^2+b^2) = 2\pi /a 1/(b+a) by contour integration. Then I =( 2\pi/a )ln(b+a) + c. Setting b=0, we get J=\int^{\infty}_{-\infty} dx ln(x^2)/(x^2+a^2) = (2/pi /a )ln a . Thus, c = 0. So, I = ( 2\pi/a )ln(b+a).
zesty
can you explain eulers identity
integrating for funsies?
Always for funsies :) But that would be a great title - Fourier for Funsies... ok yeah that's going to be an upcoming video (and an excuse to do Fourier things (if there needs to be a reason in the first place :) ))
ginger math biology takeover when?
You tell me >:|
this is so neat i love it when people do math neat
I think I’m in the wrong classroom
You ate and left no crumbs
ginger what about cultural ecosystem services :(
I'm sure there will be a Part 2 at some point :)
deforestation bad :( tree good :)
love this man
Imagine taking APES
I can barely hear you
hi! i'm so so confused, do you mind explaining the concept of squircles for a beginner in math to then explain deriving the volume formula. please😭
ceil(x)-x=1-{x} 0<={x}<1, so the geometric series for 1/(1-{x}) converges. The problem, then, is finding the ceiling of the sum, because if {x} is something like .999 the ceiling will be much higher than when it's something smaller like .6
ISABELLA GOT A LOLLIPOP!!!!!!
YES SHE DID! I should include bloopers like that more often
I understand every bit of this!
AWESOME - wanna share it with some friends then? :D
@@GingerMath Already did😂
I always thought those floors and ceilings were so hard.
Ditto! But if you split them up like this they're (hopefully) manageable
Nice video! Are you not forgetting a (-1)^m in the final solution due to the i^2m?
Yes I am... whoops - thanks for pointing that out lol
Hi 🙂Maybe at 16:41 you should add (-1)^m
4:45 Missed a t multiplying the second log.
wow the mic quality is so good
Looks interesting, but I can't figure out what steps you're skipping when you claim an bounds of zero and two for the integral wrt t.
specific heat of potato is about 3.4 kJ/kg
Here’s to hoping you continue this series
Nice one
Very interesting
WOAH!!! 🤯🤯🤯🤯
I've used f(z) = ln(z + ib) / (z² + a²) , b>0 And then contour integration by using upper side rectangular contour with residue at z = ia , and then equating real parts and we got it.
Integration by parts twice and substitution x = tan(t) leads us to the integral -4\int_{0}^{\frac{\pi}{2}}\ln{(cos{(t)})}dt
I'm curious to know how we can the pole at z=i because it is of infinite order and the power series can't be used as well due to the 1/(z+i) being there...any ideas on how to calculate it...?
The singularity at z=i is no longer a pole in this case, but an essential singularity because the Laurent series doesn’t terminate for some finite negative power.
I=int[-♾️,♾️]((1+x^2)^-(n+1))dx x=tan(y) dx=sec^2(y)dy I=int[-pi/2,pi/2](sec^(-2n)(y))dy I=2•int[0,pi/2](sin^0(y)cos^2n(y))dy beta(u,v)=2•int[0,pi/2](sin^(2u-1)(y)cos^(2v-1)(y))dy I=beta(1/2,n+1/2) I=sqrt(pi)gamma(n+1/2)/gamma(n+1)
y=x^t x=y^(1/t) dx=1/t•y^(1/t-1)•dy I=1/t^2•int[0,1](ln(y)ln(1-y)/y)dy u=ln(y) dv=ln(1-y)/y du=dy/y v=-Li_2(y) I=1/t^2•(-ln(y)Li_2(y)|[0,1]+int[0,1](Li_2(y)/y)dy) I=Li_3(1)/t^2 Li_3(1)=sum[k=1,♾️](1^k/k^3)=zeta(3) I=zeta(3)/t^2
what about the arcs tho?
I didn't use complex analysis but simple integration techniques and I got the result Integral(-½ to +½) Γ(1+x) Γ(1-x) dx = (4/π) β(2) = 4G/π Here β is dirichlet beta function.
But ginger sir , your solution is also really cool.
Please dont say lohopitals)
Choise of contours always seems completely arbitrary to me ://
bro forgot to edit out the first take
Well that's embarrassing... but hey it's fixed now :D
L'Hopital)
Take the derivative of both sides to give y’ = y’’ + y’’’ + … Then plug into the original equation to get y = 2y’ Giving the solution y = Ae^ (x/2)
@@henrydaley9686 I meannn you could… but Laplace transforms are fun…
@@GingerMathi mean why would we waste our time
Marvelous solution. Not the most original idea, but it never gets old.
cool method before i watch the video i think that you will use the box contour but i suprise by using the semicircle contour really wonderful 🥰🥰
really cool🥰🥰 i love this contour integration🤩 but sir can you explain why the second integral =- pi res(0) because i couldn't really understand. thank you !! and keep going! more contour integration😊
Glad you liked it!! Whenever we have a pole completely inside a contour (going counterclockwise), we would have 2pi*i*Res, but since the pole at zero lies ON the contour, we integrate around it with a semicircle (taking the limit as its radius goes to zero). The result is that instead of 2pi*i*Res is -pi*i*Res, as it is only a semicircle (so pi radians) and we are going around it clockwise, hence the minus sign. I'll be sure to add a bit on this for the next complex analysis video!
Another way you could do it: find a power series for ln(1-x^t)/x and then you pretty much all that's left in the integral is ln(x) ⋅ power function which is easily handled by integration by parts
try and get rid of the annoying background noise in future videos
try keeping that to yourself in the future
@@Potatos2980 lol be quiet child