- 3
- 23 310
Independent Quark
Sweden
Приєднався 26 гру 2024
Quarks seldom come alone.
I publish math & physics puzzles, lessons, tricks and more collected from years spent at science camps and international competitions.
I publish math & physics puzzles, lessons, tricks and more collected from years spent at science camps and international competitions.
How the Submarine Puzzle Uncovers Infinity
In this video we not only solve the tricky submarine puzzle - we stumble upon one of mathematics' most fascinating results: the size of infinities. In fact, we will touch the very limit of mathematical knowledge. We then introduce a new puzzle about an infinite grid of mosquitoes and a laser pointer, to test our newly learnt skills. Can you solve this new puzzle before my next video?
Original puzzle statement:
A spy submarine is moving along an infinite number line at an unknown velocity. Your mission? Find it before it disappears forever. Each turn, you get to search one position - but the submarine keeps moving at its mysterious speed.
This puzzle is rumored to be an old Google interview question. Thanks to my former calculus teacher for showing me this puzzle.
Note on Cantor diagonalization proof: there is a detail related to 1 = 0.99... which needs to be adressed in a more formal proof.
#MathPuzzles #Mathematics #Puzzle #infinity #Maths #ProblemSolving #Math #MathProblems #BrainTeaser #MathChallenge #SubmarinePuzzle #problems #phd #google #interviewquestions
Timestamps:
00:00 Intro
00:22 The Submarine Puzzle
01:15 Solution pt 1: v=0
02:25 Solution pt 2: x0=0
03:45 Solution pt 3: Full Puzzle
04:31 Approach 1
05:32 Approach 2
06:15 Countable Infinities
07:52 Other Types of Infinities
10:19 Puzzle: Mosquitoes and a Laser Pointer
10:51 Outro
Original puzzle statement:
A spy submarine is moving along an infinite number line at an unknown velocity. Your mission? Find it before it disappears forever. Each turn, you get to search one position - but the submarine keeps moving at its mysterious speed.
This puzzle is rumored to be an old Google interview question. Thanks to my former calculus teacher for showing me this puzzle.
Note on Cantor diagonalization proof: there is a detail related to 1 = 0.99... which needs to be adressed in a more formal proof.
#MathPuzzles #Mathematics #Puzzle #infinity #Maths #ProblemSolving #Math #MathProblems #BrainTeaser #MathChallenge #SubmarinePuzzle #problems #phd #google #interviewquestions
Timestamps:
00:00 Intro
00:22 The Submarine Puzzle
01:15 Solution pt 1: v=0
02:25 Solution pt 2: x0=0
03:45 Solution pt 3: Full Puzzle
04:31 Approach 1
05:32 Approach 2
06:15 Countable Infinities
07:52 Other Types of Infinities
10:19 Puzzle: Mosquitoes and a Laser Pointer
10:51 Outro
Переглядів: 15 045
Відео
This Submarine Puzzle Seems Impossible
Переглядів 8 тис.Місяць тому
A spy submarine is moving along an infinite number line at an unknown velocity. Your mission? Find it before it disappears forever! Each turn, you get to search one position - but the submarine keeps moving at its mysterious speed. This puzzle seems completely impossible at first. After all: - The submarine's velocity could be any integer (positive, negative, or zero) - The starting position co...
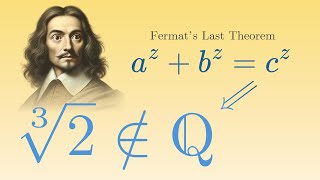
Actually Using Fermat's Last Theorem
Переглядів 710Місяць тому
Many have heard of Fermat's Last Theorem but few have actually used it as a tool for something else. It's been 358 tantalizing years but hey - New maths just dropped! We better find some use for these 128 pages and 7 years of Andrew Wiles' life. In this video we show that the cube-root of two is irrational using Fermat's Last Theorem, proven in 1995. *Note that this probably contains circular r...


the diagonalization proof presupposes that you can always do that for a unique number not on the list, but you cannot. you can do it ten times to every digit in the list and then have to do the next real number. which will only result in 10 unique numbers for every 2d integer in the bottom right quadrant. which shows its real easy to make a one to one correspondence.
I love a converse of the mosquito question. Real mosquitoes are not infinitesimal points. On any grid of real mosquitoes there is a 100% chance you’ll hit a mosquito pointing the laser in any direction. In fact, it doesn’t matter how far apart the mosquitoes are. They could be a kilometre apart or billions of light years and you’ll still eventually hit a mosquito. (For every irrational number there is an arbitrary close rational number.)
Do it with a rational number and velocity. Or on a multidimensional space (for a start, do it with gaussian integers). Or with algebraic numbers.
Each mosquito sits a point (x,y), in which both x and y are integers. Thus the angle at which the laser is pointed, equivalent to a hypotenuse, is a rational number. Firing the laser at an angle that is an irrational number is guaranteed never to hit a mosquito
Nice puzzle but music in the background very off putting.
I just saw the next video!
Very clear. Thank you for posting.
It's a really nice exposition, worth a subscription. See you around.
nice presentation. None of the daft hype and cartoons that often accompany videos on this topic; your explanations are spot on and the tenor of your voice convincing. Thank you. BTW, your question about the mosquito is irrational.
Thank you, glad you enjoyed it!
I semi-resonate with these lemniscates... ;)
What would Heisenberg say?
The solution to the mosquito puzzle (0% chance of hitting a mosquito when setting the laser at a random angle) implies that it is impossible to pick a random real number. The reason is that there are angle values for the laser that will make the laser hit a mosquito, they are just too few among the infinity type 2 angles. The mathematical implication is that the chance (infinity type 1 / infinity type 2) becomes zero, implying that one cannot even pick one of the successful angles (if that were possible, the chance would have to be non-zero). At first glance, this seems paradoxical, but I believe the paradox can be resolved in the following way: Picking an arbitrary real number requires specifying the number with infinity precision. This is simply not possible (provided one wants the pick to conclude in finite time). Although there are effective ways of picking some real numbers, one cannot pick all real numbers in an effective manner, meaning that the ability to randomly pick a real number from all real numbers is an impossible task. In other words, if one wants to set a random laser angle, one has to do it using an angle domain with a cardinality that is not larger than infinity type 1.
The ans to the second q is slope must be an iraational no .Now let's say our lazer hit a point (a,b) then the slope of line must be b/a bit if slope is irrational it is not possible
5:00 there's a lot of room for optimization here because a lot of those sports will have overlapping answers that for each spot you check you can cross off a lot of combinations
No you can’t hit no mosquitoes because if can’t hit no mosquitoes then there will be infinite mosquitoes forever and that means that the universe is broken and meaning has no meaning and that’s not possible.
This is very close to the general so-called Trawler Problem. If you only have as initial conditions a target estimate location at t0 (with or without covariance) and a max target speed Vt the optimal sensor pattern is a logarithmic spiral. But sensor has to be moving pretty fast wrt Vt. It’s simple to derive. Sensor can spiral out from t0 estimate location or in from Vt * time since t0. Inward saves a bit of time and makes the search area a bit smaller. B
I figure that, since the mosquitoes are on a grid, their coordinates are countable, essentially integers, along each axis. The direction from the origin (where you are with the laser pointer) to any given grid point P defines a line with slope Py/Px, which is a rational number. As you point out in the video, these are both of infinity type 1. The way to never hit a grid point would be to have a slope that is not a rational number, like pi. And if there are indeed infinitely more irrational numbers than there are rational ones, it would stand to reason that there's also infinitely many directions in which to shine the laser pointer without ever hitting a mosquito. The laser beam must of course also have 0 width.
I rarely subscribe to anyone nowadays, but you got it this time. I’ll remember I was here when you were still under 1k subs.
Easy. Just point the laser in any random direction. The probability of hitting a mosquito is exactly 0.
Hi, no soulution exists because at the origin there is a mosquito and the laser gun also....
mosquito puzzle seems easy. just point the Lazer in direction with a slope of any irrational number. because of the fact that irrational numbers can't be represented by the ratio of any two rational numbers there will no possible integer "rise over run" where the Lazer will hit a mosquito. ofc tho ideally we would do a spin with the laser and eliminate as many mosquitoes as possible
OHHHH WAIT A MINUTE! JUST POINT THE LASER SPIN AND CLOSE YOUR EYES THEN TURN ON THE LASER. THERE'S A ZERO PERCENT CHANCE OF HITTING A MOSQUITO
You can actually not make a list of even 1 real number.
Just turn it one radian, or turn it 60 degrees.
Use sine(zx)•e^px
Obviously, every mosquito represents some integer pair, like (a,b) which we can call a rational: a/b. Any line that does hit a mosquito must have a slope that intersects with a rational number, i.e. the slope divides a rational. In that case, any irrational number slop will be able to miss every mosquito.
You never hit a mosquito if the slope of the laser beam is irrational. The best way to avoid all mosquitos is the most irrational slope, namely the golden ratio.
Great video, but there is one mistake. We know that there is nothing between א0 and א1, Continuum hypothesis says that |R| = א1, and this we do not know, because |R| can be greater than א1 Edit: Source en.m.wikipedia.org/wiki/Continuum_hypothesis en.m.wikipedia.org/wiki/Continuum_hypothesis
Really excellent video.
I must have misunderstood what the submarine problem actually is. If the submarine starts at position 0, and has v=1, then it will move along the positive number line and get out of reach of the search position. The search will eventually reach every position that the submarine has been in the past, but will never reach the submarine.
No, you take starting position, then add the velocity times the number of seconds that have passed. This will eventually get you to the exact location that the submarine is, no matter the starting point or velocity
You understand the problem, but you’re missing a subtle part of the solution. We check every position based on velocity and time that has passed. This is not the same as just checking all positions.
i dont even know if i can 'randomly' choose a real number
Choose π
You cannot chose a real with a uniform distribution. But you can with e.g. a normal distribution.
you can’t actually sample it yourself or get a computer to do it, but that’s not to say you can’t determine properties of different distributions over the reals, such as the fact that in a uniform distribution hitting a rational has probability 0.
@@nboothhow would you go about sampling a real number from a normal distribution? I’m not sure it can be done
@ i asked to my friend, turned out it has something to do with measure idk
This is all trivial. This video doesnt even start to tackle any real issue related to infinity concept like axiom of choice or real numbers inconsistencies...
If it's all trivial to you, that means it's for people at a lower level. At some point in your life, this would have been new to you too.
Formal explanation(denote the set of all integers by Z): Assume that the start position and the velocity of the submarine is fixed, then we see that at time t, the submarine's location is at f(t)=a+bt for some fixed integers a, b for all non-negative integer time t(the function f is basically a representation of the location of the submarine). So to have a way of searching that will at some point find the submarine, its sufficient to find a sequence of integers(denote the sequence by {a} with a.i being the ith term) such that for all pair of integers (a, b), there exist a non-negative integer t with a.t=a+bt. Here, we treated a searching pattern over Z as a fixed infinite sequence of Z(which is a way to define what a searching pattern is in the first place). Let's first check that the above is indeed correct, assume such a sequence exist, then we will just search the ith term at time i, and by assumption, there exist t such that f(t)=a.t, so the submarine and where we search coincidence. Now, if for every sequence of integers, there exist (a, b) pair of integers such that f(t) = a+bt =/= a.t for all non-negative t, then indeed at least one possible movement of submarine with the given condition can escape the searching pattern. Therefore, the problem we transformed is equivalent to the original problem statement. Lemma: Given a countable set A, and an infinite sequence of functions f.t:A->Z, where t is a non-negative integer, let U:N->A(set of all non-negative integers) be a bijection, let {a} satisfy a.t = f.t(U(t)), then for all elements x of A, there exist t such that f.t(x) = a.t. Proof: This is quite simple, assume x is an element of A, then there exist non-negative integer y such that U(y) = x(since U is a bijection), so we see that a.y = f.y(U(y)) = f.y(x), as desired. Apply the above lemma to the problem with A = Z*Z and f.t(a, b) = a+bt solves the problem(specifically, imagine we mark (0, 0) at time t=0, and we will mark(one by one), all lattice points (x, y) such that |x|+|y| = k, where k=1, 2, 3... in that order(we won't move to the next value of k until we marked all the previous values of k). For example, you can mark them in the following order: (0, 1), (1, 0), (0, -1), (-1, 0), (0, 2), (1, 1), (2, 0), (-1, -1)... This is indeed a bijection between N and Z*Z(any lattice point will eventually appear in this sequence, this is similar to the proof that N and Q has the same cardinality but with a much more clear geometric intuition), call this sequence of lattice points {b}. Now, we let a.t = x+yt for all non-negative integer t, where b.t = (x, y), then since (a, b) is a fixed lattice point, we may choose t such that b.t = (a, b)({b} includes all elements in Z*Z so this must occur at some point), we indeed have a.t = a+bt = f.t(a, b), as desired). There might be some formal mistake inside of the argument, but the overall idea is basically identical.
Btw, Z can probably be replaced with any other countable set, since all we need is to just have the cardinality of the range to be the same as a. usual infinite sequence, which is just countable infinity.
Excuse me. It may well be that my English is way too bad. However, that unmotivated, superfluous and senseless background music makes it impossible for me to concentrate. Good bye to this channel.
I'm guessing that because the density of rational numbers in the reals is 0% that the mosquitoes are all completely safe from the laser.
My intuition is that the coordinates of each mosquito are countably infinite, and thus the number of angles I rotate to hit the mosquito are also countably infinite... but the possible angles my laser can point are uncountably infinite, so there is a set of angles infinitely large that would miss all mosquitoes. I have a 0% chance of hitting the mosquito on any try-and not just because my aim is bad.
Math says your aim is bad.
You can solve the mosquito puzzle by watching a Numberphile video from a few years ago.As I recall, as long as the laser is pointed at a tangent that is irrational, no bug will be hit. Of course, the lase beam has a dimension of a mathematical point.
By the way if anyone is wondering about the 3rd infinity here is how to get it: A set of all digits (0-9) has 10 elements. A set of pairs of digits (0-99) has 100 elements. A set of inf_1 digits has inf_2 elements. Real number are after all written as an infinite (inf_1) string of digits, but there is inf_2 many of them. So (a finite set)^(inf_1) is inf_2 big. An (inf_1 sized set)^(inf_1) is still just inf_2 big. In order to construct a "larger" set you would need to raise the power: (a finite set)^(inf_2) is inf_3 big. In other words, instead of being a countably infinite string of digits (real numbers) you would need an uncountably infinite string of digits. Another way to think about it is that an integer holds finite, but unlimited amount of information. A real number holds infinite, but countably information. The next level would need to hould uncountably infinite amount of information.
isn't that just map out to the difference between the space of rationals versus reals because hitting a mosquito means that you cross a point in the coordinate grid that maps to a representation of rational number
8:40 That approach does not work. 1 = 0.999…, but if you do 0,…,8, 9 → 1,…,9, 0, you map 0.999… to 1.000… which means you don’t get a new number. So if my list is nothing but copies of 0.999…, the “new” number is 1.000… which is already in the list. (If you require the numbers to be unique, use 0.999… as the first number and 0.9, 0.09, 0.009, etc. as the remaining number of the list.
Excellent point! Indeed one of the details of the proof that was left out for time. Can you come up with a method to account for this in the proof? (There are many possible ways) There is a discussion with some good approaches in another comment :)
The mosquito problem is actually easier. 😊 Short answer: Aim at such an angle θ whose tangent cannot be expressed algebraically. For ex. 10°. Or any angle (in degrees) not divisible by 3. Proof by contradiction: If tan(θ) = 9/25 for example, that means you will hit a mosquito every 25 steps horizontally and every 9 steps vertically. Going to ♾️ that's infinitely many mosquitos. Otherwise, nice video! A bit hard to understand, but... Anyway.
Point it at an irrational angle. Irrational can't be expressed with a fraction so it won't ever hit any rational coordinate.
If I could give this video 2 thumbs down, I would. Very poorly explained
Any particular part that you think could have been explained more clearly? :) Any feedback is much appreciated!
To me, it isn't clear why coming up with a clever way of counting all the integers by jumping back and forth across zero is an allowed behavior to count all the numbers
This is a really excellent video. Clean and clear videos, great explanation and you have a really nice speaking voice. I can't believe you have under 1k subscribers and I'm very glad I found you.
Thank you so much for the kind words! While getting into making videos I actually checked out your channel to try and see how successful channels do it and I really liked your content too! :)
@@IndependentQuark Oh, thanks! It's somewhat surreal to hear that other people know me from my channel.
Huge props for using a light beige background - it helps immensely with not straining eyesight at nighttime.
Actually, the rational numbers would be uncountable infinity, since you can always go up by a smaller amount. You would never make it past 1!
That's not how any of this works. It is possible to make a list of all rational numbers so therefore it is countable. You don't have to make a list /in order/
It all depends on the method of counting. As shown in the video, you can absolutely pick an approach that will not count through, for example, all integers. In fact, we can find a method that does not count through the natural numbers (the very definition of being countable!) Example: Start at 2 and go up. Once done, count 1. This will never get to the 1 since counting up will take forever. This does *not* mean that the natural numbers are uncountable. A set being countable just means that there exists at least one way to count through all the elements in that set, not that all methods need to succeed in that. To see how it works for the rationals it might help to think concretely about the order we go through them. In some sense, we grow both the numerator and denominator in parallel! So we will go "wider" and "deeper" at the same time. Hope this clarified things a bit!
I think my argument is rational enough: Take the distance between any two adjacent mosquitos (not diagonal) and call that 1 Since all mosquitos can be labeled by their distance from the laser along the x axis and along the y axis (a point with 2 coordinates) we can assign each mosquito a slope (it doesnt have to hr unique. Every slope will be a rational number, because each mosquito on an axis is an integer multiple of 1 away from the adjacent ones. All you have to do is point your laser towards a point with an irrational slope (such as the point (1, pi) and the laser will not hit any mosquitos. This is because if that line were to intersect a mosquito, it would imply the rational slope of the mosquito a/b would be equal to the irrational slope of pi/1 (or more generally, any irrational slope). This cannot happen because that would mean an irrational number is equal to a rational one.
These "infinity types" are called beth numbers
I am putting this here before he get famous
8:33 I have a question: What about situations similar to the trick where 0.(9) = 1? The numbers look different, yet they are equal. So why does having just one different digit in two numbers make them certainly unequal?
If one of those numbers is on the list then you won’t get such a number (such a number referring to one with many nines, and the number you got being the new number), since the new number will be different from any number on the list with infinite nines If such a number isn’t on the list, then either you won’t get one for your new number, in this case, no problem, or you will get one, but that would imply that at some point, there are only numbers that have 8 on the digit you select, then that digit before the 8s will have another +1 added to it, which is no problem since it’s still not equal to the original digit. And it can’t be equal to any number after since you’ll have only 0s (after you remove the 9s) in the digits when the 8s appear up to infinity, and each number has to have an 8 there. I hope I explained it well.
If the newly constructed number tails with an infinite string of nines, there are only finitely many real numbers in the list not containing an 8, so infinitely many numbers not containing an 8 are not in the list.
@ Wow, that much more straightforward than my explanation 😅
Excellent point and indeed a caveat I didn't cover for the sake of time. Some good explanations here :)
The easiest way to avoid 0.999 repeating = 1.0 schenanigans is just to add 2 to each digit instead of 1. Now the digit 9 becomes 1 instead of 0, so there's no way to accidentally run into an equivalent representation. Of course, this technique won't work in base 2... Another technique that does actually work in every base is to observe that in order to have a second representation of a number show up, we need something of the form ....0000 repeating forever (note that "9" will represent whatever number comes before "10" in whatever base we happen to be working in) in our constructed number. But this means that every number in our list had a 9 at an appropriate position, which clearly excludes a whole bunch of numbers that don't have a 9 anywhere. Even in base 2, where the only number without a "9" digit (more commonly written as "1" ) is 0, there are many numbers that only have a few "9"s near the beginning, followed by repeating 0s. We can see that we don't have enough room at the beginning of the list for all of them before our changing digit ends up falling in the repeating 0s in the tail.
Infinity is both a concurrently a singularity and an inverse of a universe pre big bang singularity. Existing in inverted dimensions of spacetime.
For the mosquito problem, just pick a direction at random, it will certainly work ;)
Wait you might be onto something actually, since the irrationals are uncountable infinity and the rationals are only countables
It will never work because you have already stepped on the mosquito at the origin
@@nayutaito9421 Make an equation ax + bc + c = 0 of the line, you can then choose a, b and c at random. The chances of choosing c=0 are infinitely small
only almost certainly :)
*almost-certainly