
- 21
- 11 836
Virtual Numbers
Pakistan
Приєднався 17 лис 2018
Exploring the mysteries of mathematics! Dive into the world of unsolved problems, mathematical paradoxes, and intriguing theories that have puzzled thinkers for centuries. Join us as we unravel fascinating concepts, challenge boundaries, and dive deep into the unknown to inspire curiosity and a love for mathematical mysteries
General Formula of Prime Numbers
In this video, we dive deep into one of the most fascinating concepts in mathematics: the general formula for prime numbers. Learn how to apply this groundbreaking formula to find prime numbers simply by inputting a number. We break down the process step by step, from understanding what prime numbers are to introducing the prime counting function and a new remainder notation. The final chapter reveals a formula that can predict prime numbers for any given natural number. Whether you're a math enthusiast or just curious about prime numbers, this video will provide a clear and easy-to-understand explanation. Watch now to discover this exciting new approach to prime number theory!
Facebook Page:
share/1D8nCAX9pk/
Hashtags:
#PrimeNumbers, #MathFormula, #PrimeCountingFunction, #Mathematics, #PrimeNumbersFormula, #NumberTheory, #MathTutorial, #MathematicsForAll, #PrimeNumberTheory, #LearnMath
Facebook Page:
share/1D8nCAX9pk/
Hashtags:
#PrimeNumbers, #MathFormula, #PrimeCountingFunction, #Mathematics, #PrimeNumbersFormula, #NumberTheory, #MathTutorial, #MathematicsForAll, #PrimeNumberTheory, #LearnMath
Переглядів: 154
Відео
Ln(0) | Value of Natural Log of 0
Переглядів 50412 годин тому
Welcome to this thought-provoking exploration of logarithms! In this video, we uncover the mystery of finding the natural logarithm of zero using the concept of the virtual unit, denoted as j. Dive into a step-by-step explanation involving the Taylor series, the Riemann zeta function, and how the unique properties of the virtual unit j redefine our understanding of mathematical extremes. From u...
Collatz Conjecture Proof !
Переглядів 56316 годин тому
In this video, we explore the Collatz Transformation, a powerful mathematical framework that provides insight into the Collatz Conjecture. We break down how this transformation works for odd and even numbers and show why it guarantees a unique loop for each . Whether you're a math enthusiast or a beginner, this video will help you understand why all numbers eventually reduce to one using the Co...
Can you Solve x/x = -1 | Singularity Numbers Part 2
Переглядів 2,1 тис.21 годину тому
In this video, we challenge traditional mathematics by exploring equations with no solutions in real or complex numbers. From equations like x times x equals one to the seemingly unsolvable x divided by x equals negative one, we journey into a realm of infinite possibilities using advanced mathematical systems. Watch as we introduce new concepts like singularity numbers and virtual units to red...
Deriving the General Quartic Formula Step-by-Step | Solve Any Quartic Equation
Переглядів 315День тому
In this video, we dive deep into the quartic formula, showing you step-by-step how to solve any 4th-degree polynomial equation. Starting from the general quartic equation, we use transformations, substitutions, and algebraic techniques to derive the complete solution for x. This comprehensive guide includes: 1.Shifting the equation to simplify calculations. 2. Introducing a cubic variable s to ...
is π rational? Understanding π = j / i
Переглядів 1,3 тис.День тому
In this video, we explore the equation using the principles of complex and virtual numbers. We’ll break down the calculation step by step, showing how this relationship provides a unique insight into the value of π (pi). We’ll delve into the mathematics behind this equation and its implications in modern mathematical frameworks. By understanding this relationship, viewers will gain a deeper app...
Singularity Numbers: Redefining Division by Zero and Beyond
Переглядів 723День тому
Explore the fascinating world of Singularity Numbers, where the mysteries of dividing by zero are finally unraveled. In this video, we redefine concepts like one divided by zero and zero divided by zero, introducing the unique number "k" with its extraordinary properties. Learn how "k" behaves in exponential, sine, cosine, and logarithmic functions, and discover its applications in physics, eng...
why e^j = 1+j | Virtual Numbers Part 2
Переглядів 82714 днів тому
In this video, we dive into the fascinating world of virtual numbers and explore why the square and all higher powers of these numbers are zero. I'll explain this concept step-by-step, starting with the product of logarithms, then showing how we can derive that powers of virtual numbers lead to zero. If you're curious about virtual numbers and want to understand their unique properties, this vi...
Introduction to Virtual Numbers: A New Era in Mathematics
Переглядів 5 тис.14 днів тому
Explore the groundbreaking world of Virtual Numbers, a revolutionary concept in mathematics that simplifies exponential and trigonometric functions, redefines powers, and introduces new perspectives on logarithms. In this video, we discuss how Virtual Numbers are built around the unit j, defined as the natural logarithm of negative one, and how they differ from imaginary and complex numbers. Le...
Cubic Formula
Переглядів 320Місяць тому
Discover the secrets of solving cubic equations as we derive the general formula step by step! From the rich historical origins of cubic equations, dating back to ancient Babylon and Renaissance breakthroughs by mathematicians like Tartaglia and Cardano, to the modern method of solving them using substitutions and algebra, this video covers it all. Learn about the behavior of cubic functions, h...
1 + 1 = 3
Переглядів 166Місяць тому
In this video, we're exploring a fascinating mathematical trick that "proves" seemingly impossible equations, like "1 1 = 3" or "2 2 = 5." I'll take you step-by-step through a clever manipulation of numbers to show how this works and even reveal a method that can make *any two numbers equal each other*! It's an intriguing dive into the world of math tricks and unusual equations that anyone can ...
Derive a New Quadratic Formula Easily | Step-by-Step Math Tutorial
Переглядів 26Місяць тому
In this video, we dive into deriving a fresh quadratic formula using the classic quadratic equation as our foundation. Follow along as we explain each step in a straightforward and accessible way, providing insights for both beginners and advanced math enthusiasts. Subscribe for more in-depth math content! #QuadraticFormula #MathTutorial #Mathematics #Algebra #MathProof #EquationDerivation #Adv...
How to Find the Product of Two Logarithms: log(a) × log (b)
Переглядів 78Місяць тому
In this video, I’ll guide you through finding the product of two logarithms, breaking down each step to make it easy to follow. We’ll explore essential logarithmic rules, simplify complex equations, and put the formula to the test with a practical example. By the end of this tutorial, you’ll understand how to approach similar problems with confidence. If you enjoyed this video or found it helpf...
Difference between Two Relative Squares
Переглядів 163 роки тому
Difference between Two Relative Squares
13_4 Conjecture and Collatz Conjecture @veritasium
Переглядів 2683 роки тому
13_4 Conjecture and Collatz Conjecture @veritasium






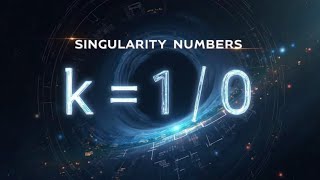


im pretty sure these are isomorphic to complex numbers
just use phi:C->V with phi(a+bi)=a+(b/pi)*j
aren't this dual numbers?
e^j + 1 = 0 e^j = 1 + j Subtract both equations j = -2 Which is impossible You should create also new axioms for consistency. I think there’s no working axioms like “(a + bj) + (c + dj) = (a+c) + (b+d)j” and “(a + bj) * (c + dj) = ac + (bc + ad)j”. So even the “e^j + 1 = 0” is wrong, or just j^2 is not 0.
You are write, problem with j² and other higher powers, I will fix it in my next video
Sqrt(sqr(a)) =|a| - proof is incorrect
It's true for real numbers √(-2)²= ±2
"The Hindu religion is the only one of the world's great faiths dedicated to the idea that the Cosmos itself undergoes an immense, indeed an infinite, number of deaths and rebirths. It is the only religion in which the time scales correspond to those of modern scientific cosmology. Its cycles run from our ordinary day and night to a day and night of Brahma, 8.64 billion years long. Longer than the age of the Earth or the Sun and about half the time since the Big Bang." “Most cultures imagine the world to be a few hundred human generations old. Hardly anyone guessed that the cosmos might be far older but the ancient Hindus did,” "It is the only religion in which the time scales correspond, to those of modern scientific cosmology" ~ CARL SAGAN (famous astronomer, cosmologist)
💕
crazy for someone to think that their math leads to 1 + 1 equalling 3 doesn't mean that their math is wrong but that 1 + 1 does equal 3
I don't know enough about mathematics yet to be a critic of the creative process. I don't know if this is right or wrong but you got a new subscriber! 😊
Thanks
Why j²=0 ?
watch second video of e^j video
I've applied the same concept in creating a prime sequence. This method is straightforward because it involves keeping track of the prime count. The principle used here is p ∣ (n, k) for all k except 1 and n. However, finding the n-th prime using this approach requires the prime counting function π(x), which provides an approximation of the number of primes less than or equal to x using x/ln(x), rather than the exact value. Consequently, we cannot determine with certainty the exact value of n for which a specific prime is found.
I also tried using the method p | (m, floor(m/2) menus 1), but it may not work for all cases. I'm currently working on an alternative method to find all prime
This video is incomplete. The general formula presented (a) is not proven, and (b) if true, then still needs (at worst) floor(m/2) divisions against the set to verify if m is prime. [Remember that formation of set needs lots of computation!] If true, then this can beat the simple division test of m that always needs floor(m/2) divisions.
Yes, it's not perfect, but it works well. In my next video, I will create a formula for primes using a different method.
At 6:15 it is said none of m=9 elements are divisible by 9, even though first two elements are.
Must divide set S9 , all elements
Then a language barrier? Correct way is to say the whole set is not divisible by 9.
yes , 9 must divide all elements of S9 as R(S9,9) must be 0
dats cray cray!
Glad you find it interesting!
It is not first ever formula for prime numbers. There exists others, but they are so inefficient that it is faster to compute them directly.
also explain more practical and graphical approach
you can try your self , Ej =0 , Oj=j , E is even O is odd and j^n=0 for n>1
use binomial theorem to expand such numbers
Please make it right for the future. Dont let this not need construct in mathematic. Redefine "e" to what it realy is. It is nicer and more intuitive. Nothing will change but it will work better. I can show you how it work. I didt want public before, but if you do so. Make it moce intuitive for reality and for future.
Send.detail on Facebook page
x/x=-1 then x=0 0=-1*0 Because 0/0 is set of all number
We can write 0/0 = -1 as 0= 0×-1 0/0 is not 1.. It's 0k in singularity numbers
Irational a/b only if a and b is integer lol 😂
It means 1/0 is rational?
@@log_menus_1 you demolished & annihilated that oversmart coward 😂
Lets do it right with redefine "e" to fractal. Dont let this no need of extra structures in mathematic for future. It will be better. Please contact me.
You can contact me on my Facebook page, check out UA-cam bio
Thank fo you work, ihave been working on this concept a half year ago. I did not want to put it into world. If you put into world already, lets do it complex as it is without virtual numbers but with redefine "e" as it is in reality. You can mail mail me.
Are u talking about primes formula or virtual numbers?
Cool it's like CP Willians sequential prime number formula except with virtual numbers.
Today I learnt that X/log(X) gives the number of primes below X. Crazy!!!!
My answer: x = 0, because 0 divided itself can be any numbers.
Any numbers? 😂😂😂 Can it be 2?
If any number then this will not satisfy the equation but I think only singularity numbers satisfy the equation
this is a great exercise to test your ability to detect false logic
x/x = -1 already in F2
j = i*π
Multiply by 2
A general formula for P would take a number n and find m such that P(n)=m. What your formula does is take a number m and find n such that P(n)=m, and your formula does this by defining n=π(m). All this video shows is that P is the inverse function of π if and only if its argument is prime :/ That's not a technique for computing P for any input
We can also find the nth prime. For example, if π(m) = 100, this represents the 100th prime. By using the π formula, we can determine the value of m and then proceed with the calculation.
@ That's not at all what it means to have a general formula!! If you want to compute P(100000), you need to first know the smallest m such that π(m)=100000, and that m is the output of P. Looking through the π function for such a value is not an "efficient technique" for calculting P, you are offloading the work of finding primes onto your π function.
We can find m using pi function
@@log_menus_1 Looking through the π function for such a value is not an "efficient technique" for calculting P, you are offloading the work of finding primes onto your π function.
We can find all the primes using formula.. I will make it better in future videos
There is a solution to your equation in infinitely many fields. Since x/x = 1 in any field and for any non-zero value of x, if you want x/x = -1 then you must have -1 =1. That is true in any field with characteristic 2: in other words, 1 + 1 = 0. This is true on the residue classes modulo 2, often written as ℤ₂ or ℤ/[2]. In this field, the only values are 0 and 1, with 1+1 = 0 and 1*1 = 1. There are many other such fields; for example there is one with a size of any power of 2, and there are infinite-sized such fields. I do not know the "virtual number" system that you use, but it seems not to be a field (a number system with the usual, base properties such as closure, commutativity, and associativity of addition and multiplication as well as the distributive property of multiplication over addition).
Watch virtual numbers video to understand
@@log_menus_1 I watched that video after posting my comment. I found the video hard to understand, since you use some properties of the Real and Complex numbers but ignore others, and it is not clear which properties you are keeping, which ones you are not, and which new properties you are adding. (Those things are clear for a few properties but not for all.) Is there a reference that gives the axioms for the Virtual Numbers?
I understand how the use of familiar properties from real and complex numbers, along with new concepts like Virtual Numbers, can create some confusion. The goal is to establish a new framework that extends beyond the traditional number systems. I'll be sure to clarify the axioms of Virtual Numbers in future videos and provide more concrete examples.
When one divides by 0, then anything is true.
No 👎
@@log_menus_1 At 4:50, that's a division by 0.
1/0 is singularity unit which is k
so zeta 1 is basically infinity, this is like saying log 0 = - infinity
Infinity is not a number; it's a concept that represents something extremely large that we cannot write or count. If infinity were a number, we could add 1 to it, but that would contradict the idea of infinity. Additionally, there is no such thing as positive or negative infinity-it's simply a concept that doesn't have a sign. Remember, infinity is just a concept, not a number.
Not agree... knowing ALLAH is obvious by just admiring creatures around you (yourself, nature...)
I will create a comprehensive video on the existence of God using mathematics in future videos. So stay tuned and subscribe to the channel. This video was just a short preview, and I didn’t explain much.
Total fraud. Before solving any equation, domain must be specified.
If I had asked you to solve x² = -1 before the discovery of complex numbers, would you still ask me to specify the domain? My question is straightforward: find x if x / x = -1.
@@log_menus_1 If you do not specify the domain, then symbol "/" has no meaning and the problem is nonsense. And yes, to pose problem x^2=-1 you have to either specify the domain, or to explicitly request to invent new domain in which symbols -1 and ^2 have meaning. Again: your formulation is dishonest and intends to confuse those who do not have serious mathematical education.
@@log_menus_1😂😂😂😂 "discovery of complex numbers"...
The question of whether mathematics is discovered or invented is indeed a long-standing debate. In the case of complex numbers, I believe they were discovered, not invented. While the formalization of complex numbers as we know them today involved human ingenuity, the concept itself already existed, much like negative or irrational numbers. These mathematical ideas were always part of the structure of the universe; we simply needed to recognize and understand them. So, complex numbers weren’t invented-they were uncovered.
@@xgx899 The question is simple: find x if x / x = -1. You can use any domain or even invent a new one, as long as it satisfies the equation, just like how we extended the domain from real numbers to complex numbers.
this really demands a proof that j^2 = 0. j^2 = j*j = j*log-1 = log((-1)^j) log(j^2) = 2*log j = -2 you can't have the laws of logarithms if you head down that path
Well... 0 = j^2 = j*j = j*ln-1 = ln((-1)^j) = ln(1 + j*ln(-1)) = ln(1 + j*j) = ln(1+ 0) = ln(1) = 0 ...does follow. Also, ln(j^2) would be ln(0), which was not defined anyway, so... You're kind of misusing the notation like that.
Pointless, useless, totally absurd. 😂😂😂
If my method is wrong, feel free to try your own method. Find .
@log_menus_1 What method??? As if it wasn't enough absurd with the imaginary numbers now we get the singularity numbers!
I understand that the concepts of Virtual Numbers and Singularity Numbers might seem unconventional at first, especially when compared to more familiar ideas like imaginary numbers. The goal here is to explore new ways of thinking about numbers and challenge traditional frameworks.
@log_menus_1 Traditional framework is just fine and it's the base for everything. Imaginary, singularity, etc numbers belong to marvel universe and serve other purposes.
Mushroom time :)
I love this idea! Personally, I’ve been using д^0=д,д^2=-д as an infinity, with 0*д=2/3. I think you would find it interesting to review?
XD you just typed in some command for chat gpt
I couldn't write these equations in PowerPoint, so I used ChatGPT. However, ChatGPT doesn't work on its own-it follows the instructions I provide on what to do and how to do it.
1. Wtf is j? I would say it is i*pi/2, bit you write that cj=j for even c. So what the hell is it??2. Harmonic series is not zeta of 1, it diverges. Zeta is undefined for numbers with real part less or equal 1. You would have to do an analytic continuation, which you don't say anything about
It seems you haven’t watched my video on Virtual Numbers. To clarify, j is the unit of Virtual Numbers, and ζ(1) refers to the sum of reciprocals of natural numbers: ζ(1) = 1 + 1/2 + 1/3 + 1/4 + ... This series is typically labeled as divergent, but I call it an irrational series, as its sum is unknown and not finite. Another example is the Gregory-Leibniz series for π: π = 3.14159265359... π= 3 + .14159265359... π - 3 = 1/10..0 + 4/10..0 + 1/10..0 + ... This series diverges but still converges to the value of π, showing how infinite processes can yield meaningful results. The k in the video is the Singularity Unit, and terms like divergent, infinity, and undefined simply reflect our current lack of understanding or methods to solve such concepts.
@log_menus_1 from what I understand you coined the term virtual number yourself (I couldn't find it anywhere on the internet). Could you rigourously define it? The harmonic sum IS divergent to infinity no matter how you look at it. And it IS NOT zeta of 1. The sum IS known. From what i searched the Gregory Leibniz series is just arctangent Taylor expansion evaluated at 1. What you wrote is just a sequence of decimal expansions of subsequent approximations of pi. It converges to pi. You can define every real number as limit of sequence of rationals.
The term 'sum/sequence divergent to infinity' has a strict definition, so don't yap about 'current lack of understanding'
The concepts of Virtual Numbers and Singularity Numbers are not just imaginative ideas-they’re part of an effort to push the boundaries of traditional mathematical thinking. While these concepts may seem unconventional, they are based on logical frameworks that challenge existing number systems. As for the channel, we focus on providing explanations and theoretical foundations. If you're open to it, I'd be happy to explain the reasoning behind these ideas in more detail!
AI is simply a tool, much like a calculator, used to assist in tasks like script writing and text-to-speech. As English is not my native language, I use AI to help ensure my message is clear and understandable to a wider audience. The purpose is to make sure everyone can follow along with the ideas I’m presenting. If my concepts are difficult to grasp, it might be due to a lack of familiarity with advanced mathematical ideas, but I encourage you not to misguide others. Ultimately, viewers will decide whether they find the videos valuable-if they do, they will watch; if not, they are free to move on. My goal is to share these concepts with those who are interested.
j=ln(-1)=iπ iπ/i=π
j = iπ π = j/i
@log_menus_1 exactly what i've said, thank you for your videos, could you make more about this new number's family ? i really love your channel
Another amazing video 💯👍🤩
Wait for another video on (-1)! 😊
Greetings Sir. Could you show me how you obtein the expression √j = e^√j / e ? Thank you for you kind response.
In my future videos
Is 0k=1? because k=1/0
No... 0k = 0/0 which is not 1 .. K = 1/0 Multiply by 0 on both sides 0k = (1/0)*0/1 0k = 0/0 So singularity numbers={...,-2k,-k, 0k,1k,2k,...}
Is this is true 0=1/k
Not sure whats going on here, but it seems suspicious.
X,2×+5=8
??
No, sorry. Just like multiplication is repetitive addition, division is repetitive subtraction. A divided by B is the number of times you can subtract B from A until you reach 0. So X divided by X is the number of times x is subtracted from x to equal 0. It is 1 and only 1. It can never be -1. Basically you're asking us to solve an equation that is incorrect. Not a valid question.
If I had asked you to solve the equation x² = -1 before the discovery of complex numbers, you would have said the question is invalid because no number satisfies that equation. Similarly, my question is: find x if x/x = -1. This is analogous to asking for x when x² = -1 before complex numbers were introduced. The point is, my question is valid-you just don’t have an answer for it in the complex numbers framework you're using.
@@log_menus_1 My man, complex numbers are a model that works mathematically and is consistent with basic arithmetic and geometric rules. You just made up a random ass number, said it works, and then broke math. You can always introduce new definitions in mathematics, but you MUST make sure that they are consistent or at least put some sort of boundaries. When using definitions that have some errors, results, at best, need some huge asterisks. However this is complete nonsense. Also are you an AI? Is this a social experiment?
I didn’t break math at all; virtual numbers are consistent and have their own framework where all operations are done, similar to how complex numbers and real numbers each have their own mathematical system. In my introduction to virtual numbers, I showed how they work when we extend the domain of functions from real to virtual. For example, 0! = 1 makes sense if we consider n! = n * (n-1) * (n-2) * ... * 1. When we put n = 0, we get 0! = 0 * (-1) * (-2) * ... * (-n). This results in 0! = 0. Would you say I’m wrong here as well? Also, negative numbers are undefined in the for factorial, but when we extend the domain of integers to complex numbers, we get different results. For instance, (-1/2)! = √π. The results with virtual numbers may seem counterintuitive, but they are correct due to the extension of the real domain to the virtual numbers system. I hope this makes sense.
uhh
ln(0) is undefined
Nothing is undefined
Undefined means it can have more than one definition. Depends on how you want to define it.
1/0 is also undefined but we use it often as infinity instead of writing 1/0
AI is simply a tool, much like a calculator, used to assist in tasks like script writing and text-to-speech. As English is not my native language, I use AI to help ensure my message is clear and understandable to a wider audience. The purpose is to make sure everyone can follow along with the ideas I’m presenting. If my concepts are difficult to grasp, it might be due to a lack of familiarity with advanced mathematical ideas, but I encourage you not to misguide others. Ultimately, viewers will decide whether they find the videos valuable-if they do, they will watch; if not, they are free to move on. My goal is to share these concepts with those who are interested.
It's an interesting idea to try to define ln(-1) as j, an entirely new number system. But if you try and follow basic rules, then ln(-1)+ln(-1)=ln(1)=0 j+j=0 j=0 But if you're proposing this is an entirely new system that doesn't follow standard arithmetic rules, then does this solution to x/x=-1 work? It seems to be using j with standard arithmetic rules, which leads to contradictions. If you don't want contradictions and want to create an entirely new system, it might be better off defining what you're allowed to do and what you can't do with j.
j is actually i*pi, if you do j+j, or 2*j, you'll get 2*i*pi
Actually it follows the basic arithmetic as well j + j = 2j = 2ln(-1) = ln(-1)²= ln(1) = 0 Here 2j = 0 not 2 or j individually 0
Also j+j = 0 is true j = -j Ln(-1) = - ln(-1) Ln(-1) = ln(-1) j= j Actually -j = j So it follows basic arithmetic
2j = 0 so it means 2i*pi = 0?
@@log_menus_1 Ok, that's interesting. So you propose 2j=0 doesn't mean you can divide both sides by 2? Then it wouldn't follow that 2ipi=0, because in this case, you CAN divide by 2, giving ipi=0, which isn't true. This means that you might need to try redefining what it means to "multiply", so that the definition allows you to deal with both normal numbers like pi or 2 or i, and your new number j, and even k. For example, if 2j=0 but j isn't 0, what does the 2 mean? Without the 2, j=ln(-1), but with it, 2j=0. Can you find some rule to figure out what 3j is, then what 4j is, etc. without any contradictions arising? Are you able to do the same with K? How about numbers like j^k? Right now, your theory is interesting, but it isn't standing on many foundations. People are pointing out "there's contradictions" or "there's inconsistencies", because the rules for what you can and can't do with j and k are unclear. If you can figure out what these rules are, and prove they're perfectly consistent, then you'll have a theory that some experts might start considering, and something maybe no one has done before. If you fail, maybe it wasn't possible, but it would be one of the greatest learning experiences, and you'd have a much deeper understanding of why you can't divide by 0 than many other people who just read textbooks. Learning from actually researching and proving things yourself is incredibly valuable and something not many people can do. And if you're curious about how you're even supposed to build these rules for j and k, you might want to look into areas of math called "foundations of mathematics", "mathematical logic" or "proof theory". These areas deal with axiomatic systems, or those rules you place, and how to prove they're reliable rules.
why j²=0? ln(-1)²=0?
Watch my other video title e^j = 1+j
solutions for x/x=1 are all non-complex numbers
No, all numbers. Every number is complex. Complex numbers can be expressed in polar or standard form, so we’ll go with standard: a+bi 6=6+0i has a real and imaginary part and is therefore complex. You must think that a and/or b can’t be zero, but even in that case, x/x is always 1 Plus, that wasn’t even the question
But question was x/x = -1
I know always x/x = 1 , My question was find x if x/x = -1
@@log_menus_1 you’re actually stupid
thanks
j=ln(-1) j=iπ j²=-π² 😂not 0
It's correct that Ln(-1) = πi Multiply both sides by 2 .. 2Ln(-1) = 2πi 0 = 2πi Also j² = 0.if you watch my video on e^j = 1+j u will understand