
- 145
- 120 732
Calimath
Приєднався 17 лип 2022
We present motivations and solutions to olympiad problems in algebra, combinatorics, geometry and number theory.
For every video, we publish an additional pdf document with the explanation on our website and give references for all statements we used in our proof. You can find all the answers and our wiki on our website calimath.org.
So we want to give everyone the chance to have a close look at olympiad maths.
For every video, we publish an additional pdf document with the explanation on our website and give references for all statements we used in our proof. You can find all the answers and our wiki on our website calimath.org.
So we want to give everyone the chance to have a close look at olympiad maths.
Why almost everything holds for 60-degree triangles
In this video, we present a solution to MEMO 2024/T5.
00:00 Problem Statement
01:03 Solution
Check out our website www.calimath.org to find past problems, solutions and other cool stuff.
Solution PDF: calimath.org/pdf/MEMO2024-T5.pdf
branch: geometry
difficulty: 6
Our website: www.calimath.org
Contact us: calimath.business@gmail.com
00:00 Problem Statement
01:03 Solution
Check out our website www.calimath.org to find past problems, solutions and other cool stuff.
Solution PDF: calimath.org/pdf/MEMO2024-T5.pdf
branch: geometry
difficulty: 6
Our website: www.calimath.org
Contact us: calimath.business@gmail.com
Переглядів: 165
Відео
Foundations of Probability Theory: Building a Rigorous Understanding of probability
Переглядів 4,8 тис.14 днів тому
The skillpage to probability theory: calimath.org/skillpages/probability-theory Check out our 500 subscriber special, where we introduced the skillpages: ua-cam.com/video/5w38Tln6p_4/v-deo.html Check out our website www.calimath.org to find past problems, solutions and other cool stuff. 00:00 Introduction 00:51 1.1 Probability Space (Definition) 06:10 1.2 Probability Space (Elementary Propertie...
Power towers in a functional equation?!
Переглядів 61221 день тому
In this video, we present a solution to IMO Shortlist 2023/N8. 00:00 Problem Statement 00:31 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/ISL2023-N8.pdf branch: number theory difficulty: 9 Our website: www.calimath.org Contact us: calimath.business@gmail.com
All properties of radical axes in one problem
Переглядів 36728 днів тому
In this video, we present a solution to EGMO 2023/6. 00:00 Problem Statement 01:06 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/EGMO2023-6.pdf Wiki links to used statements: - 4:35 calimath.org/wiki/power-of-a-point-theorem - 5:10 calimath.org/wiki/harmonic-pairs-of-points branch: geometry difficulty: 9 Our...
A functional inequality from last year's IMO shortlist
Переглядів 459Місяць тому
In this video, we present a solution to IMO Shortlist 2023/A4. 00:00 Problem Statement 00:36 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/ISL2023-A4.pdf branch: algebra difficulty: 7 Our website: www.calimath.org Contact us: calimath.business@gmail.com
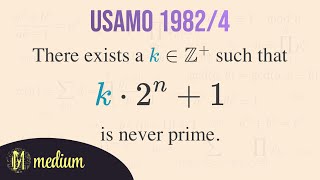
Pierre de Fermat couldn't find this solution
Переглядів 1,5 тис.Місяць тому
In this video, we present a solution to USAMO 1982/4. 00:00 Problem Statement 00:24 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/USAMO1982-4.pdf branch: number theory difficulty: 6 Our website: www.calimath.org Contact us: calimath.business@gmail.com
This FE was the most difficult problem on the INTERNATIONAL MATH OLYMPIAD 2024
Переглядів 2,2 тис.Місяць тому
In this video, we present a solution to IMO 2024/6. 00:00 Problem Statement 01:07 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/IMO2024-6.pdf branch: algebra difficulty: 11 Our website: www.calimath.org Contact us: calimath.business@gmail.com
This is a solution using a result from graph theory
Переглядів 3592 місяці тому
In this video, we present a solution to the German National Competition 2020/2.4. 00:00 Problem Statement 00:47 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/BWM2020-2-4.pdf Wiki links to used statements: - 4:32 calimath.org/wiki/halls-marriage-theorem branch: combinatorics difficulty: 8 Our website: www.cal...
The fastest solution to a problem 2 in INTERNATIONAL MATH OLYMPIAD
Переглядів 1,2 тис.2 місяці тому
In this video, we present a solution to IMO 2024/2. 00:00 Problem Statement 00:35 Motivation 04:42 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/IMO2024-2.pdf Wiki links to used statements: - 4:47 calimath.org/wiki/eulers-totient-function - 4:58 calimath.org/wiki/fermats-little-theorem branch: number theory ...
INTERNATIONAL MATH OLYMPIAD 2024/4: The power of reflection
Переглядів 4382 місяці тому
In this video, we present a solution to IMO2024/4 00:00 Problem Statement 01:09 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/IMO2024-4.pdf branch: geometry difficulty: 7 Our website: www.calimath.org Contact us: calimath.business@gmail.com
You can probably guess the solution to this INTERNATIONAL MATH OLYMPIAD 2024 problem
Переглядів 8072 місяці тому
In this video, we present a solution to IMO 2024/1. 00:00 Problem Statement 00:32 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/IMO2024-1.pdf branch: algebra difficulty: 5 Our website: www.calimath.org Contact us: calimath.business@gmail.com
A math problem every sports player has thought about (feat. Fibonacci numbers)
Переглядів 6884 місяці тому
In this video, we present a solution to RMM Shortlist 2018/C3. 00:00 Problem Statement 00:57 Solution Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/RMM-SL2018-C3.pdf branch: combinatorics difficulty: 7 Our website: www.calimath.org Contact us: calimath.business@gmail.com
You can't guess the solutions to this functional equation
Переглядів 8975 місяців тому
In this video, we present a solution to Malaysia Selection Test for the APMO 2024/3. 00:00 Problem Statement 00:34 Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/MalaysiaAPMOSelection2024-3.pdf branch: algebra difficulty: 7 Our website: www.calimath.org Contact us: calimath.business@gmail.com
Only the best IMO participants are invited to this competition
Переглядів 7635 місяців тому
In this video, we present a solution to IMO 2023/5. 00:00 Problem Statement 00:39 Check out our website www.calimath.org to find past problems, solutions and other cool stuff. Solution PDF: calimath.org/pdf/RMM2024-4.pdf branch: number theory difficulty: 6 Our website: www.calimath.org Contact us: calimath.business@gmail.com
These numbers rarely divide each other
Переглядів 6255 місяців тому
These numbers rarely divide each other
INTERNATIONAL MATH OLYMPIAD 2023 | Problem 5
Переглядів 4,1 тис.5 місяців тому
INTERNATIONAL MATH OLYMPIAD 2023 | Problem 5
Peculiar numbers in the European Girls Math Olympiad
Переглядів 5215 місяців тому
Peculiar numbers in the European Girls Math Olympiad
How to count the 1's in an infinite binary representation
Переглядів 5106 місяців тому
How to count the 1's in an infinite binary representation
Can you find subsets with the best properties?
Переглядів 5906 місяців тому
Can you find subsets with the best properties?
A probabilistic approach to a very hard number theory problem
Переглядів 7276 місяців тому
A probabilistic approach to a very hard number theory problem
An interesting property of the divisors of n!
Переглядів 4627 місяців тому
An interesting property of the divisors of n!
How to deal with infinitely large sets
Переглядів 4187 місяців тому
How to deal with infinitely large sets
A functional equation for number theorists
Переглядів 3837 місяців тому
A functional equation for number theorists
Counting the number of subsets with a prescribed sum
Переглядів 2817 місяців тому
Counting the number of subsets with a prescribed sum
One of the most important properties about excircles
Переглядів 1 тис.8 місяців тому
One of the most important properties about excircles
Discovering a beautiful property of this sequence
Переглядів 3858 місяців тому
Discovering a beautiful property of this sequence
The final problem in the INTERNATIONAL MATH OLYMPIAD 1977
Переглядів 1,1 тис.8 місяців тому
The final problem in the INTERNATIONAL MATH OLYMPIAD 1977
The hardest functional equation in the history of USA Team Selection Tests.
Переглядів 1,5 тис.9 місяців тому
The hardest functional equation in the history of USA Team Selection Tests.
Chinese students had to solve this to qualify for the International Math Olympiad
Переглядів 3439 місяців тому
Chinese students had to solve this to qualify for the International Math Olympiad








How does the bound from above @6:08 work? Why is there a fixed c such that for every large enough n the inequality holds?
No way, I have actually compeated on this MEMO, It took us (Croatia) to solve this one 10 minutes but it was the only one on the team competition that We aced.
Awesome funcional equation and clever solution!
3:38 Why was it enough to show that AF=OF?
Nice
Thank you so much 🙌🏾
Thanks for the video. I am unsure as to why you motivate the expected value as a linear function that must be equal to the probability of the event for the case of an indicator. Why is that more fundamental or why not just observe that from the definition?
You are welcome :) From the perspective of introducing new theory, it is nice if you can motivate it, i.e., argue why the definitions are natural. That is why I took this axiomatic approach: I wanted to make the argument that this is really the only sensible definition of expectation. Since both characterizations of expectations are equivalent, they are equally fundamental and doing it this way was just a personal choice.
@@calimath6701 But wanted to ask you why is it something evident that E(I{A})=P(A) must hold?
I don't think that that is totally evident. In the discrete case, I would even agree that it is not more evident than the definition of expectation. However, it is a nice way to think about expectations and in hindsight I probably presented it with that order since we can use the ideas to define (lebesgue) integrals which we need in order to define the expectation of a general (not necessarily discrete) random variable
@@calimath6701 it would be awesome if you made a video connecting that to general continuos variables, and the analogous way of defining X as a sum of indicators in that case
This is really well done, thank you 🙏
Thank You
This is really great, better than A LOT of measure theoretic so called "Introduction" textbook! Please make more :)
Great video. A Second video on this topic would be highly appreciated.
Funny intro. These type of videos are amazing. More of then would be amazing.
Sooo gooodd
Hi there ,there's a small request pls bring series that teaches mathematics from basic to Olympiad level if you do that then it is going to very helpful for students ❤ by the way your knowledge is amazing appreciate your work man
On Friday, we will be publishing a new introduction video. We hope you will enjoy it! Besides that, check out the skillpage and wiki entries on our website. Our goal is to bring all the important basics for Olympiad math onto it.
Can you do some videos on Grid combinatorics problems and Combinatorics problems from Russia?
from Morocco thank you son...despite my foccusing and attempt to understand...i couldnot...surely i m so old for that🤣😂 i shared your link on my facebook page
Thanks for sharing us on facebook. If you have any suggestions on how we can improve the understandability, we would be happy if you let us know.
@@calimath6701 you re pefect just go on...thank you
amazing!
Very very cool! Keep up the great work
Amazing
Amazing vid/!!
Nice stuff
MATHA SIZE THORAA BARAA KARO...
It all seems very complex, it would be great if you could make a video starting from grass root level of maths and explaining all concepts irrespective of it being long otherwise great video🫡
Thanks. I see your point. Starting from grass root level in all aspects is not feasible right now. However: we will have a video attempting exactly that for probability theory in a few weeks. So, this is going to help with this video a lot :)
Guys, if you start organising contests you probably will have a very good community.
Recently I solved a problem to find all finite sets that satisfy: if a and b distinct elements of S then (a+b) / gcd(a,b) is also in S. The funny thing is, the solution is: exactly the same, just characterizing the 3 smallest elements.
Amazing explanation
4:55 why does that imply f is injective and bijective?
ok i see why
Thank you! We want more. Keep going!
Why dony you use triagular inequality
Sir, which book will be great for learning that level of number ? please can you tell me
5:00 Unless you’re not allowed to use calculus, it seems like it would be easier to show the square root function is concave by showing its second derivative is always negative. If f(x) = x ¹/² then f’(x) = x ⁻¹/² /2 and f’’(x) = - x ⁻³/² / 4. Note that x ⁻³/² is always positive (it’s the reciprocal of the cube of a positive square root), so the second derivative is always negative (since it’s negative that number divided by 4).
Yes, that's very true. I just felt like doing it in a very elementary way :D
. On a natural number n you are allowed two operations: (1) multiply n by 2 or (2) subtract 3 from n. For example starting with 8 you can reach 13 as follows: 8 → 16 → 13. You need two steps and you cannot do in less than two steps. Starting from 11, what is the least number of steps required to reach 121? Is 10 correct or there can be least number of steps which are less than 10
10 is correct. The question is equivalent to solving the problem for the inverted process. Start with n = 121. One step consists of (A) replacing n with n / 2 or (B) replacing n with n + 3. What is the minimum number of steps required to reach 11? Steps BBA and AB are equivalent, so we never use BBA in a shortest sequence. Therefore, before the last occurence of A, we use B if and only if n is odd. As soon as n <= 11 and 3 | 11 - n, it is possible to finish without using A again and we will never use A again in an optimal sequence (since this is never better than only using B until reaching 11). Together, these observation determine the shortest sequence of steps uniquely (and this generalizes). So, the shortest sequence is 121 > 124 > 62 > 31 > 34 > 17 > 20 > 10 > 5 > 8 > 11 and has 10 steps.
@@calimath6701 thanks bro
this was ioqm problem 20 right
Wow what a solution ❤
Wild 💪🏼
But what’s the thinking process behind for coming up the inequality a_(n+2) >= a_n + 3?
The thought is that if you show that sequence is steadily increasing with a lower bound, you could use that to show that it’ll reach 3034 by the 2023rd term
@@filipeoliveira7001but why the +3?
@@raepiste8354 usually the person doesn’t start by making that insanely accurate guess, they start by trying to establish a lower bound by seeing if there can be, for example, repeated or decreasing terms in the sequence, eventually reaching a good lower bound such as that one, which leads to the solution
Your videos are just mind blowing 🤘
I solved it with mod 16. The hardest part is to see that you should take mod 16/mod 4 than it's easy.
Only Geo in this years imo
I want to get connected with you in any social media you want. I want to discuss maths. I want you and viewers of this channel to be my friends . Reply all please
Is your black pen also a Pilot V Board Master? Black line looks thicker that blue and red lines.
We switched our pens to ESENG Magic Markers, except for the orange one which is still a Pilot V Board Master.
@@calimath6701 Why have you switched to ESENG? Is it better than Pilot markers?
Yeah, we prefer ESENG, as our Pilot Markers weren't as consistent when low on ink.
I just press like button to appreciate your effort, but I never watch it, since I know I wouldn't get it even if I watch it hundred times
Thanks for your support anyways :). If you do watch a video and don't get something, feel free to ask us in the comments. We are happy to explain in more detail. Then, we are sure that you can understand this
@@calimath6701 My math is not that good, I solved several IOI questions, so I know one must be very good at math to solve IMO questions
Sehr schönes Video!
why did assume alpha is even in the first line?
The "alpha even" on the right is just to clarify that we have already found this solution to the problem. It is not an assumption, and in the rest of the proof, I also work with a general alpha.
The description says RMM Shortlist, not IMO
Whoops :D Thanks for the reminder
I don’t even understand the question…
So, in other words you're saying that triangles TNC and TNC' are equal, which further implies that TC = TC'. But how are these traingles equal? We only have a common side TN, NC = NC' and <NTC=<NTC'. Is that really enough to conclude the equation?
It is only enough if the angle is obtuse. This is given in our case, since N and C lie on different sides of SI_B and <NTS = 90.
Continue like that !!
There is another proof to this beautiful problem: Let O be the center and r be the radius of (AFE). So: BO² - r² = BF. BA = BD.BC CO² - r² = CE. CA = CD.CB We can substract them: BO² - CO² = BD² - CD² That means OD is perpendecular to BC. So: BO² = BD² + OD² We can use first equation: BD² + OD² - r² = BD.BC Hence: OD² - r² = BD.DC Also if we define T as the second intersection of AD and (AFE): OD² - r² = DT.DA Hence: DP.DA = DB.DC = DT.DA That means DP = DT. Also we know that the symmetry of T whit respect to OD is on the (AFE) again. Let's name that point Q'. By symmetry, DP = DT = DQ'. That means <TQ'P = 90°. So BC || TQ' hence OD perpendecular BC and OD perpendecular TQ'. And BC bisects TP. Hence BC is perpendecular bisector of Q'P. So Q' = Q and we are done.
Cool solution!
12:47 I don't think p(c) is unique. It seems easy to find a counterexample for n=3.
For n=6, m=7 is not >=11?
True. I intended to say that IF n satisfies the given condition, THEN m >= 11 as 9, 10 are consecutive divisors of n! Therefore, your example shows that n=6 doesn't satisfy the given condition.